Students can refer to the following Complex Numbers and Quadratic Equations MCQ Questions for Class 11 Maths with Answers provided below based on the latest curriculum and examination pattern issued by CBSE and NCERT. Our teachers have provided here a collection of multiple choice questions for Complex Numbers and Quadratic Equations Class 11 covering all topics in your textbook so that students can assess themselves on all important topics and thoroughly prepare for their exams
Complex Numbers and Quadratic Equations MCQ Questions for Class 11 Maths with Answers
We have provided below Complex Numbers and Quadratic Equations MCQ Questions for Class 11 Maths with answers which will help the students to go through the entire syllabus and practice multiple choice questions provided here with solutions. As Complex Numbers and Quadratic Equations MCQs in Class 11 Mathematics pdf download can be really scoring for students, you should go through all problems provided below so that you are able to get more marks in your exams.
Question. The val ues of ( ) / 16 1 4 are
(a) ±2, ± 2 i
(b) ±4 , ± 4 i
(c) ±1, ± i
(d) None of these
Answer
A
Question:
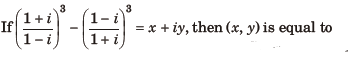
(a) (0, – 2)
(b) (-2, 0)
(c) (0, 2)
(d) (2, 0)
Answer
C
Question:

Answer
B
Question: The real value of a for which the expression

Answer
C
Question: The value of 1 + i2 + i4 + i6 +… + i2n is
(a) positive
(b) negative
(c) zero
(d) cannot be determined
Answer
D
Question:
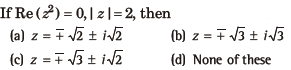
Answer
A
Question: The value of sum
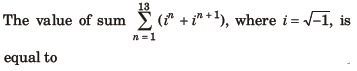
(a) i
(b) i – 1
(c) – i
(d) 0
Answer
B
Question: The real value of q for which the expression

Answer
B
Question: If(1+i)2/2-i=X+iY, then the value of x+y is equal to
(a) 5/2
(b) – 2/5
(c)2/5
(d) – 5/2
Answer
C
Question: Given z=q+ir/1+P,, then p+iq/1+r=1+iz/1-iz, if
(a) p2+ q2+ r2=1
(b) p2+q2+r2=2
(c) p2+ q2-r2=1
(d) None of these
Answer
A
Question: Two students while solving a quadratic equation in x, one copied the constant term incorrectly and got the roots 3 and 2. The other copied the constant term coefficient of x2 correctly as -6 and 1 respectively the correct roots are
(a) 3,- 2
(b) – 3, 2
(c) -6, 1
(d) 6, -1
Answer
D
Question: If the roots of the given equation
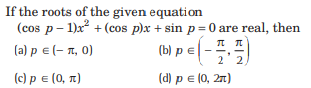
Answer
C
Question: The coefficient of x in the equation x2+px+q=0 was taken as 17 in place of 13 its roots were found to be – 2and -15. The roots of the original equation are
(a) 3, 10,
(b) – 3 -10 ,
(c) – 5- 8 ,
(d) None of these
Answer
B
Question: If P (x)= ax2+bx+c and Q(x) = – ax2+dx+c,where ac ≠0, then P(x) Q (x) = 0 has atleast
(a) four real roots
(b) two real roots
(c) four imaginary roots
(d) None of these
Answer
B
Question: If the roots of (a2+b2)x2-2(bc+ad)x+c2+d2=0 are equal, then
(a) a/b=c/d
(b) a/c+b/d=0
(c) a/d=b/c
(d) a+ b= c +d
Answer
A
Question: If α,β,y are the roots of x3+2x2-3x-=0,then α-2+β-2+y-2 is equal to
(a) 12
(b) 13
(c) 14
(d) 15
Answer
B
Question: If sin α sin β and cos α are in GP, then roots of x2+2x cot β+1=0 are always
(a) real
(b) real and negative
(c) greater than one
(d) non-real
Answer
A
Question: If sin and cos a a are the roots of the equation ax2+bx+c=0, then
(a) a2-b2+2ac=0
(b) (a-c)2=b2+c2)
(c) a2+b2-2ac=0
(d) a2+b2+2ac=0
Answer
A
Question: If one root of equation x2+ax+12=0 is 4 while the equation x2+ax+b=0 has equal roots, then the value of b is
(a) 4/49
(b) 49/4
(c) 7/4
(d) 4/7
Answer
B
Question: The solution set of the equation
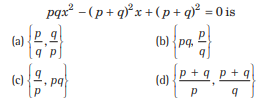
Answer
D
Question: Let f (x)= x2+ax+b;a,b ∈R.If f (1)+f(2)+f (3)=0, then the roots of the equation f (x) = 0
(a) are imaginary
(b) are real and equal
(c) are from the set {1, 2, 3}
(d) real and distinct
Answer
D
Question: If the product of the roots of the equation (a+1)x2+(2a+3)x+(3a+4)=0 is 2,, then the sum of roots is
(a) 1
(b) -1
(c) 2
(d) -2
Answer
B
Question: If the roots of the equation ax2+bx+c=0 of the form
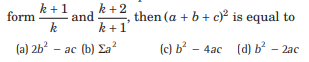
Answer
C
Question:The system y(x2+7x+12)=1 andx+y=6,y>0 has
(a) no solution
(b) one solution
(c) two solutions
(d) more than 2 solutions
Answer
D
Question:
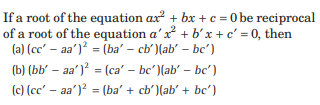
Answer
A
Question: If [x]2= [x+2], where [x]= the greatest integer less than or equal to x, then x must be such that
(a) x = 2, 1,
(b) [-1,0) ∪ [2 , 3)
(c) x ∈-[-1, 0)
(d) None of these
Answer
B
Question: If(1+i/1-i)x =1,then
(a) x = 2n + 1
(b) x = 4n
(c) x = 2n
(d) x = 4n + 1, where n ∈ N
Answer
B
Question: The conjugate of
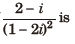
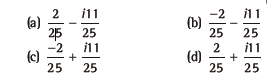
Answer
B
Question: If z-1/z+1is a purely imaginary number ( z≠ – 1), then the value of |z| is
(a) –1
(b) 1
(c) 2
(d) –2
Answer
B
Question: sin x+i cos 2x and cos x – i sin 2x are conjugate to each other for
(a) x= nπ
(b) x =(n+1/2) π/2
(c) x = 0
(d) No value of x
Answer
D
Question: If z = x + iy lies in the third quadrant, then
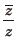
also lies in the third quadrant, if
(a) x > y > 0
(b) x < y < 0
(c) y < x < 0
(d) y > x > 0
Answer
B
Question:

(a) 0
(b) 1/2
(c) 1
(d) 2
Answer
C
Question: The value of ( z + 3)( ¯z + 3) is equivalent to
(a) | z + 3 |2
(b) | z – 3 |
(c) z2 + 3
(d) None of these
Answer
A
Question: If |z + 1|= z + 2 (1 + i), then the value of z is
(a)1/2- 2i
(b)1/2+ 2i
(c)1/2- 3i
(d)1/3- 2i
Answer
A
Question:
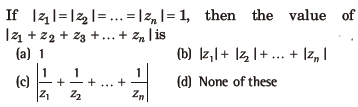
Answer
D
Question:|z1+z2 |=|z1 |+|z2 | is possible, if
(a) z2 ¯z1 =
(b) z2 =1/z1
(c) arg (z1) = arg (z2)
(d) | z1 | | z2 |
Answer
C
Question: If| z – 2|= min {|z – 1|,|z – 5|} , where z is a complex number, then
(a) Re (z) = 3/2
(b) Re (z) = 7/2
(c) Re (z ) ∈{3/2,7/2}
(d) None of these
Answer
C
Question: If z is a comp lex num ber, then ( z-1)( z) is equal to
(a) 1
(b) -1
(c) 0
(d) None of these
Answer
A
Question: If the points z1,z2 and z3 and are the verti ces of anequilateral triangle in the complex plane, then the value of
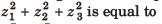
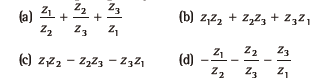
Answer
B
Question: In the argand plane the complex number z = 4 – 3 i is
turned in the clockwise sense through 180° and stretched three times. The com plex num ber rep re sented by the new num ber is
(a) 12 + 9i
(b) 12 – 9i
(c) -12 – 9i
(d) -12 + 9i
Answer
D
Question: In a given parallelogram, if the points P1 and P2 represent two complex numbers z1 and z2,then the point P3 represents the num ber
(a) z1+ z2
(b) z1– z2
(c) z1x z2
(d) z1÷ z2
Answer
A
Question: The points rep re sented by the com plex num bers 1 + i, -2 +3 i and 5/3 i on the ar gand di a gram are
(a) vertices of an equilateral triangle
(b) vertices of an isosceles triangle
(c) collinear
(d) None of the above
Answer
C
Question: Let z1 and z2 be the non-real roots of the equa tion 3z2+ 3z+b=0. If the ori gin to gether with the points rep re sented by z1 and z2 form an equi lat eral tri an gle, then the value of b is
(a) 1
(b) 2
(c) 3
(d) None of these
Answer
A
Question:
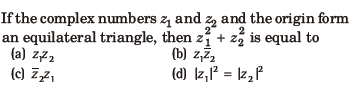
Answer
A
Question: If the comp lex num bers iz, z and z + iz rep re sent the three ver ti ces of a triangle, then the area of the triangle is
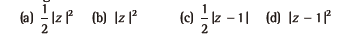
Answer
B
Question:
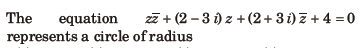
(a) 2
(b) 3
(c) 4
(d) 6
Answer
B
Question: The area of the triangle whose Vertices are represented by the complex numbers
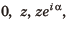
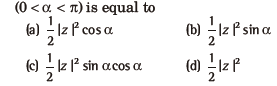
Answer
D
Question: If the real part of
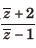
is 4, then the locus of the point representing z in the complex plane is
(a) a circle
(b) a parabola
(c) a hyperbola
(d) an ellipse
Answer
A
Question:
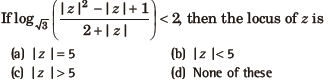
Answer
B
Question: When z+ i/z+2 is purely imaginary, the locus described by the point z in the argand diagram is a
(a) circle of radius√5/2
(b) circle of radius 5/4
(c) straight line
(d) parabola
Answer
A
Question:
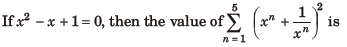
(a) 8
(b) 10
(c) 12
(d) None of these
Answer
A
Question: The equation of a circle whose radius and centre are r and z0 respectively, is
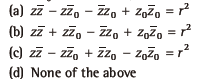
Answer
A
Question: If iz3+ z2– z +i =0,then |z| is equal to
(a) 1
(b) i
(c) -1
(d) – i
Answer
A
Question: The centre of the circle
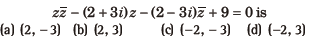
Answer
A
Question:
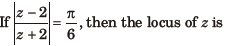
(a) a straight line
(b) a circle
(c) a parabola
(d) an ellipse
Answer
B
Question: The maximum distance from the origin of coordinates to the point z satisfying the equation
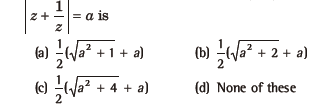
Answer
C
Question: The value of x4+9x3+35x2-x+4 for
x = – 5 + 2√-4 is
(a) 0
(b) –160
(c) 160
(d) –164
Answer
B
Question: Let z1,z2 and z3 be the affixes of the vertices of a triangle having the circumcenter at the origin. If z is the affix of it’s orthocenter then z is equal to
(a)z1+ z2+ z3
(b)z1+ z2+ z3/2
(c) z1+ z2+ z3
(d) None of these
Answer
C
Question:
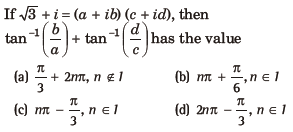
Answer
B
Question:
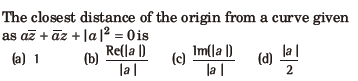
Answer
D
Question: The num ber of non-zero integral solutions of the equation |1 – i|x = 2x is
(a) infinite
(b) 1
(c) 2
(d) None of these
Answer
B
Question:
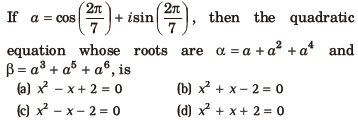
Answer
D
Question: Given that the equation z2+(p+iq) z+ r+ is =0 where p, q, rand s are real and non-zero roots, then
(a) pqr = r2 + p2s
(b) prs = q2 + r2p
(c) qrs = p2 + s2q
(d) pqs = s2 + q2r
Answer
D
Question:
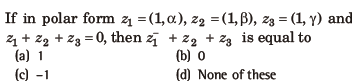
Answer
B
Question. If x = a + b, y = aa + bb and z = ab + ba, where a and b are complex cube roots of unity, then xyz is equal to
(a) a2 + b2
(b) a3 + b3
(c) a3b3
(d) a3 – b3
Answer
B
Question. If w( ¹ 1) is a cube root of unity and (1 ) , + w 7 = A + Bw then A and B are re spec tively
(a) 0 , 1
(b) 1, 1
(c) 1, 0
(d) -1, 1
Answer
B
Question. If w is a com plex cube root of unity, then for pos i tive in te gral value of n, the prod uct of w× w2 × w3 w ….. n will be
(a) 1-i√3/2
(b) 1-I√3/2
(c) 1
(d) Both (b) and (c)
Answer
B
Question. Com mon roots of the equa tions z3+z2 + 2z + 1 = 0 and z1985 + z100 = 0 are
(a) ω, ω2
(b) ω, ω3
(c) ω2 ω3 ,
(d) None of these
Answer
B
Question. If a1 , a2 , …, an -1 are n roots of unity, then the value of (1- a1 ) (1- a2) (1- a3) . . . (1- an-1) is equal to
(a) 3
(b) 1/2
(c) n
(d) 0
Answer
C
Question. If Im (z-1/2z+1) = -4, then lo cus of z is
(a) an ellipse
(b) a parabola
(c) a straight line
(d) a circle
Answer
D
Question. In the ar gand plane the com plex num ber z = 4 – 3 i is turned in the clock wise sense through 180° and stretched three times. The com plex num ber rep re sented by the new num ber is
(a) 12 + 9i
(b) 12 – 9i
(c) -12 – 9i
(d) -12 + 9i
Answer
D
Question. If the com plex num bers z z 1 2 and and the origin from an equilateral triangle, then z12 + z22 is equal to
(a) z1z2
(b) z1z2
(c) z2z1
(d) |z1|2 = |z2|2
Answer
A
Question. In a given parallelogram, if the points P1 and P2 represent two complex numbers z1 and z2,then the point P3 represents the number
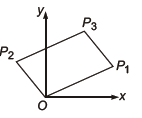
(a) z1 + z2
(b) z1 – z2
(c) z1 x z2
(d) z1 + z2
Answer
A
Question. Let z1 and z2 be the non-real roots of the equa tion 3z2 + 3z + b = . If the ori gin to gether with thepoints rep re sented by z1 and z2 and form an equi lat eral tri an gle, then the value of b is
(a) 1
(b) 2
(c) 3
(d) None of these
Answer
A
Question. Locus of z, if arg (z-1/z+1) = π/2 , is
(a) a circle
(b) a semi-circle
(c) a straight line
(d) None of these
Answer
B
Question. The lo cus of the points z which sat isfy the con di tion arg (z-1/z+1) = π/3 , is
(a) a straight line
(b) a circle
(c) a parabola
(d) None of these
Answer
B
Question. Let z be a complex number and a be a real parameter such that z2 + ax + a2 = 0, then
(a) locus of z is a pair of straight lines
(b) locus of z is a circle
(c) arg (z) = ± 5π/3
(d) |z | = – 2|a |
Answer
A
Question. If z1 , z2 , z3 and z4 are the af fixes of four points in the ar gand plane and z is the af fix of a point, such that
|z – z1|=|z – z2|=|z – z3|=|z – z4|, then and are
(a) concyclic
(b) vertices of a parallelogram
(c) vertices of a rhombus
(d) in a straight line
Answer
A
Question. Let z and ω be com plex num bers such that z + iw = 0 and arg ( zω) = p. Then, arg (z̄) is equal to
(a) π /4
(b) π /2
(c) 3π /4
(d) 5π /2
Answer
C
Question. If |z2 – | |z|2 + 1 = + 1 then z lies on
(a) the real axis
(b) the imaginary axis
(c) a circle
(d) an ellipse
Answer
B
Question. The points rep re sented by the com plex num bers 1 + i, -2 + 3i and 5/3 on the ar gand di a gram are
(a) vertices of an equilateral triangle
(b) vertices of an isosceles triangle
(c) collinear
(d) None of the above
Answer
C
Question. If the area of the triangle on the complex plane formed by the points z, z + iz and iz is 200, then the value of |3 z| must be equal to
(a) 20
(b) 40
(c) 60
(d) 80
Answer
C
Question. Re( z ) 2 = 1 is rep re sented by
(a) the circle x2 + y2 = 1
(b) the hyperbola x2 – y2 = 1
(c) parabola or a circle
(d) All of these
Answer
B
Question. If the comp lex num bers iz, z and z + iz rep re sent the three ver ti ces of a tri an gle, then the area of the tri an gle is
(a) 1/2|z|2
(b) |z|2
(c) 1/2|z – 1|
(d) |z – 1|2
Answer
A
Question. The area of the tri an gle whose ver ti ces are rep re sented by the com plex num bers 0, z zei , a,(0 < a < p) is equal to
(a) 1/2|z|2 cosα
(b) 1/2|z|2 sinα
(c) 1/2|z|2 sinαcosα
(d) 1/2|z|2
Answer
D
Question.|z-2/z+2| =π/6 , then the lo cus of z is
(a) a straight line
(b) a circle
(c) a parabola
(d) an ellipse
Answer
B
Question. If z1 and z2 are two non-zero com plex num bers such that |z1 + z2| = |z2| |z1| + |z2| then arg ( z2 ) arg is equal to
(a) -π/2
(b) 0
(c) -π
(d) π/2
Answer
B
Question. If z and w are two non-zero com plex num bers such that |zw|= 1 and arg ( z) – arg (w) = , π/2 then z̄w is equal to
(a) 1
(b) -1
(c) i
(d) – i
Answer
D
Question. If the real part of z̄+2/z̄-1 is 4, then the lo cus of the point representing z in the com plex plane is
(a) a circle
(b) a parabola
(c) a hyperbola
(d) an ellipse
Answer
A
Question. The equa tion of a cir cle whose ra dius and centre are r and z0 respectively, is
(a) zz̄ – zz̄0 – z̄z0 + z0z̄0 = r2
(b) zz̄ + zz̄0 – z̄z0 + z0z̄0 = r2
(c) zz̄ – zz̄0 + z̄z0 – z0z̄0 = r2
(d) None of the above
Answer
A
Question. If z3 + z2 – z + i = 0, then |z| is equal to
(a) 1
(b) i
(c) -1
(d) – i
Answer
A
Question. The centre of the circle
zz̄ – (2 + 3i) z – (2 – 3i)z̄ + 9 = 0 is
(a) (2, – 3)
(b) (2, 3)
(c) (-2, – 3)
(d) (-2, 3)
Answer
A
Question. The num ber of non-zero in te gral so lu tions of the equa tion |1 – i|x = 2x is
(a) infinite
(b) 1
(c) 2
(d) None of these
Answer
B

We hope you liked Complex Numbers and Quadratic Equations MCQ Questions for Class 11 Maths provided above. If you have any questions, please post them in the comments box below. Our teachers will provide a response.