Please refer to Constructions Class 9 Mathematics notes and questions with solutions below. These revision notes and important examination questions have been prepared based on the latest Mathematics books for Class 9. You can go through the questions and solutions below which will help you to get better marks in your examinations.
Class 9 Mathematics Constructions Notes and Questions
Construction of Perpendicular Bisector of a Line Segment and Its Verification The Perpendicular Bisector
Have you ever seen a carpenter cutting a plank of wood? How does he cut the plank into two equal parts? The carpenter does so by placing the hacksaw in the middle of the plank such that the latter is aligned perpendicularly to the former.
Thus, the carpenter makes use of the concept of the perpendicular bisector while cutting the plank of wood.
In this lesson, we will learn about:
1) The perpendicular bisector of a line segment
2) The construction of the bisector and its verification
Understanding Perpendicular Bisector of a Line Segment
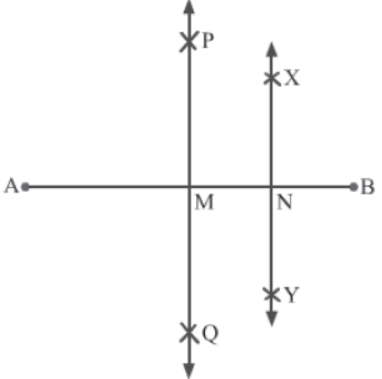
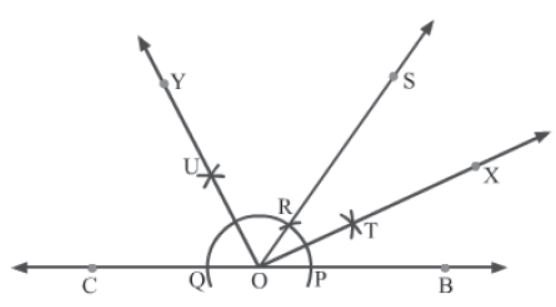
Observe the given figure.
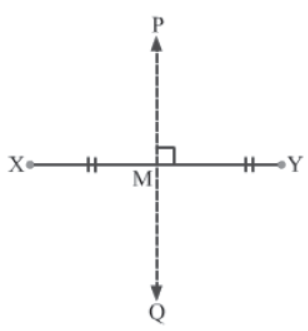
We can see that: XM = MY and ∠PMY = 90°.
This means that the line PQ not only bisects the line segment XY but is also perpendicular to it. So, PQ is the perpendicular bisector of the line segment XY.
We can, thus, define the perpendicular bisector of a line segment as follows:
A line that bisects a line segment at 90° is called the perpendicular bisector of the line segment.
Solved Examples
Easy
Example 1: Construct the perpendicular bisector of a seven-centimetre-long line segment AB. Measure the length of each bisected part.
Solution:
i) Draw line segment AB = 7 cm using a ruler.
ii) Draw two intersecting arcs on each side of AB, taking A and B as the centres and the radius as more than 3.5 cm. Let the arcs intersect at points P and Q. Join P and Q. Let PQ intersect AB at point M.
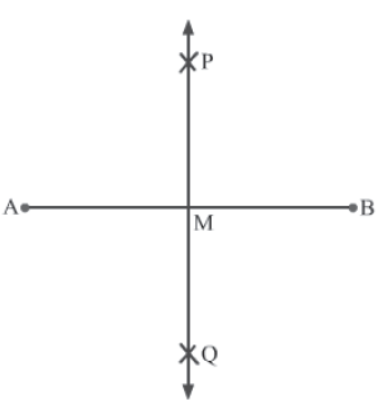
PQ is the perpendicular bisector of line segment AB. It bisects AB at M into the two equal parts AM and MB.
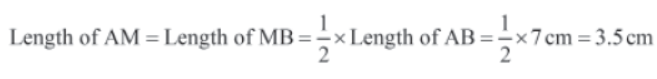
Medium
Example 1: Construct the perpendicular bisector of an eight-centimetre-long line segment. Further, bisect one of the bisected parts of the line segment and measure its length.
Solution:
i) Draw a line segment AB = 8 cm using a ruler.
ii) Draw two intersecting arcs on each side of AB, taking A and B as the centres and the radius as more than 4 cm. Let the arcs intersect at points P and Q. Join P and Q. Let PQ intersect AB at point M.
PQ is the perpendicular bisector of line segment AB. It bisects AB at M into the two equal parts AM and MB.
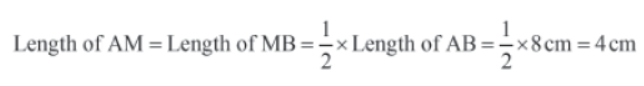
iii) Now, draw two intersecting arcs on each side of MB, taking M and B as the centres and the radius as more than 2 cm. Let the arcs intersect at points X and Y. Join X and Y. Let XY intersect MB at N.
XY is the perpendicular bisector of line segment MB. It bisects MB at N into the two equal parts MN and NB.
Hard
Example 1: Construct a circle centred at O and having two non-parallel chords AB and CD. Construct the perpendicular bisectors of AB and CD. At what point do the bisectors intersect?
Solution:
i) Draw a circle of any radius with the centre as O.
ii) Draw two chords AB and CD inside the circle such that AB is not parallel to CD.
iii) Draw two intersecting arcs on each side of AB, taking A and B as the centres and the radius as more than half of AB. Let the arcs intersect at points X and Y. Join X and Y. Let XY intersect AB at point E.
XY is the perpendicular bisector of chord AB. It bisects AB at E into the two equal parts AE and EB.
iv) Now, draw two intersecting arcs on each side of CD, taking C and D as the centres and the radius as more than half of CD. Let the arcs intersect at points P and Q. Join P and Q. Let PQ intersect CD at point F.
PQ is the perpendicular bisector of chord CD. It bisects CD at F into the two equal parts CF and FD.
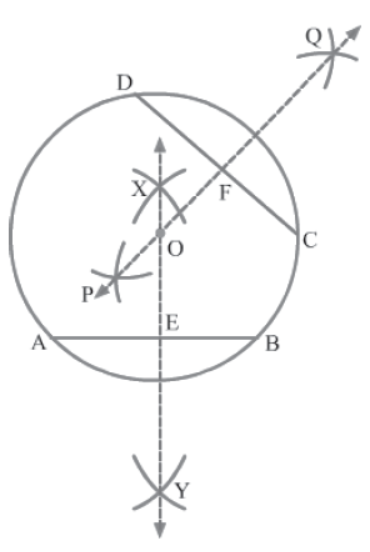
It is clear from the figure that the perpendicular bisectors of chords AB and CD intersect at point O, i.e., the centre of the circle.
Construction of Bisector of an Angle and Its Verification
The Bisector of an Angle
You must have seen a catapult and perhaps even used it to propel pebbles. What happens when you release the pulled sling of the catapult? When the pulled sling is released, the pebble placed on it is made to move forward. The path followed by the pebble bisects the angle formed by the pulled sling. In other words, the line traced by the forward-moving pebble divides the angle formed by the pulled sling into two equal angles.
Thus, the propelling of pebbles with a catapult involves the concept of the angle bisector.
In this lesson, we will learn about:
1) The bisector of an angle
2) The construction of the bisector and its verification
Understanding the Angle Bisector
Observe the given figure.
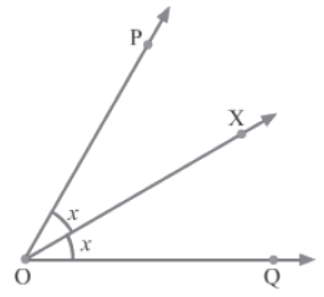
We can see that OX divides ∠POQ into ∠POX and ∠XOQ, which are equal.
This means that OX is the bisector of ∠POQ.
If ∠POQ = 2x
Then ∠POX = ∠XOQ = 2x/x = x
We can, thus, define the bisector of an angle as follows:
A ray that divides an angle into two equal parts is called the bisector of the angle.
Solved Examples
Easy
Example 1: Construct an angle measuring 52.5° by bisecting an angle measuring 105°.
Solution:
i) Draw ∠POQ = 105° using a protractor.
ii) Draw an arc of any radius taking O as the centre. Let this arc intersect the arms OP and OQ at points X and Y respectively.
iii) Now, draw intersecting arcs taking X and Y as the centres and the radius as more than half of XY. Let the arcs intersect at point R. Join O and R. Extend OR to form a ray.
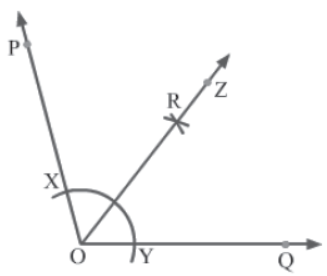
OR is the bisector of ∠POQ. It divides the angle into the two equal parts ∠POR and ∠ROQ.
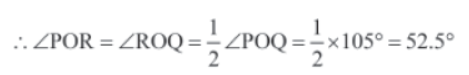
Medium
Example 1: Draw an angle measuring 90° using a protractor. With the help of this angle, construct an angle measuring 67.5°.
Solution:
i) Draw an ∠AOB = 90° using a protractor.
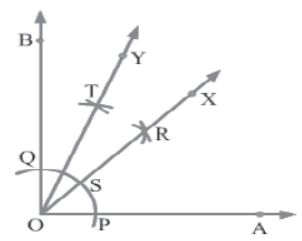
ii) Draw an arc of any radius taking O as the centre. Let this arc intersect the arms OA and OB at points P and Q respectively.
iii) Now, draw intersecting arcs taking P and Q as the centres and the radius as more than half of PQ. Let the arcs intersect at point R. Join O and R. Extend OR to form a ray OX.
OX is the bisector of ∠AOB. It divides the angle into the two equal parts ∠AOX and ∠XOB.
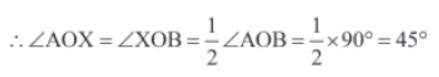
iv) Mark the point of intersection of ray OX and arc PQ as S.
v) Then, draw intersecting arcs taking Q and S as the centres and the radius as more than half of QS. Let the arcs intersect at point T. Join O and T. Extend OT to form a ray OY.
OY is the bisector of ∠XOB. It divides the angle into the two equal parts ∠XOY and ∠YOB.
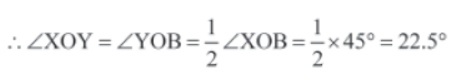
Now,
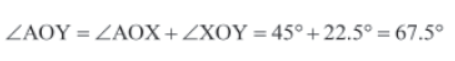
Thus, ∠AOY is the required angle of measure 67.5°.
Hard
Example 1: Draw a linear pair of angles. By bisecting each angle, show that the angle bisectors are perpendicular to each other.
Solution:
i) Draw a line COB using a ruler.
ii) Taking O as the centre and a suitable radius, draw an arc intersecting COB at points P and Q.
iii) Then, taking P as the centre and keeping the same radius as before, draw an arc intersecting the previous arc, say at point R. Join O and R. Extend OR to form a ray OS.
Hence, we will get ∠SOB and ∠SOC as a linear pair of angles.
iv) Next, draw intersecting arcs taking P and R as the centres and the radius as more than half of PR. Let the arcs intersect at point T. Join O and T. Extend OT to form a ray OX.
OX is the bisector of ∠SOB. It divides the angle into the two equal parts ∠SOX and ∠XOB.
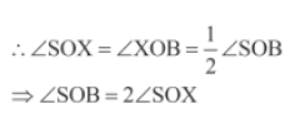
v) Now, draw intersecting arcs taking Q and R as the centres and the radius as more than half of QR. Let the arcs intersect at point U. Join O and U. Extend OU to form a ray OY.
OY is the bisector of ∠SOC. It divides the angle into the two equal parts ∠SOY and ∠YOC.
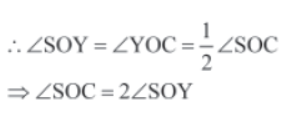
We know that ∠SOB and ∠SOC form a linear pair.
So,
∠SOB + ∠SOC = 180°
⇒ 2∠SOX + 2∠SOY = 180°
⇒ 2(∠SOX + ∠SOY) = 180°
⇒ ∴ ∠SOX + ∠SOY = 180°/2 = 90°
Thus, angle bisectors OX and OY are perpendicular to each other.
Construction of Angles of Special Measures and Its Verification
Constructing Angles of Special Measures
You can easily draw different angles using a protractor. Another way of constructing angles involves the use of a ruler and a compass. Angles that are multiples of 15° (e.g., 30°, 45°, 60°, 90°, 120°, 135°, etc.) can be constructed in this way. For example, we can construct an angle measuring 45° by bisecting an angle measuring 90°. Similarly, we can construct an angle measuring 120° with the help of an angle measuring 60°. In this lesson, we will learn how to construct such angles with the help of a ruler and a compass.
Constructing Angle of 30°
i) Construct an angle measuring 60° using a ruler and a compass, as is shown in the figure.
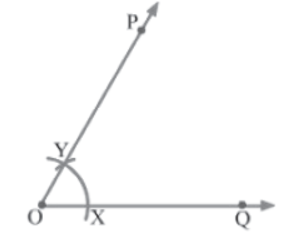
ii) Construct the bisector of ∠POQ in the following manner. Draw two intersecting arcs taking X and Y as the centres and the radius as more than half of XY. Let the arcs intersect at point R. Join O and R. Extend OR to form a ray OZ. OZ is the bisector of ∠POQ.
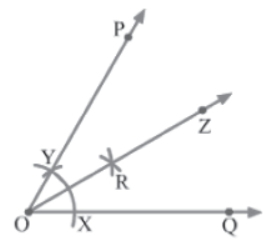
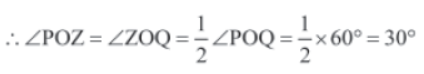
Constructing Angle of 45°
i) Construct an angle measuring 90° using a ruler and a compass, as is shown in the figure.
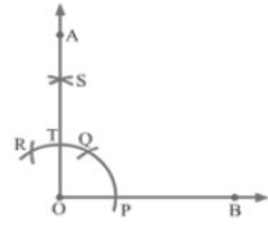
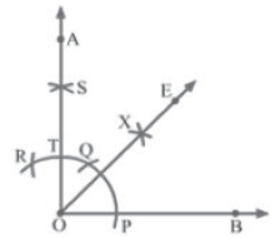
ii) Construct the bisector of ∠AOB in the following manner. Draw two intersecting arcs taking T and P as the centres and the radius as more than half of TP. Let the arcs intersect at point X. Join O and X. Extend OX to form a ray OE. OE is the bisector of ∠AOB.
Constructing Angle of 180°
i) Draw a line AB.

ii) Take a point O on AB, in between points A and B.
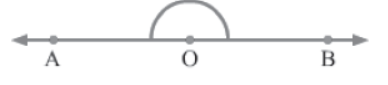
∠AOB is the required angle of measure 180°.
Solved Examples
Medium
Example 1: Construct an angle measuring 135°.
Solution:
We know that 135° = 45° + 90°. So, we will first construct an angle measuring 90° and then an angle measuring 45°.
i) Draw a line AB and take a point O on it.
ii) Taking O as the centre and with a suitable radius, draw an arc such that it cuts AB at points C and F.
iii) Next, taking C as the centre and keeping the same radius as before, draw an arc intersecting the previous arc, say at point D. Now, with D as the centre and the same radius, draw an arc intersecting the first drawn arc, say at point E.
iv) Then, draw intersecting arcs taking D and E as the centres and the radius as more than of DE. Let the arcs intersect at point G. Join O and G. Extend OG to form a ray OH. Let OH intersect the first drawn arc at point I.
We will have ∠HOA = ∠HOB = 90°.
v) Construct the bisector of ∠HOA in the following manner. Draw intersecting arcs taking I and F as the centres and the radius as more than half of IF. Let the arcs intersect at point J.
Join O and J. Extend OJ to form a ray OK. OK is the bisector of ∠HOA.

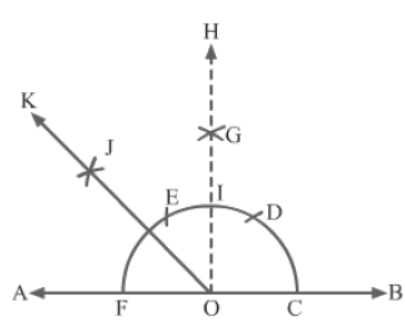
Now, ∠KOB = ∠HOK + ∠HOB = 90° + 45° = 135°
Thus, ∠KOB is the required angle of measure 135°.
Hard
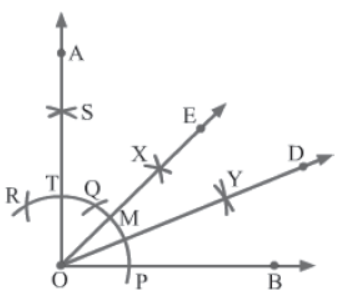
Example 1: Construct an angle measuring 22.5°.
Solution: We know that 22.5° = 1/2 x 45° and 45° = 1/2 x 90° . So, we will first construct an angle measuring 90°; then, we will bisect it to get an angle measuring 45°; and, finally, we will bisect the 45° angle to get an angle measuring 22.5°.
i) Draw a ray OB with the initial point O.
ii) Draw an arc taking O as the centre and with any radius, such that it cuts OB at point P.
iii) Taking P as the centre and with the same radius as before, draw an arc intersecting the previous arc, say at point Q. Now, with Q as the centre and the same radius, draw an arc intersecting the first drawn arc, say at point R.
iv) Then, draw intersecting arcs taking Q and R as the centres and the radius as more than half of QR. Let the arcs intersect at point S. Join O and S. Extend OS to form a ray OA. Let OA intersect the first drawn arc at point T.
We will have ∠AOB = 90°.
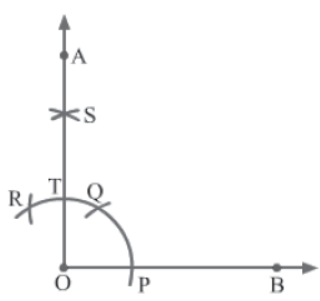
i) Construct the bisector of ∠AOB in the following manner. Draw intersecting arcs taking T and P as the centres and the radius as more than half of TP. Let the arcs intersect at point X.
Join O and X. Extend OX to form a ray OE. Let OE intersect the first drawn arc at point M. OE is the bisector of ∠AOB.
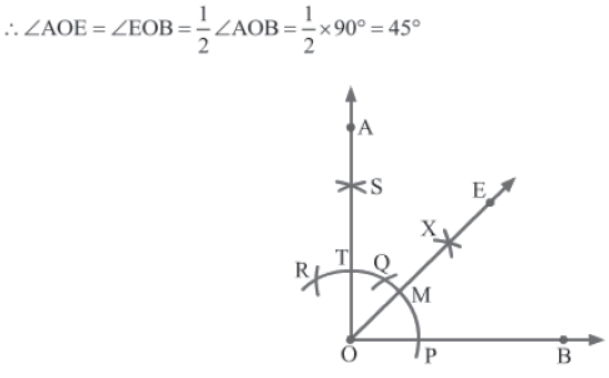
ii) Construct the bisector of ∠EOB in the following manner. Draw intersecting arcs taking M and P as the centres and the radius as more than half of MP. Let the arcs intersect at point Y. Join O and Y. Extend OY to form a ray OD. OD is the bisector of ∠EOB.
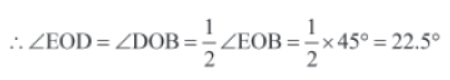
Construction of a Triangle Whose Base, Sum or Difference of Other Two Sides and Base Angle are Given and Reasoning behind It
Constructing a Triangle
You have learnt how to construct some common angles, the bisector of an angle and the perpendicular bisector of a line segment. These are some of the basic constructions used while constructing polygons. In this lesson, we will learn about constructing triangles.
A triangle is a three-sided polygon, the sum of whose interior angles is 180°. It can be easily constructed when:
• The lengths of all its sides are given.
• The lengths of any two sides and the measure of the angle between them are given.
• The measures of two angles and the length of the side between them are given.
• The lengths of the hypotenuse and either of the perpendicular sides of a right-angled triangle are given.
There are some additional ways of constructing a triangle. In this lesson, we will learn how to construct a triangle using the following information.
• The length of its base
• The measure of one of the base angles
• The sum of or the difference between the lengths of the other two sides
Solved Examples
Medium
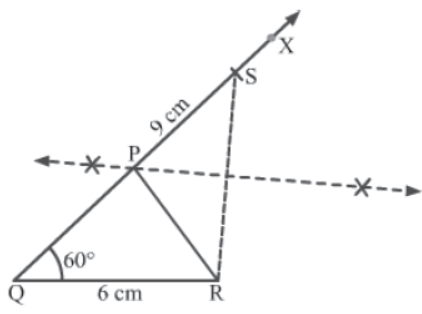
Example 1: Construct a triangle PQR in which QR = 6 cm, ∠Q = 60° and PQ + PR = 9 cm.
Solution:
i) Draw a line segment QR = 6 cm.
ii) At vertex Q, draw an angle measuring 60° and name it ∠RQX.
iii) Taking Q as the centre and the radius as 9 cm (i.e., the given sum of PQ and PR), draw an arc intersecting QX, say at point S. Join R and S.
iv) Draw the perpendicular bisector of RS. Let it intersect QS at P. Join P and R to get the required triangle PQR.
Solved Examples
Medium
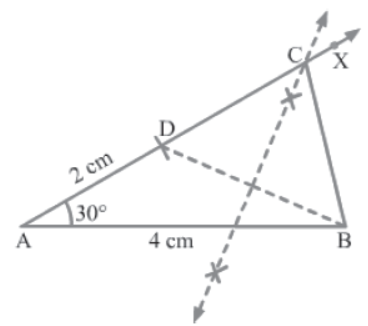
Example 1: Construct a triangle ABC in which AB = 4 cm, ∠A = 30° and AC − BC = 2 cm.
Solution:
i) Draw a line segment AB = 4 cm.
ii) At vertex A, draw an angle measuring 30° and name it ∠BAX.
iii) Taking A as the centre and the radius as 2 cm (i.e., the given difference between AB and BC), draw an arc intersecting AX, say at point D. Join B and D.
iv) Draw the perpendicular bisector of BD. Let it intersect AX at C. Join B and C to get the required triangle ABC.
Construction of a Triangle When Its Perimeter and Base Angles are Given and Reasoning behind It
Constructing a Triangle Using Its Perimeter
There are different conditions in which a triangle can be constructed; for example, when the lengths of all its sides are given, or when the measure of two angles and the length of the side between them are given. In this lesson, we will study how to construct a triangle when its perimeter is given.
Is perimeter sufficient to construct a unique triangle? Let us see. If we know the perimeter of a triangle, then we can construct many triangles with different dimensions. For example, if the perimeter of a triangle is given as 13 cm, then we can construct triangles with sides 3 cm, 4 cm and 6 cm, or 4 cm, 4 cm and 5 cm, or 3 cm, 5 cm and 5 cm, etc.
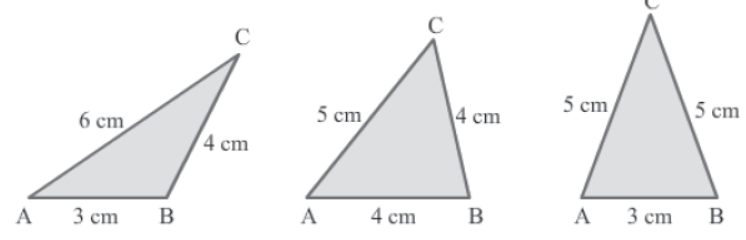
So, to construct a unique triangle, we need to know some components of the triangle in addition to its perimeter. In this lesson, we will learn how to construct a triangle when its perimeter and the base angles are given.
Verification of the Constructed Triangle
We can verify the constructed triangle by showing that ∠B = 30°, ∠C = 90° and AB + BC + CA = 11 cm.
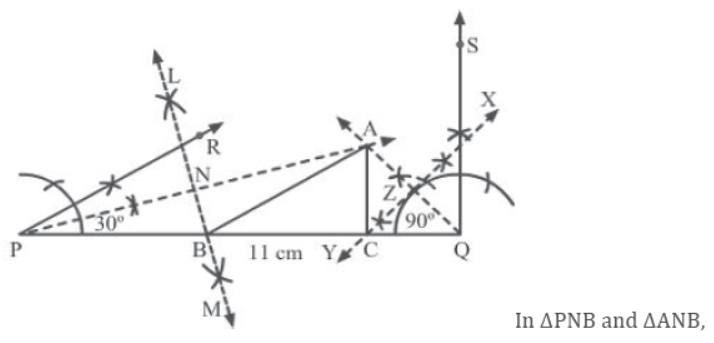
PN = AN ( LM is the perpendicular bisector of AP)
∠PNB = ∠ANB = 90° ( LM is the perpendicular bisector of AP)
NB = NB (Common)
∴ ΔPNB ≅ ΔANB (By SAS congruency criterion)
⇒ AB = BP and ∠NPB = ∠NAB, i.e., ∠APB = ∠PAB (By CPCT)
Similarly, we can prove AC = CQ and ∠CAQ = ∠CQA.
∴ AB + BC + CA = BP + BC + CQ = PQ = 11 cm
∠ABC = ∠APB + ∠PAB (Using the exterior angle property)
= 2∠APB ( ∠APB = ∠BAP)
= ∠RPQ
∴ ∠ABC = ∠RPQ = 30°
Similarly, we can prove ∠ACB = ∠SQP = 90°.
Thus, the construction is valid.
Solved Examples
Easy
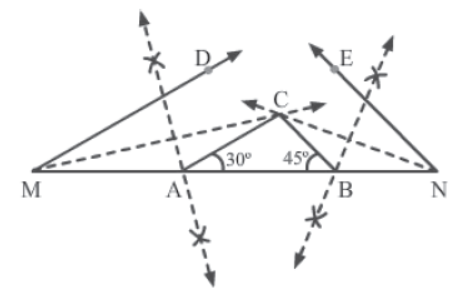
Example 1: Construct a triangle ABC with perimeter as 12 cm, ∠A = 30°and ∠B = 45°.
Solution:
i) Draw a line segment MN = 12 cm (i.e., same as the given perimeter). Construct angles measuring 30° and 45° at M and N respectively.
ii) Construct the angle bisectors of ∠DMN and ∠ENM. Let the angle bisectors meet at point C.
iii) Construct the perpendicular bisectors of CM and CN. Let the perpendicular bisectors intersect MN at points A and B respectively.
iv) Connect point C to points A and B to obtain the required triangle ABC.
Hard
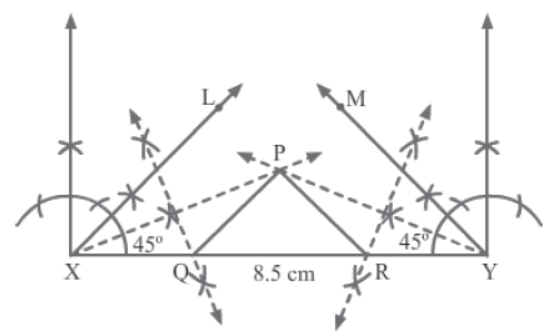
Example 1: Construct an isosceles right triangle PQR whose perimeter is 8.5 cm.
Solution:
The angles of an isosceles right triangle are 90°, 45° and 45°.
In ΔPQR, let us consider that ∠P = 90°, ∠Q = 45° and ∠R = 45°.
Also, perimeter of ΔPQR = PQ + QR + RP = 8.5 cm
Let us construct this triangle.
i) Draw a line segment XY = 8.5 cm (i.e., same as the given perimeter). Construct angles measuring 45° each at X and Y.
ii) Construct the angle bisectors of ∠LXY and ∠MYX. Let the angle bisectors meet at point P.
iii) Construct the perpendicular bisectors of PX and PY. Let the perpendicular bisectors intersect XY at points Q and R respectively.
iv) Connect point P to points Q and R to obtain the required isosceles right triangle PQR.