Please refer to Coordinate Geometry Class 9 Mathematics notes and questions with solutions below. These revision notes and important examination questions have been prepared based on the latest Mathematics books for Class 9. You can go through the questions and solutions below which will help you to get better marks in your examinations.
Class 9 Mathematics Coordinate Geometry Notes and Questions
Cartesian Plane and Reading Location of Points Plotted on It
Need for Coordinate Geometry
Consider a situation wherein we have the students of a class sitting in different rows and we need to locate the position of one particular student. Let us suppose that this student is sitting in the second row. Using this information, can we ascertain the student’s exact location?
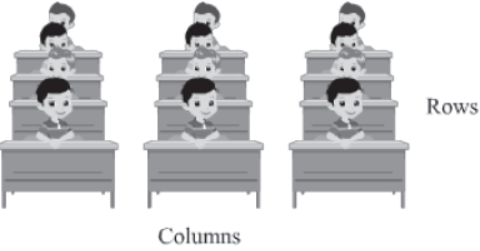
No, we cannot. The given information is insufficient. For us to be able to locate this student in the class, we also need to know the column in which he/she is sitting. So, we require two variables to define the student’s position—one for the row and the other for the column.
Such situations—wherein we require two attributes to locate points, objects, etc.—can be dealt with by using coordinate geometry. It is the geometric system in which the positions
of points on a plane are described using ordered pairs of numbers.
Topics to be covered in this lesson:
•Terminology related to coordinate geometry
•Convention of signs in the different quadrants of the Cartesian plane
•Reading the positions of points plotted on the coordinate plane
Did You Know?
The Cartesian plane is named after the famous mathematician and philosopher René Descartes to honour his contributions to the field of coordinate geometry.
Know Your Scientist

An ordered pair is a pair of two objects taken in particular order. In co-ordinate geometry, an ordered pair means, a pair of two numbers in which order is important.
To form an ordered pair, the numbers are written in specific order, separated by a comma, and enclosed in small brackets.
For example:
(3, 5), (−2, 8), (14, 67)
All of the above represents an ordered pair.
Coordinates of a Point
A point on the Cartesian (or coordinate) plane is defined by an ordered pair. The first value in this pair – also known as the x-coordinate or the abscissa – represents the perpendicular distance of the point from the y-axis. The second value – also known as the ycoordinate or the ordinate – indicates the erpendicular distance of the point from the xaxis.
These coordinates are written in the form (x-coordinate, y-coordinate), i.e., (x, y).
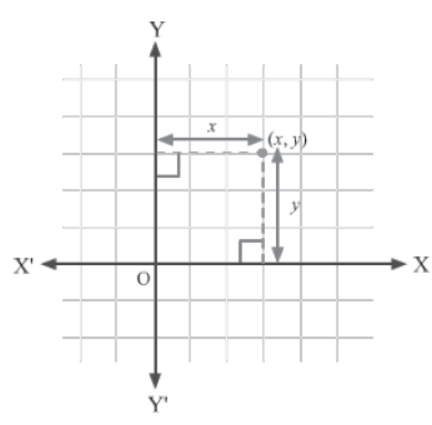
The line X’OX is called the x-axis and Y’OY is called the y-axis. The point O is called the origin of the coordinate system. The equation of the x-axis is y = 0 whereas the equation of the y-axis is x = 0.
Sign conventions of coordinates
Suppose the coordinates of a point are (x, y). Then, depending upon the quadrant of the plane in which the point lies, the signs of (x, y) will be as follows:
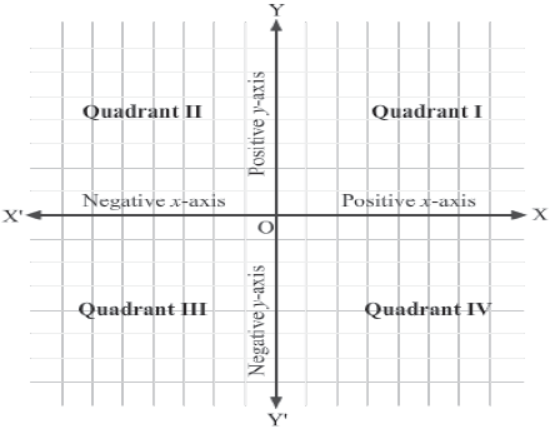
(1)If the point lies in quadrant I, then x > 0 and y > 0. So, both x and y will be positive.
(2)If the point lies in quadrant II, then x < 0 and y > 0. So, x will be negative and y will be positive.
(3)If the point lies in quadrant III, then x < 0 and y < 0. So, both x and y will be negative.
(4)If the point lies in quadrant IV, then x > 0 and y < 0. So, x will be positive and y will be negative.
Note: If a point lies on the x-axis, then y = 0; if it lies on the y-axis, then x = 0.
Sign conventions of coordinates
Solved Examples
Easy
Example 1: A point lies on the x-axis. Find the ordinate of this point.
Solution:
We know that the ordinate of a point is the perpendicular distance of the point from the xaxis.
Since the given point lies on the x-axis, its perpendicular distance from the x-axis is zero. Thus, the ordinate of this point is 0.
Medium
Example 1: In which quadrants will the points (−1, −1), (0, −7), (3, 4), (−2, 0) and (0, 3) lie? Also identify the abscissa and ordinate of each point.
Solution:
We know that the coordinates of a point are written as (x, y), where x is the abscissa and y is the ordinate.
In case of point (−1, −1), x = −1 and y = −1.
Since x < 0 and y < 0, this point lies in quadrant III.
In case of point (0, −7), x = 0 and y = −7.
Since x = 0 and y < 0, this point lies on the negative y-axis (not in any specific quadrant).
In case of point (3, 4), x = 3 and y = 4.
Since x > 0 and y > 0, this point lies in quadrant I.
In case of point (−2, 0), x = −2 and y = 0.
Since x < 0 and y = 0, this point lies on the negative x-axis (not in any specific quadrant).
In case of point (0, 3), x = 0 and y = 3.
Since x = 0 and y > 0, this point lies on the positive y-axis (not in any specific quadrant).
Whiz Kid
The distance between any two points on the Cartesian plane, with coordinates (x1, y1) and (x2, y2), is given by √(x2 – x1)2 + (y2 – y1)2
For example, the distance between the point A (7, −1) and the origin O (0, 0) is given as follows:
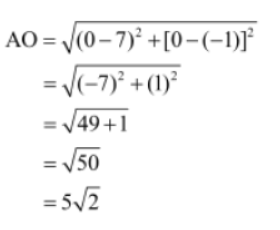
Activity
Do this activity to practise locating points on the coordinate plane.
•Take some marbles (or buttons) and arrange them in a grid, i.e., in rows and columns. The number of marbles should be the square of an odd number, say n.
• Let (n+1/2)th row be the x-axis and (n+1/2)th column be the y-axis.
• Let the marble lying at the point of intersection of the x and y axes be the origin O (0, 0).
• Now, find the coordinates of all the marbles with respect to the x-axis, y-axis and origin.
Solved Examples
Medium
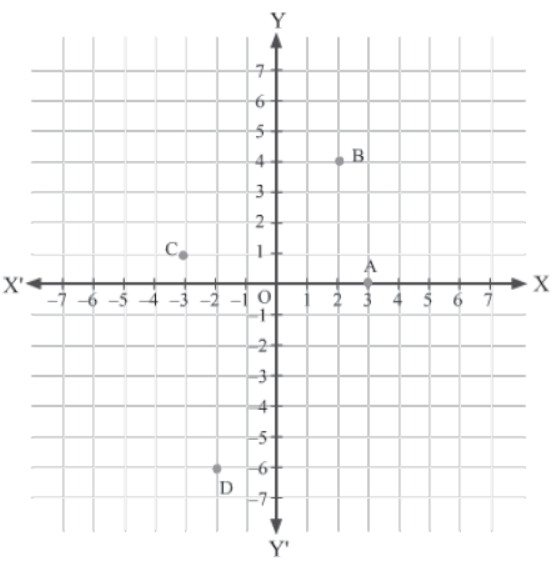
Example 1: Write the coordinates of the points A, B, C and D plotted in the figure.
Solution:
Draw perpendiculars from A, B, C and D to the coordinate axes as shown in the figure.
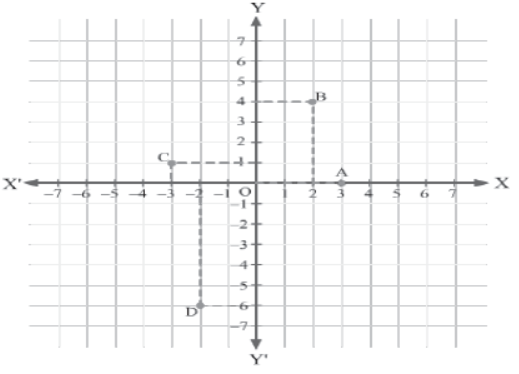
We know that the x-coordinate (or the abscissa) of a point is the perpendicular distance of
the point from the y-axis. Let the abscissa of each of the given points be a.
Also, the y-coordinate (or the ordinate) of a point is the perpendicular distance of the point
from the x-axis. Let the ordinate of each of the given points be b.
The coordinates of each of the given points will then be written as (a, b).
In case of point A, a = 3 and b = 0.
So, the coordinates of A are written as (3, 0).
In case of point B, a = 2 and b = 4.
So, the coordinates of B are written as (2, 4).
In case of point C, a = −3 and b = 1.
So, the coordinates of C are written as (−3, 1).
In case of point D, a = −2 and b = −6.
So, the coordinates of D are written as (−2, −6).
Plotting of Points on a Coordinate Plane
Plotting of Points
Take a look at the following grid of squares containing different items.
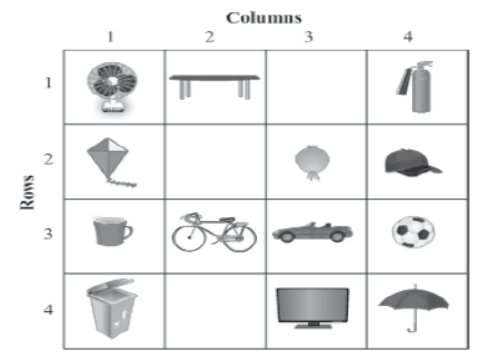
Certain squares in the grid are empty. Suppose one of these squares contained an orange before it was taken out, and we need to find that particular square. Let us say the orange was in Column 2. Using this information, can we locate the correct square? No, we cannot.
The given information is insufficient as there are two empty squares in Column 2. To correctly locate the required square, we also need to know the row number. Let us say that the orange was in Row 4. This information helps us to single out the fourth square in the second column. We were able to identify the correct square with the help of two attributes—the column number and the row number. In the same way, to plot a point on a graph, we require both its attributes—the abscissa and the ordinate.
In this lesson, we will learn how to plot points with given coordinates on the coordinate plane.
Did You Know?
Global Positioning System (GPS): GPS is a navigation system developed and maintained by the United States government. It is made up of a network of twenty-four satellites placed in Earth’s orbit. Anyone with a GPS receiver can freely access GPS.
GPS satellites transmit precise microwave signals. A GPS receiver uses these signals and ‘triangulates’ to determine the user’s exact location and time. The result is accurate to within ten to hundred metres.
GPS receivers determine 2D position (longitude and latitude) by using signals from at least three satellites in view. They ascertain 3D position (longitude, latitude and altitude) from four or more satellites.
GPS is used in clock synchronization, vehicle and aircraft tracking, map making, robotics, etc.
Solved Examples
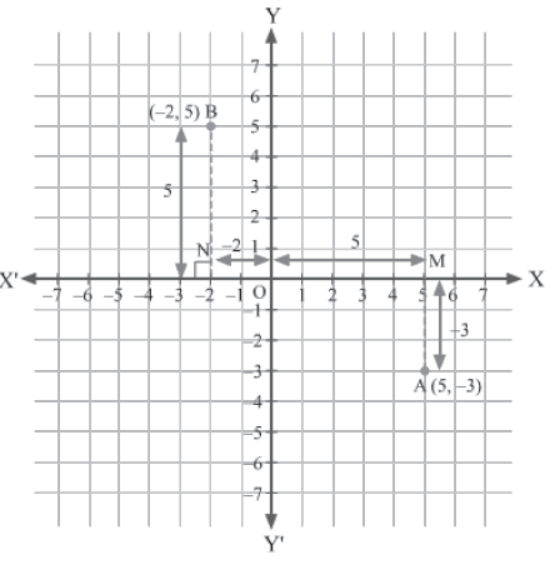
Example 1: Plot the points A (5, −3) and B (−2, 5) on the Cartesian plane.
Solution:
To plot A (5, −3):
(1) Move 5 units along OX and mark the endpoint as M.
(2) From M and perpendicular to the x-axis, move 3 units along OY’. Mark the endpoint as A; this is the location of the point (5, −3) on the Cartesian plane.
To plot B (−2, 5):
(1) Move 2 units along OX’ and mark the endpoint as N.
(2) From N and perpendicular to the x-axis, move 5 units along OY. Mark the endpoint as B; this is the location of the point (−2, 5) on the Cartesian plane.
Points A and B are plotted in the following graph.
Medium
Example 1: Draw a quadrilateral ABCD having vertices as A(0, 5), B(6, 5), C(4, –1) and D(–2, –1). Also, name the type of the quadrilateral ABCD.
Solution: According to the given coordinates, the points A, B, C and D can be plotted as in the given graph.
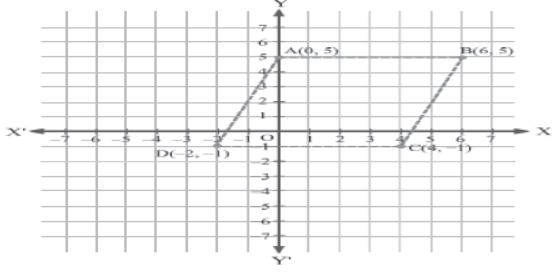
It can be observed that the distance of points B and C from points A and D respectively is 6 units.
Thus, AB = CD.
From the figure, it can be seen that CD intersects y-axis at F(0, –1). Also, perpendicular drawn from B meets CD extended at E.
Now, the distance of points A and B from points F and E respectively is 6 units.
Thus FA = EB
This shows that distance between the sides AB and CD is equal at different points and hence, these sides are parallel.
Since, one pair of opposite sides (AB and CD) is equal and parallel in quadrilateral ABCD, it is a parallelogram.
Example 2: Ravi travels 9 km east and then 5 km north. Sheena travels 4 km east and then 2 km south. They both start from the same point of origin. Write the coordinates of each person’s destination with reference to the magnetic compass.
Take 1 km as 1 unit.
Solution:
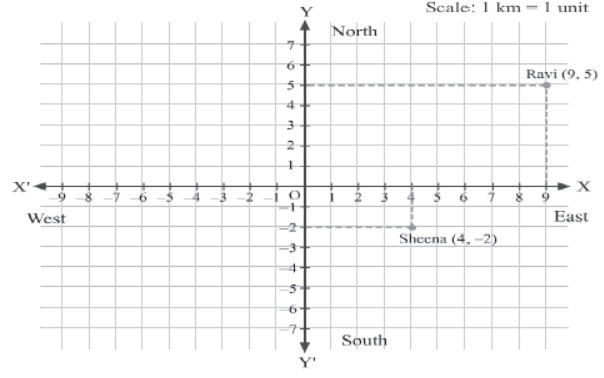
Let the coordinates of Ravi’s destination be (x, y).
Ravi travels 9 km east, i.e., along OX; so, x = 9.
He then travels 5 km north, i.e., along OY; so, y = 5.
Hence, (x, y) = (9, 5)
Let the coordinates of Sheena’s destination be (a, b).
Sheena travels 4 km east, i.e., along OX; so, a = 4.
She then travels 2 km south, i.e., along OY’; so, b = −2.
Hence, (a, b) = (4, −2)


