Students can refer to the following MCQ Questions for Relations and Functions with Answers Class 11 Maths MCQ Questions with Answers provided below based on the latest curriculum and examination pattern issued by CBSE and NCERT. Our teachers have provided here a collection of multiple choice questions for Relations and Functions with Answers Class 11 covering all topics in your textbook so that students can assess themselves on all important topics and thoroughly prepare for their exams
Relations and Functions with Answers Class 11 Maths MCQ Questions with Answers
We have provided below Relations and Functions with Answers Class 11 Maths MCQ Questions with answers which will help the students to go through the entire syllabus and practice multiple choice questions provided here with solutions. As Relations and Functions with Answers MCQs in Class 11 pdf download can be really scoring for students, you should go through all problems provided below so that you are able to get more marks in your exams.
Relations and Functions with Answers Class 11 Maths MCQ Questions
Question: Let R be the relation from A = {2, 3, 4, 5} to B = {3, 6, 7,10} defined by ‘x divides y’,then R-1 is equal to
(a) {(6, 2), (3, 3)}
(b) {(6, 2), (10, 2), (3, 3), (6, 3), (10, 5)}
(c) {(6, 2), (10, 2), (3, 3), (6, 3)}
(d) None of the above
Answer
B
Question: R is a relation from {11, 12, 13} to {8, 10, 12} defined by y = x – 3. The relation R-1 is
(a) {(11, 8), (13, 10)}
(b) {(8, 11), (10, 13)}
(c) {(8, 11), (9, 12), (10, 13)}
(d) None of these
Answer
B
Question: If R is a relation from a set A to the set B and S is a relation from B to C, then the relation SoR
(a) is from C to A
(b) is from A to C
(c) does not exist
(d) None of these
Answer
B
Question: Let a relation R be defined by
R = {(4, 5), (1, 4), (4, 6), (7, 6), (3, 7)}. The relation R-1oR is given by
(a) {(1, 1), (4, 4), (7, 4), (4, 7), (7, 7)}
(b) {(1, 1), (4, 4), (4, 7), (7, 4), (7, 7), (3, 3)}
(c) {(1, 5), (1, 6), (3, 6)}
(d) None of the above Functions
Answer
B
Question: Let f (x) = √1 + x2 then
(a) f(xy ) = f(x) × f( y )
(b) f(xy ) ³ f(x) × f( y )
(c) f(xy ) £ f(x) × f( y )
(d) None of these
Answer
C
Question: Let R be a relation on N defined by x + 2y = 8. The domain of R is
(a) {2, 4, 8}
(b) {2, 4, 6, 8}
(c) {2, 4, 6}
(d) {1, 2, 3, 4}
Answer
C
Question: The relation Rdefined on set A = {x : lxl <3,x ∈I}by R = {(x, y) : y = lxl} is
(a) {(- 2, 2), (- 1, 1), (0, 0), (1, 1), (2, 2)}
(b) {(- 2, – 2), (- 2, 2), (- 1, 1), (0, 0), (1, – 2), (1, 2),
(2, -1), (2, – 2)}
(c) {(0, 0), (1, 1), (2, 2)}
(d) None of the above
Answer
A
Question:The relation R defined on the set of natural numbers as {(a, b) : a differs from b by 3}, is given by
(a) {(1, 4), (2, 5), (3, 6), . . . . }
(b) {(4, 1), (5, 2), (6, 3), . . . . }
(c) {(1, 3), (2, 6), (3, 9), . . . . }
(d) None of the above
Answer
B
Question: Domain of √a2 -x2 (a > 0) is
(a) (-a, a)
(b) [-a, a]
(c) [0, a]
(d) (-a, 0]
Answer
B
Question:
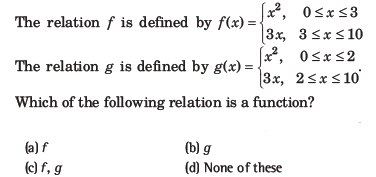
Answer
A
Question: The domain of the function f defined by f (x)=√4-x+1/√x2-1is equal to
(a) (-∞ -1)∪(1, 4]
(b) (-∞, -1]∪(1, 4]
(c) (–∞, -1)∪[1, 4]
(d) (–∞, -1)∪[1, 4)
Answer
A
Question: The domain and range of the real function f defined by f (x)=4-x/x-4 is given by
(a) Domain = R, Range = {-1, 1}
(b) Domain = R – {1}, Range = R
(c) Domain R=- { }4 , Range ={-}1
(d) Domain = R – {-4}, Range = {-1, 1}
Answer
C
Question: The domain of the function f given by f (x)=x2+2x+1/x2-x-6
(a) R – {3, – 2}
(b) R – {-3, 2}
(c) R – [3, – 2]
(d) R – (3, – 2)
Answer
A
Question: The domain and range of the function f given by f (x) = 2 -|x – 5|is
(a) Domain = R+ , Range = (-∞, 1]
(b) Domain = R, Range = (-∞, 2]
(c) Domain = R , Range = (–∞, 2)
(d) Domain = R+ , Range = (–∞, 2]
Answer
B
Question: If f (x) = ax + b, where a and b are integers, f (-1) = – 5 and f (3) = 3, then a and b are equal to
(a) a = – 3,b = -1
(b) a = 2, b = – 3
(c) a = 0 , b = 2
(d) a = 2, b = 3
Answer
A
Question:
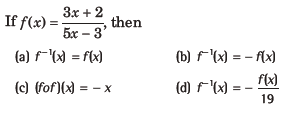
Answer
A
Question: Given, f (x)= log(1+x/1-x)and g (x)3x+x3/1+3x2‘ then fog(x) equals
(a) -f(x)
(b) 3f(x)
(c) [f(x)]3
(d) None of these
Answer
B
Question: If f : R → R is given by
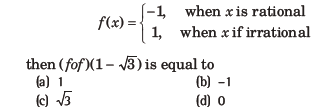
Answer
B
Question: If f : R → R, g : R → R and h : R → R are such that f (x) = x2, g(x) = tan x and h(x) = log x, then the value of (ho( gof ))(x), if x =√π/4 will be
(a) 0
(b) 1
(c) –1
(d) p
Answer
A
Question: If [x]2-5 [x] + 6 = 0, where [ · ] denote the greatest integer function, then
(a) x ∈3, 4]
(b) x ∈(2, 3]
(c) x ∈[2, 3]
(d) x ∈[2, 4)
Answer
D
Question: Let f (x) = x2 – x + 1, x ≥1/2, then the solution of the equation f (x) = f -1(x) is
(a) x = 1
(b) x = 2
(c) x = 1/2
(d) None of these
Answer
A
Question: The domain for which the functions defined by f (x) = 3x2 -1 and g(x) = 3 + x are equal to
(a){-1,4/3}
(b){-1,-4/3}
(c){1,4/3}
(d){1,-4/3}
Answer
A
Question: Range of f (x)=1/1-2cos x is
(a) [1/3,1]
(b) [-1,1/3]
(c) (-∞,-1)∪[1/3,∞)
(d)[-1/3,1]
Answer
B
Question:
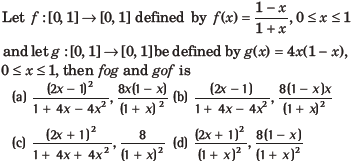
Answer
A
Question: Let f : R → R, g : R → R be two functions such that f (x ) = 2x -3, g(x ) = x3 + 5 .
The function ( fog)-1(x) is equal to

Answer
D
Question: A real valued function f (x) satisfies the functional equation
f (x – y) = f (x) f ( y) – f (a – x) f (a + y)
where a is a given constant and f (0) = 1, f (2a – x) is equal to
(a) f(-x)
(b) f(a) + f(a – x)
(c) f(x)
(d) -f(x)
Answer
D
Question: If f : R → R satisfies f (x + y) = f (x) + f ( y), for all x y ∈ R , and f ( 1)= 7 = then n∑=f (r) is
r=1
(a)7n/2
(b)7(n+1)/2
(c) 7n(n + 1)
(d)7n (n+1/2
Answer
B
Question: The inverse of the function y=10x-10-x/10x+10-xis
(a) log10 (2 – x)
(b)1/2 log101+x/1-x
(c)1/2log10 (2x – 1)
(d)1/4log(2x/2-x)
Answer
B
Question: If f (x) = cos (log x), then f (x) f (y)-1/2[f(x/y)+f(xy)] has the value 2
(a) -1
(b)1/2
(c) –2
(d) 0
Answer
D
Question: Let f : N → Y be a function defined as f (x) = 4x + 3 where If f is invertible, then its inverse is
(a) g (y)=y-3/4
(b) g (y)=3y+4/3
(c) g (y)=4+y+3/4
(d) g (y)=y+3/4
Answer
A

