Please refer to Class 12 Mathematics Sample Paper Term 1 With Solutions Set C provided below. The Sample Papers for Class 12 Mathematics have been prepared based on the latest pattern issued by CBSE. Students should practice these guess papers for class 12 Mathematics to gain more practice and get better marks in examinations. The Term 1 Sample Papers for Mathematics Standard 12 will help you to understand the type of questions which can be asked in upcoming examinations.
Term 1 Sample Paper for Class 12 Mathematics With Solutions Set C
Section A
1. The least value of m satisfying 150 = 10 (mod m) is
(a) 4
(b) 5
(c) 7
(d) 2
Answer
D
2. 13 mod 20 is
(a) 0
(b) 7
(c) 13
(d) 6
Answer
C
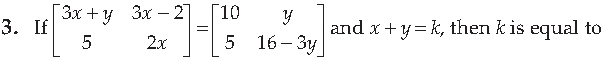
(a) 1
(b) 6
(c) 0
(d) 5
Answer
B
4. From a lot of 30 bulbs which includes 6 defectives, a sample of 4 bulbs is drawn at random one-by-one with replacement. Find the probability distribution of the number of defective bulbs. Hence, the mean of the distributions, will be
(a) 3/5
(b) 1/5
(c) 2/5
(d) 4/5
Answer
D
5. If X is the number of tails in three tosses of a coin, then the standard deviation of X is
(a) 3/4
(b) 2 3
(c) 3/6
(d) 3/2
Answer
D
6. There are 50 telephone lines in an exchange. The probability that any one of them will be busy is 0.1. The probability that all the lines are busy is

Answer
C
7. Let Z be a standard normal variate. If P(0 < Z < t) = 0.4265, then value of t is
(a) -1.45
(b) 1.32
(c) 1.56
(d) 1.45
Answer
D
8. If the wholesale price index for first week is 400 and for second week is 450, then rate of inflation is
(a) 16%
(b) 14%
(c) 12.50%
(d) 15.25%
Answer
C
9. Bowley’s index number is based on
(a) GM of Laspeyre’s and Paasche’s index
(b) AM of Laspeyre’s and Paasche’s index
(c) HM of Laspeyre’s and Paasche’s index
(d) None of the above
Answer
B
10. A composite price index where the prices of the items in the composite are weighted by their relative importance is known as the
(a) price relative
(b) wholesale price index
(c) weighted aggregate price index
(d) None of these
Answer
C
11. If x = 2at and y = at2 , then dy/dx is equals to
(a) 1/t2
(b) t
(c) 1/t
(d) -1/t2
Answer
B

Answer
B
13. The Demand matrix which is consistent with the technology matrix
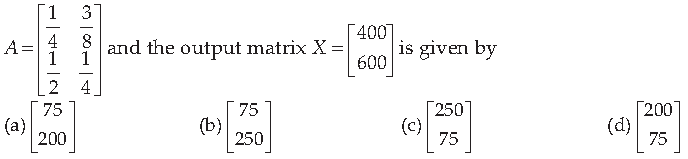
Answer
B
14. The slope of the normal at the point ‘t’ on the curve x = 1/t and y = t is
(a) 1/t
(b) t
(c) 1/t2
(d) t2
Answer
C
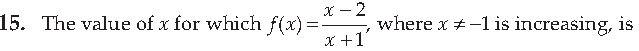
(a) R
(b) R – {- 1}
(c) (-C, -1)
(d) (-1, ∞)
Answer
B
16. The point of local minima for f (x) = x3 – x 3 is
(a) -1
(b) 1
(c) -1 and 1
(d) None of these
Answer
B
17. Solution set of | x – 1|£ 5 is
(a) [-4, 6]
(b) (-4, 6)
(c) [-4, ∞)
(d) [6, ∞)
Answer
A
18. A cover’s a distance of 1 km in 4 min 54 sec and B in 5 min. In a race of 1000 km than B should stand ahead of A, so that both reach last point together
(a) 20 m
(b) 25 m
(c) 15 m
(d) 10 m
Answer
A
19. Mr X and Mr Y start a business by investing ₹ 40000 and ₹ 50000 respectively. They decide to keep 50% of the profit in equal amount and remaining is distributed between them, in the ratio of their investment. If Mr X gets ₹ 800 less than Mr Y. Then, total profit of Mr X is
(a) ₹ 5800
(b) ₹ 7800
(c) ₹ 4800
(d) ₹ 6800
Answer
D
20. Suppose 2 ´ 3matrix A = [aij], whose elements are given by
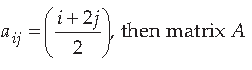

Answer
A
Section B
21. The unit’s digit is 7291 is
(a) 0
(b) 7
(c) 3
(d) 9
Answer
C
22. The remainder when 225 + 1 is divided by 641 is
(a) 0
(b) 1
(c) 2
(d) 3
Answer
A

(a) 1
(b) 2
(c) -1
(d) 0
Answer
D
24. If the average cost function is given by AC(x) x= 2x – 11+ (50/x) , then the marginal cost,
MC(x), when x = 4, is
(a) 4
(b) 5
(c) 6
(d) 7
Answer
B
25. The value of k for which f (x) = kx3 – 9kx2 + 9x + 3 is increasing on R is

Answer
B
26. Two probability distributions of the discrete random variables X and Y are given below.
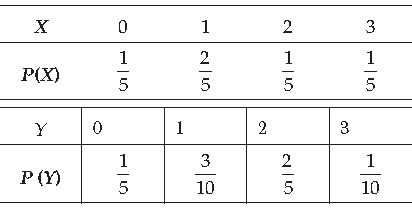
Then, E(Y2) is equal to
(a) E(X)
(b) 2 E(X)
(c) E(X)/2
(d) 3E(X)
Answer
B
27. If X follows a Binomial distribution with mean 4 and variance 2, then P(X > 7) is
(a) 3/256
(b) 5/256
(c) 7/256
(d) 9/256
Answer
D
28. In a distribution exactly normal, 10.03% of the items are under 25 kg weight and 10.03% of the items are above 70 kg weight. The mean and standard deviation of the distribution are
(a) 47.5 and 14.58
(b) 47.5 and 17.58
(c) 44.5 and 17.58
(d) 44.5 and 14.58
Answer
B
29. If X is a Poisson variable such that P(X = K) = P(X = K + 1), then variance of X is
(a) K – 1
(b) K
(c) K + 1
(d) K + 2
Answer
C
30. Given that ∑p1q1 = 408, ∑p0q0 = 320, ∑p1q0 = 401 and ∑p0q1 = 374, where subscript 0 and 1 are used for base year and current year respectively. The Paasche’s index number is
(a) 116.46
(b) 106.48
(c) 109.09
(d) 112.17
Answer
C
31. For data regarding ∑p1q0 = 500, ∑p0q0 = 450, ∑p1q0 = 480 and ∑p1q1 = 525, where subscript 0 and 1 are used for base year and current year respectively. The Laspeyre’s price index is
(a) 114.52
(b) 109.62
(c) 111.11
(d) 113.12
Answer
C
32. For given data ∑p0q0 = 340, ∑p1q0 = 355, ∑p0q1 = 350 and ∑p1q1 = 362, where subscript 0 and 1 are used for base year and current year respectively. The Bowley’s index number is
(a) 1.54
(b) 1.20
(c) 1.04
(d) 0.95
Answer
C
33. Given that ∑p0 q0 = 165, ∑p1q1 = 180 , ∑p0q1 = 130 and ∑p1q0 = 162, where subscript 0 and 1 are used for base year and current year respectively. The Edgeworth’s index number is
(a) 110.13
(b) 121.42
(c) 115.93
(d) 118.36
Answer
C
34. A simple aggregate quantity index is used to
(a) measure the change in price of a product
(b) measure the change in quantity of product
(c) measure the overall change in price of range of products
(d) measure the overall change in quantity of range of product
Answer
C
35. If xy = 1, then dy/dx is equal to
(a) x2
(b) y2
(c) -x2
(d) -y2
Answer
D
36. For the system of equations 2x + 5y = 9, x + y = 3 the value of x and y are respectively
(a) 0 and 1
(b) -1 and 2
(c) 1 and 2
(d) 2 and 1
Answer
D
37. For the system of equations x + y + z = 3, x – 2y + 3z = 2 and 2x – y + z = 2, then the value of x, y and z are respectively
(a) 1, 1 and 0
(b) 1, 1 and 1
(c) 1, 2 and 3
(d) 3, 1 and 1
Answer
B
38. Pipes A and B can fill a tank in 15 and 12 min, respectively. Pipe C empties the tank at the rate of 2 L/min. If all the pipes are opened at the same time, then the tank is filled in 8 (4/7) min. The capacity of tank is
(a) 80 L
(b) 75 L
(c) 60 L
(d) 90 L
Answer
C
39. One type of liquid contains 20% water and the second type of liquid contains 35% of water. A glass is filled with 10 parts of first liquid and 4 parts of second liquid. The water in the new mixture in the glass is

Answer
B
40. The value of k for which the following function is continuous at x = 3

(a) 12
(b) 10
(c) 8
(d) 6
Answer
A
Section C
41. Abhishek started a business by investing ₹ 50000. After one year, he invested another ₹ 80000 and Sudhir also joined him with a capital of ₹ 70000. If the profit earned in three years from the starting of business was ₹ 87500, then the share of Sudhir in the profit is
(a) ₹ 37500
(b) ₹ 32500
(c) ₹ 38281
(d) ₹ 35000
Answer
D
42. Find the remainder when 987 + 876 + 765 + 654 + 543 + 432 + 321 + 210 is divided by 6
(a) 0
(b) 1
(c) 3
(d) 5
Answer
A
43. Consider the probability distribution of a random variable X
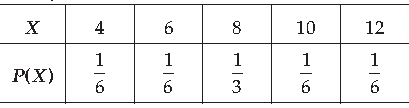
The value of Var(X) is
(a) 5/3
(b) 10/3
(c) 20/3
(d) 25/3
Answer
C
44. In a normal distribution 31% of the items are under 45 and 8% are over 64. The mean and standard deviation of the distribution are
(a) 50 and 10
(b) 55 and 10
(c) 45 and 5
(d) 54 and 5
Answer
A
45. For the curve y = 4x3 – 2x5 , the points at which the tangent passes through the origin is/are
(a) (0, 0)
(b) (1, 2)
(c) (-1, -2)
(d) All of these
Answer
D
Case Study
A man can row against the current, three fourth of a kilometer in 15 min and returns same distance in 10 min.

Based on the above information answer the following question.
46. The ratio of speeds in downstream to the speed in upstream is
(a) 1 : 3
(b) 2 : 3
(c) 3 : 2
(d) 3 : 4
Answer
C
47. The speed of the current is

Answer
A
48. The speed of a man in still water is

Answer
B
49. If a man can row a distance of 3 km against the stream, then he would cover the distance in
(a) 55 min
(b) 50 min
(c) 1 h
(d) 1 h 15 min
Answer
D
50. If the time taken by a man to cover 4.5 km downstream and x km upstream is same, then the value of x is
(a) 2.5
(b) 5
(c) 3
(d) 4
Answer
C

