Class 12 Mathematics Sample Paper With Solutions Set D

Please refer to Class 12 Mathematics Sample Paper With Solutions Set D provided below. The Sample Papers for Class 12 Mathematics have been prepared based on the latest pattern issued by CBSE. Students should practice these guess papers for class 12 Mathematics to gain more practice and get better marks in examinations. The Sample Papers for Mathematics Standard 12 will help you to understand the type of questions which can be asked in upcoming examinations.

1. Equation of straight line ax + by + c = 0 where 3a+4b+ c = 0, which is at maximum distance from (1, –2), is
(a) 3x + y – 17 = 0
(b) 4x + 3y – 24 = 0
(c) 3x + 4y – 25 = 0
(d) x + 3y – 15 = 0   

2. 

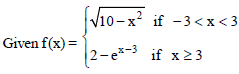
The graph of f (x) is
(a) continuous and differentiable at x = 3
(b) continuous but not differentiable at x = 3
(c) differentiable but not continuous at x = 3
(d) neither differentiable nor continuous at x = 3 

3. The solution of the equation 2z = | z | + 2i, where z is a complex number, is
(a) z = √3/3 − i
(b) z = √3/3 + i
(c) z = √3/3 ± i
(d) None of these 

4. 

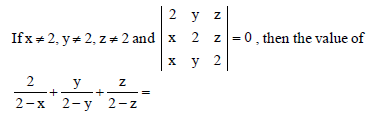
(a) 1
(b) 0
(c) 3
(d) 4  

5. Box contains 2 one rupee, 2 five rupee, 2 ten rupee and 2 twenty rupee coin. Two coins are drawn at random simultaneously. The probability that their sum is ` 20 or more, is
(a) 1/4
(b) 1/2
(c) 3/4
(d) 1/8 

6. The equation (5x – 1)² + (5y – 2)² = (λ² – 4λ + 4) (3x + 4y – 1)² represents an ellipse if λ∈  
(a) (0, 1]
(b) (–1, 2)
(c) (2, 3)
(d) (–1, 0) 

7. The value of the definite integral, 

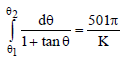
where θ₂= 1003π/2008 and θ₂ = π/2008. The value of K equals
(a) 2007
(b) 2006
(c) 2009
(d) 2008 

8. The straight line y = m (x – a) meets the parabola y² = 4ax in two distinct points for
(a) all m Î R
(b) all m Î [–1, 1]
(c) all m Î R – {0}
(d) None of these 

9. The expansion of (1 + x)ⁿ has 3 consecutive terms with coefficients in the ratio 1 : 2 : 3 and can be written in the form ⁿC_k : ⁿC_k+1 : ⁿC_k+2. The sum of all possible values of (n + k) is
(a) 18
(b) 21
(c) 28
(d) 32 

10. The mean and standard deviation of 6 observations are 8 and 4 respectively. If each observation is multiplied by 3, find the new standard deviation of the resulting observations.
(a) 12
(b) 18
(c) 24
(d) 144 

11. p ∨ (p ∧ q) is equivalent to
(a) q
(b) p
(c) ~p
(d) ~q 

12. 

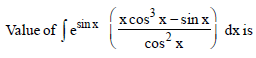
(a) x e^{sin x} – e^{sin x} sec x + C
(b) x e^{cos x} – e^{sin x} sec x + C
(c) x² e^{sin x} + e^{sin x} sec x + C
(d) 2x e^{sin x} – e^{sin x} tan x + C 

13. The function f :[2, ∞)→(0, ∞) defined by f (x) = x² – 4x + a, then the set of values of ‘a’ for which f(x) becomes onto is
(a) (4,¥)
(b) [4, ¥)
(c) {4}
(d) f 

14. If α and β are the real roots of the equation
x² – (k – 2) x + (k² + 3k + 5) = 0 (k ∈ R) .
Find the maximum and minimum values of (α² + β² ) .
(a) 18, 50/9
(b) 18, 25/9
(c) 27, 50/9
(d) None of these 

15. The sum of the coefficient of all the terms in the expansion of (2x – y + z)20 in which y do not appear at all while x appears in even powers and z appears in odd powers is
(a) 0
(b) 2²⁰ − 1/2
(c) 2¹⁹
(d)3²⁰ − 1/2 

16. All the five digit numbers in which each successive digit exceeds is predecessor are arranged in the increasing order. The (105)^th number does not contain the digit
(a) 1
(b) 2
(c) 6
(d) All of these 

17. 

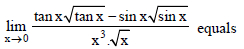
(a) 1/4
(b) 3/4
(c) 1/2
(d) 1 

18. Three people each flip two fair coins. The probability that exactly two of the people flipped one head and one tail, is
(a) 1/2
(b) 3/8
(c) 5/8
(d) 3/4 

19. If a̅, b̅, c̅  are non-coplanar unit vector such that a̅ × (b̅ × c̅) = 1/√2 (b̅ + c̅)  then the angle between the vectors a̅, b̅  is
(a) 3p/4
(b) p/4
(c) p/8
(d) p/2 

20. Statement-1 : If | z₁ | = 30, | z₂ – (12 + 5i) | = 6, then maximum alue of | z₁ – z₂ | is 49.
Statement-2 : If z₁, z₂ are two complex numbers, then | z₁ – z₂ | ≤| z₁| + | z₂ | and equality holds when origin, z₁ and z₂ are collinear and z₁, z₂ are on the opposite side of the origin.
(a) Statement-1 is false, Statement-2 is true.
(b) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1.
(c) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1.
(d) Statement-1 is true, Statement-2 is false. 

21. Consider the family of straight lines
2x sin²θ + y cos²θ = 2 cos ²θ
Statement-1 : All the lines of the given family pass through the point (3, –2).
Statement-2 : All the lines of the given family pass through a fixed point.
(a) Statement-1 is false, Statement-2 is true.
(b) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1.
(c) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1.
(d) Statement-1 is true, Statement-2 is false. 

22.

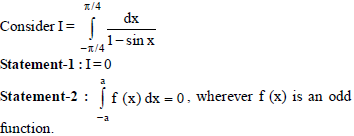
(a) Statement-1 is false, Statement-2 is true.
(b) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1.
(c) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1.
(d) Statement-1 is true, Statement-2 is false. 

23. Statement-1 : Let f : R → R be a function such that 
f (x) = x³ + x² + 3x + sin x. Then f is one-one.
Statement-2 : f (x) neither increasing nor decreasing function.
(a) Statement-1 is false, Statement-2 is true.
(b) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1.
(c) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1.
(d) Statement-1 is true, Statement-2 is false. 

π. Statement-1 : If the lengths of subtangent and subnormal at point (x, y) on y = f (x) are respectively 9 and 4. Then x = ± 6
Statement-2 : Product of sub tangent and sub normal is square of the ordinate of the point.
(a) Statement-1 is false, Statement-2 is true.
(b) Statement-1 is true, Statement-2 is true; Statement-2 is a correct explanation for Statement-1.
(c) Statement-1 is true, Statement-2 is true; Statement-2 is not a correct explanation for Statement-1. 
(d) Statement-1 is true, Statement-2 is false. 

25. Consider the following statements :
S₁ : Number of integrals values of ‘a’ for which the roots of the equation x² + ax + 7 = 0 are imaginary with positive real parts is 5.
S₂ : Let α, β are roots x² – (a + 3) x + 5 = 0 and α, a, β are inA.P. then roots are 2 and 5/2
S₃ : Solution set of logx (2 + x) ≤ logx (6 – x) is (1, 2] 
State, in order, whether S₁, S₂, S₃ are true or false.
(a) FFT
(b) TFT
(c) TFF
(d) TTT 

26. 

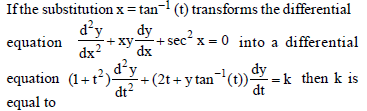
(a) –2
(b) 2
(c) –1
(d) 0 

27. Let f : R → R and f_n (x) = f (f_n–1 (x)) ∀ n ≥ 2, n ∈ N,
the roots of equation f₃(x) f₂(x) f (x) – 25f₂(x) f (x) + 175 f (x) = 375 which also satisfy equation f (x) = x will be
(a) 5
(b) 15
(c) 10
(d) Both (a) and (b) 

28. A triangle ABC satisfies the relation
2 sec 4C + sin² 2A + √sinB = 0 and a point P is taken on  the longest side of the triangle such that it divides the side in the ratio 1 : 3. Let Q and R be the circumcentre and orthocentre of Δ ABC. If PQ : QR : RP = 1 : α∈: β, then the value of α² + β², is
(a) 9
(b) 8
(c) 6
(d) 7 

29.

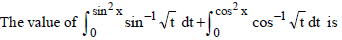
(a) π
(b) π/2
(c) π/4
(d) 1 

30. If a is real and √2ax + sinBy + cosBz = 0 ,
x + cosBy + sinBz = 0 , – x + sin By – cos Bz = 0, then the set of all values of a for which the system of linear equations has a non-trivial solution, is
(a) [1, 2]
(b) [–1, 1]
(c) [1, ∞)
(d) [2^–1/2, 2^1/2]