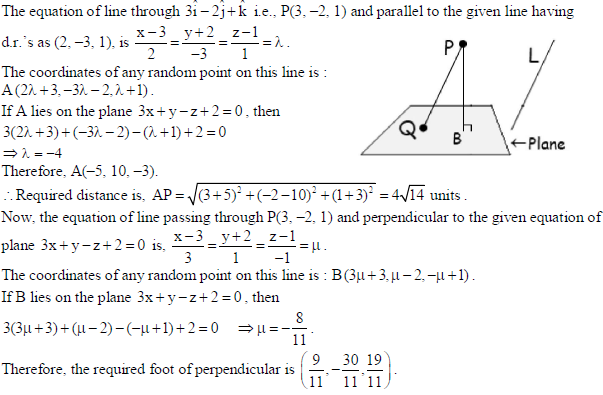Class 12 Mathematics Sample Paper With Solutions Set E

Please refer to Class 12 Mathematics Sample Paper With Solutions Set E provided below. The Sample Papers for Class 12 Mathematics have been prepared based on the latest pattern issued by CBSE. Students should practice these guess papers for class 12 Mathematics to gain more practice and get better marks in examinations. The Sample Papers for Mathematics Standard 12 will help you to understand the type of questions which can be asked in upcoming examinations.

PART – A
Section I

1. Find the number of non-reflexive relations defined on a set with three elements.
Answer: Number of non-reflexive relations defined on a set of n elements = 2^n² − 2ⁿ⁽ⁿ⁻¹⁾ .
So, for a set with 3 elements, no. of non-reflexive relations = 2^3² − 2³⁽³⁻¹⁾
                                                                                      = 2⁹ − 2⁶
                                                                                      = 64×7
                                                                                     = 448 .

2. Let f :R →(−π, 0) be defined as f (x) = cot⁻¹ x .
Then find the value of f (−1) .
Answer: Given that, f :R →(−π, 0) defined as f (x) = cot⁻¹ x
Now f (−1) cot⁻¹(−1) = cot ⁻¹ (cot(−π/4)) = π/4
Note that here the range of f (x) is (−π, 0) , which is other than principal value branch range of cot⁻¹ .

OR

If sin ⁻¹ x = π/5 , x ∈ (−1,1) then, find cos⁻¹ x .
Answer: sin ⁻¹ x = π/5 , x ∈ (−1,1)
⇒ sin sin ⁻¹x = sin π/5
⇒ x = sin π/5
⇒ x = cos(π/2 − π/5)
⇒ cos ⁻¹ x = cos ⁻¹ cos(3π/10)
⇒ cos ⁻¹ x = 3π/10.

3. Let R be the relation defined on Q (set of rational numbers) as a R b ⇔ |a − b| ≤ 1/2.
Then state the reason why R is not a transitive relation?
Answer: Let a=1, b =2/3 and c = 1/4.

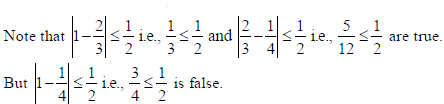
That is, aRb and bRc does not necessarily imply aRc for all a, b,c ∈ Q .
Therefore, R is not transitive

OR

Discuss the surjectivity of f : Z → Z given as f (x) = 3x + 2 ∀ x ∈ Z.
Answer: f (x) = 3x + 2 ∀ x ∈ Z, f : Z → Z Let y be an element of codomain Z such that y = f (x) = 3x + 2 .
⇒ x = y−2/3 ∉ Z ∀ y ∈ Z                  [When y = 0 ∈ Z, x = 2/3 ∉ Z
So, y = 0 (which lies in the codomain Z) doesn’t have its pre-image in the domain Z.
Hence f isn’t surjective.

4. Find the value of |A||adj.A| if 

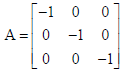
Answer: 

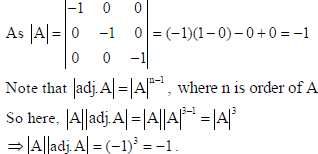
5. If order of A, B and C are 4×3, 5× 4 and 3×7 respectively then, find the order of C'(A’×B’) .
Answer: Order of A’, B’ and C’ are 3× 4, 4×5 and 7×3 respectively.
So, order of (A’×B’) is 3×5 .
Therefore, the order of C'(A’×B’) is 7×5 .

OR

Write the ‘sum of cofactors’ of element ‘13’ and ‘1’ in 

Answer: 

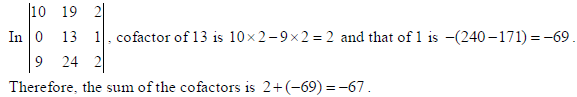
cofactor of 13 is 10×2 −9×2 = 2 and that of 1 is −(240 −171) = −69 .
Therefore, the sum of the cofactors is 2+ (−69) = −67 .

6. It is given that at x =1, the function x⁴ − 62x² + a x + 9 attains its maximum value on the interval [0, 2]. Find the value of ‘a’.
Answer: Let f (x) = x⁴ − 62x² + a x + 9
⇒ f'(x) = 4x³ −124x + a
Since f (x) attains its maximum value at x = 1, so f ‘(1) = 4×1³ −124 x 1+ a = 0
∴ a =120 .

7.

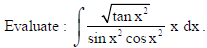
Answer: 

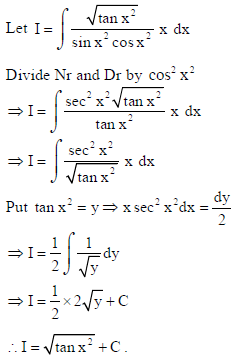
OR

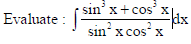
Answer: 

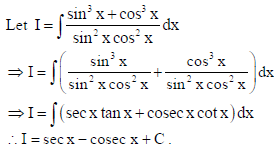
8. Using integration, find the area of the smaller region as depicted in the diagram below :  

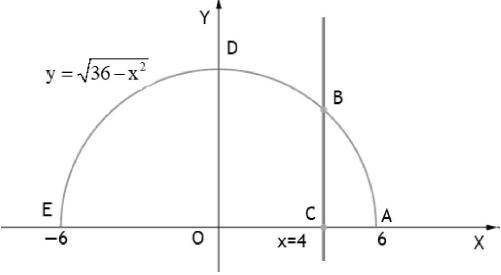
Answer: Clearly the smaller region is (ABCA).
So, required area = ar(ABCA) 

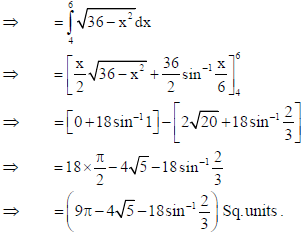
9. Solve the differential equation dy/dx + 2xy = y and express the result in the form of y = f (x) .
Answer: We have dy/dx + 2xy = y
⇒ dy/dx = y(1 − 2x)
⇒ ∫dy/y = ∫(1 − 2x)dx
⇒ log y = x − x² + k
⇒ y = e^{x−x² +k}
⇒ y = e^x−x² × e^k
That is, y = Ce^x−x² , where e^k = C.

10. What is a ∈ R , such that |ax̅| =1, where x̅ = î − 2j+ 2k̂ ?
Answer: Given that |ax̅| = 1

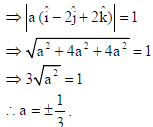
11. If |a̅| = 2,|b̅| = 2√3  and a̅ ⊥ b̅, then write the value of |a̅ + b̅|.
Answer: Let y = |a̅ + b̅|
⇒ y² =|a̅ + b̅|² 
⇒ y² = (a̅ + b̅).(a̅ + b̅)
⇒ y² = |a̅|² + |b̅|² + 2a̅.b̅
⇒ y² = 4 +12+ 2×0                       [ a̅ ⊥ b̅  ∴ a̅.b̅ = 0
⇒ y = 4
∴ |a̅ + b̅| = 4

OR

Let  a̅ = î + xĵ and b̅ = 2î − ĵ+ 2k̂  be two vectors such that the projection of a̅ on b̅ is 2. Then,
find the value of x.
Answer: Projection of a̅ on b̅ = a̅.b̅ = 2

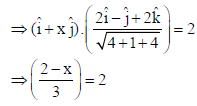
∴ x = −4 .

12. X and Y are two points with position vectors 3a̅ + b̅ and a̅ −3b̅ respectively. Write the position vector of a point Z which divides the line segment XY in the ratio 2 : 1 externally.
Answer: Here O̅X̅ = 3a̅ + b̅ and O̅Y̅ = a̅−3b̅
As Z which divides the line segment XY in the ratio 2 : 1 externally,
⇒ O̅Z̅ = 2O̅Y̅ − O̅X̅/2−1 = 2(a̅−3b̅) (3a̅−b̅)/1
⇒ O̅Z̅ = −a̅ − 7b̅.

13. If the product of the distances of the point (1, 1, 1) from the origin and the plane x − y + z + k = 0 be 5, then determine the value (s) of ‘k’.
Answer: Given point is P(1, 1, 1) and the plane is x − y + z + k = 0 . 

14. Find the vector equation of a line passing through the point (2, –3, –5) and perpendicular to theplane r̅ .(6î − 3ĵ+ 5k̂ ) + 2 = 0.
Answer: Since the required line passing through (2, –3, –5) is perpendicular to the given plane
r̅.(6î − 3ĵ+ 5k̂ ) + 2 = 0 so, the line must be parallel to the normal vector of the plane i.e.,
m̅ = 6î −3ĵ+ 5k̂ .
So, a̅ = 2î −3ĵ−5k̂, b̅ = m̅ = 6î −3ĵ+ 5k̂
Hence, by using r̅ = a̅ + λb̅
⇒ r̅ = 2î − 3ĵ− 5k̂ + λ(6î −3ĵ+ 5k̂) .

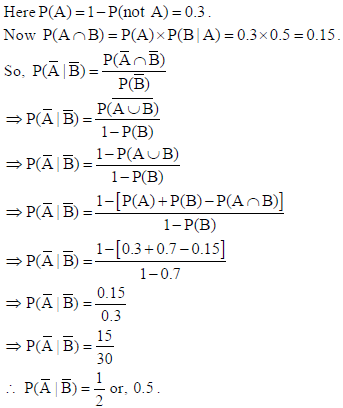
15. If P(not A) = 0.7, P(B) = 0.7 and P(B|A) = 0.5 , then find P( A̅|B̅ ).
Answer: 

16. From the set {1, 2, 3, 4, 5}, two numbers ‘a’ and ‘b’ (such that, a ≠ b ) are chosen at random.
Find the probability that a/b is an integer.
Answer: Total possible outcomes will be 5×4 = 20 .
For favorable cases,

Section II

Both the Case study based questions are compulsory. Attempt any 4 sub-parts from each question
17 (i-v) and 18 (i-v).

17. Meghna has two boxes I and II. Box I contains 3 ‘red and black’ and 6 ‘white and black’ balls. Box II contains 5 ‘red and black’ and ‘n’ balls are ‘white and black’ balls.

One of the two boxes, box I and box II is selected by her friend Radha at random, and then Radha draws a ball at random. The ball drawn is found to be ‘red and black’.
Based on the above information answer the following :

(i) Meghna notices that the probability of the ‘red and black’ ball taken out from the box II is 3/5. Then Radha asks her about the value of n. The value of ‘n’ is
(a) 1
(b) 3
(c) 5
(d) 6 

(ii) The probability that box I is selected given that the ball drawn is found to be ‘red and black’, is
(a) 3/5
(b) 2/5
(c) 1/5
(d) 1   

(iii) What is the probability that the ball drawn is found to be ‘red and black’?
(a) 5/12
(b) 7/12
(c) 5/21
(d) 12/5   

(iv) Let A be the event of getting a ‘red and black’ ball from then box.
Also let  E₁ and E₂ be the events that box I and box II is selected, respectively.

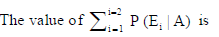
(a) 1
(b) 1/2
(c) 1/3
(d) 0 

(v) 

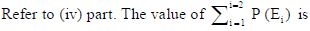
(a) 0
(b) 1/2
(c) 1
(d) 1/10 

18. There is a local printing press, whose owner is given a bulk order for printing of a magazine by a school of the same locality. He shows variety of pages to school administration.
Following is the pictorial description for a particular page, selected by school administration.  

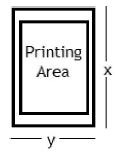
The total area of the page is 150 cm².
The combined width of the margin at the top and bottom is 3 cm and the side 2 cm.
Using the information given above, answer the following :

(i) The relation between x and y is given by
(a) (x −3)y =150
(b) xy =150
(c) x(y − 2) =150
(d) (x − 2)(y − 3) =150 

(ii) The area of page where printing can be done, is given by
(a) xy
(b) (x + 3)(y + 2)
(c) (x −3)(y − 2)
(d) (x −3)(y + 2) 

(iii) The area of the printable region of the page, in terms of x, is
(a) 156 + 2x + 450/x
(b) 156 − 2x + 3(150/x)
(c) 156 − 2x − 15(3/x)
(d) 156 − 2x − 3(150/x) 

(iv) For what value of ‘x’, the printable area of the page is maximum?
(a) 15 cm
(b) 10 cm
(c) 12 cm
(d) 15 units 

(v) What should be dimension of the page so that it has maximum area to be printed?
(a) Length =1 cm, width =15 cm
(b) Length =15 cm, width =10 cm
(c) Length =15 cm, width =12 cm
(d) Length =150 cm, width =1 cm 

PART – B
Section III

19. Find the value of tan(2 tan⁻¹1/5 −π/4).
Answer: 

OR

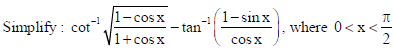
Answer: 

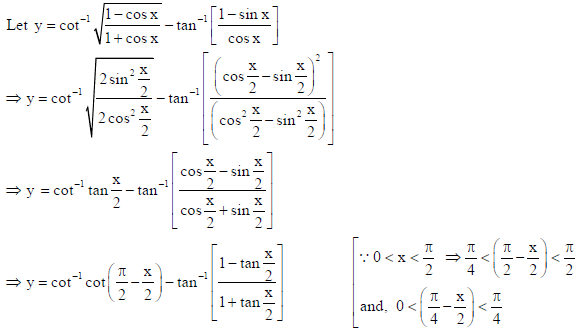
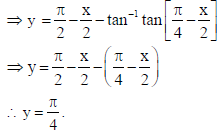
20. 

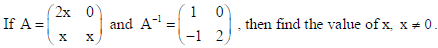
Answer: 

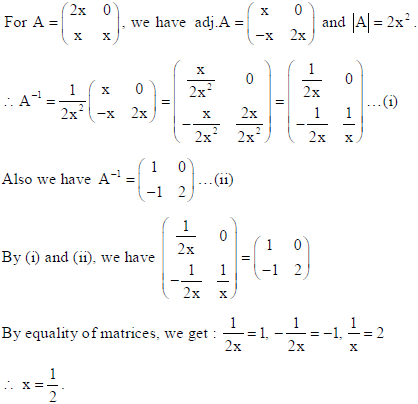
21. Find the value of lim_x→0 f (x) and lim_x→0 f(x) ,where

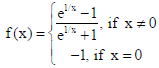
Hence, discuss the continuity of f (x) at x = 0.
Answer:

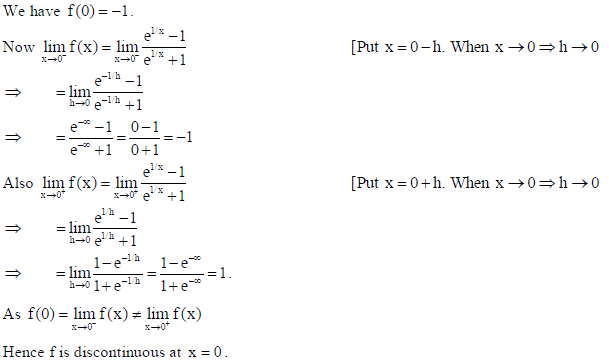
22. Find the interval in which the function f (x) = x e^x(1−x) is increasing.
Answer: 

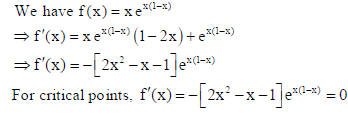
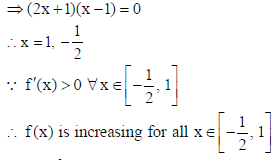
23. Find : 

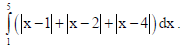
Answer:

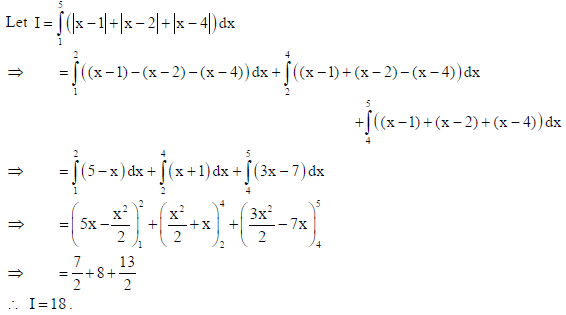
OR

Evaluate :  

Answer:  

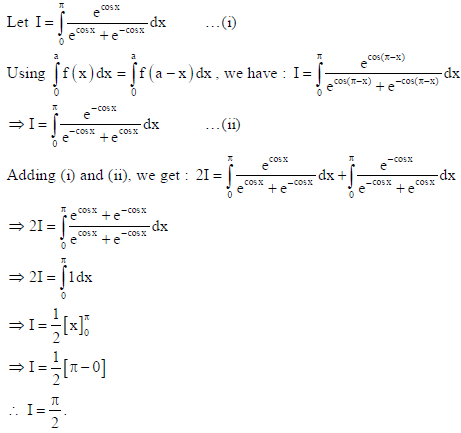
24. Find the area bounded by x² = 4y , x = 4y − 2 and y = 0 .
Answer: x² = 4y , x = 4y − 2 and, y = 0 .
On solving these equations, we get
x² = x + 2
⇒ x² − x − 2 = 0
⇒ (x +1)(x − 2) = 0
∴x = −1, 2 

25. Solve the differential equation dy/dx+1 e^x+y.
Answer: Given dy/dx + 1 e^x+y
Put x + y = t ⇒ dy/dx + 1 = dt/dx
∴ dt/dx = e^t
⇒ ∫e^−tdt = ∫dx
⇒ −e^−t = x + C
⇒ k − x = e^−t , where k = −C
∴(k − x)e^x+y =1 is the required solution.

26. If r̅ = xî + yĵ+ zk̂ , find (r̅ ×î).(r̅ ×ĵ) + xy.  
Answer: 

27. Find the coordinates of the point where the line through the points A(3, 4, 1) and B(5, 1, 6)
crosses the XY-plane.
Answer: Equation of XY-plane is z = 0    …(i)
Equation of line passing through the points A(3, 4, 1) and B(5, 1, 6) is :

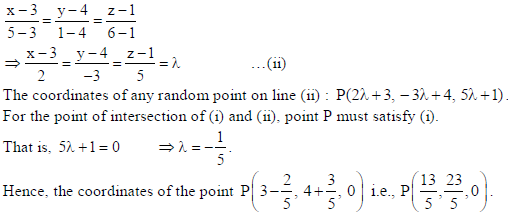
OR

Find the equation of plane containing the following lines :

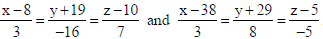
Answer: 

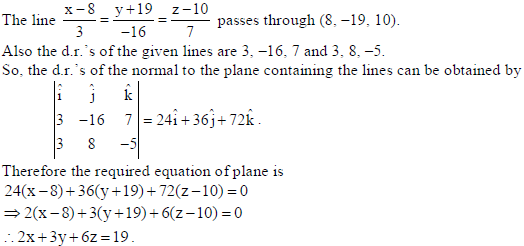
28. The probability distribution of a random variable X, where k is a constant, is given below : 

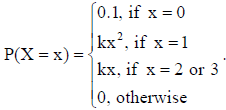
Determine
(a) the value of k
(b) P(X ≤ 2) .
Answer: (a) As ∑ P(X) =1
∴P(0) + P(1) + P(2) + P(3) + P(4) +… = 1
⇒ 0.1+ k(1)² + k(2) + k(3) + 0 +… =1
⇒ 6k = 0.9
⇒ k = 3/20.
(b) P(X ≤ 2) = P(0) + P(1) + P(2)
     P(X ≤ 2) = 0.1 + k + 2k = 1/10 + 3 x 3/20
∴ P(X≤2) = 11/20

Section IV

Questions in this section carry 3 marks each.

29. Show that the function f :R →{x ∈ R : −1< x <1} defined by f (x) x = x/1+|x| x ∈ R  is one and onto function.
Answer: 

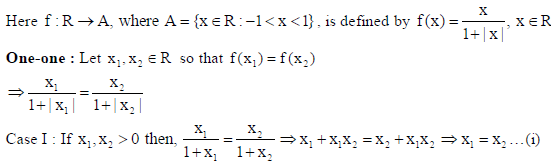
That is, for all f-image in the Codomain A, we’ve a pre-image in the Domain R of the function f.
So, f is onto function.

30. 

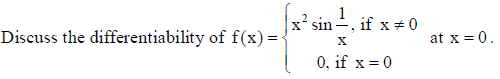
Answer: 

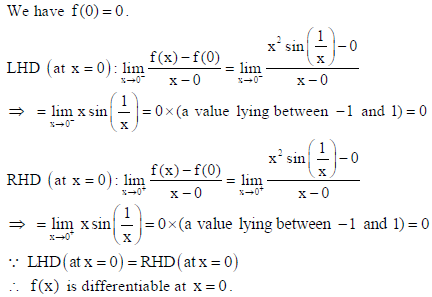
31. If x = e^cos2t and y = e^{sin 2t} , prove that dy/dx =  y log x/x log y.
Answer: 

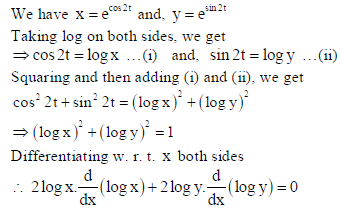
OR

If x^myⁿ = (x + y)^m+n , then prove that
(i) dy/dx = y/x and
(ii) d²y/dx² = 0.
Answer:  

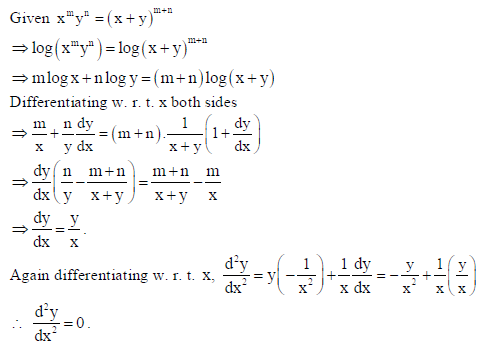
32. Show that the equation of normal at any point t on the curve x = 3cos t − cos³ t and y = 3sin t −sin³ t is 4(ycos³ t − x sin³ t) = 3sin 4t .
Answer: 

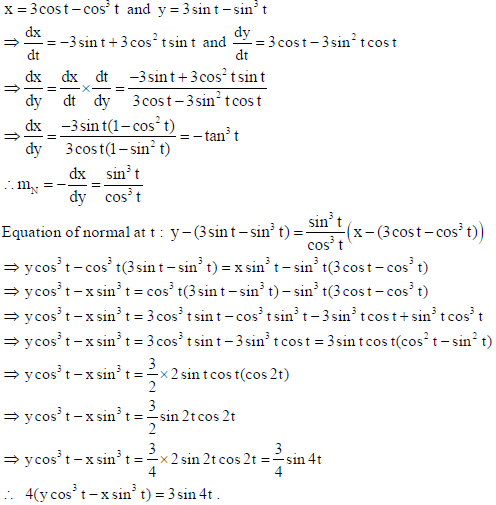
33. Find

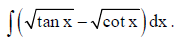
Answer: 

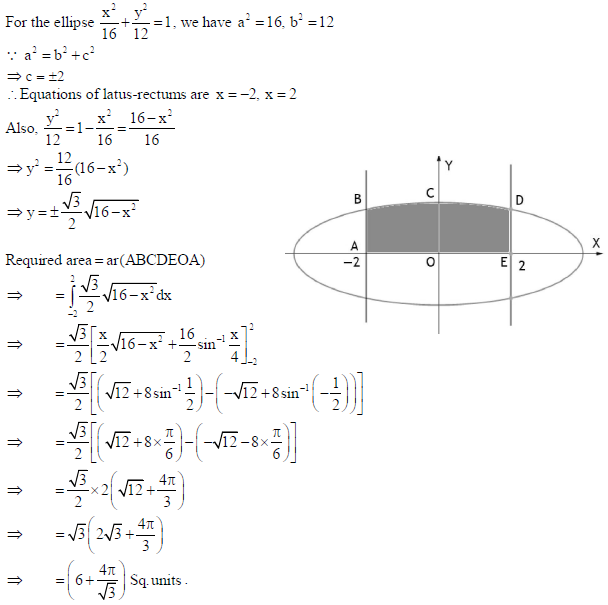
34. Using integration, find the area above x-axis, which is bounded by x²/16 + y²/21 = 1 , y = 0 and the ordinates represented by both the latus-rectums of the given ellipse. 
Answer: 

35. Solve : y + d/dx (xy) =  x(sin x + log x).
Answer: 

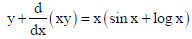
OR

If y(x) is a solution of (2 + sin x/1+y) dy/dx = −cos x and y(0) = 1, then find the value of y (π/2).
Answer: 

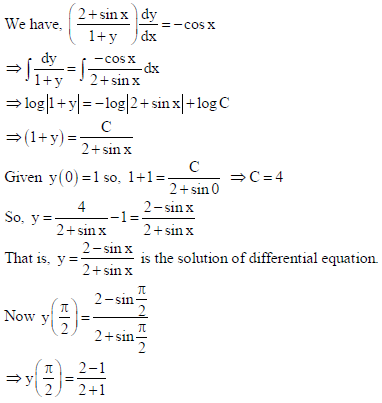
Section V

Questions in this section carry 5 marks each.

36. 

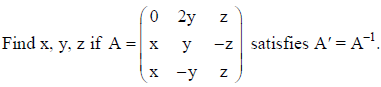
Answer: 

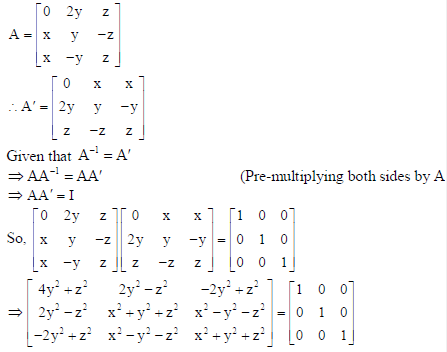
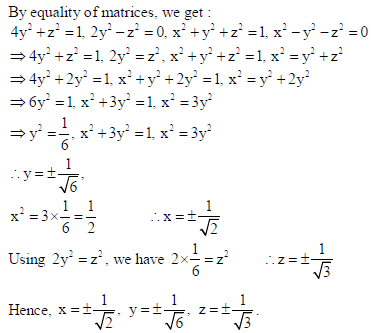
OR

Using matrices, solve the following system of equations :
3x + 2y + z = 10,
4x + y + 3z = 15,
x + y + z = 6.
Answer: 

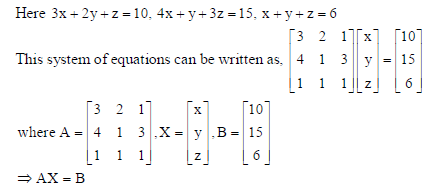
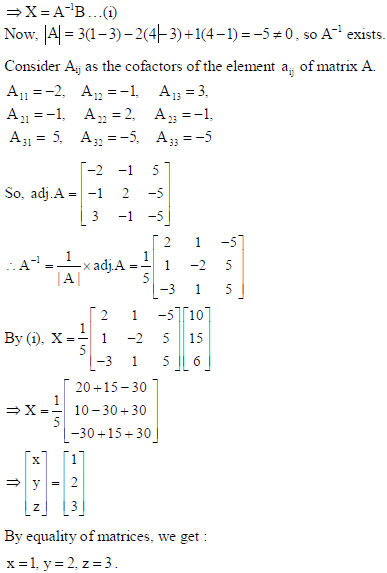
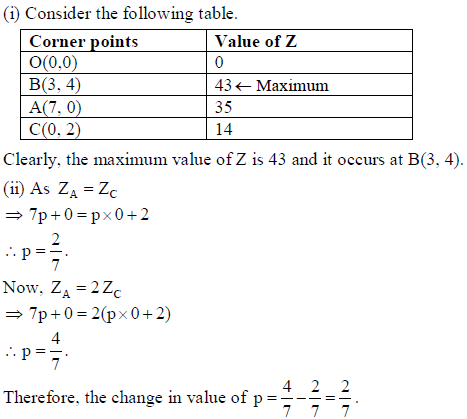
37. The corner points of the feasible region determined by the system of linear constraints are as shown below : 

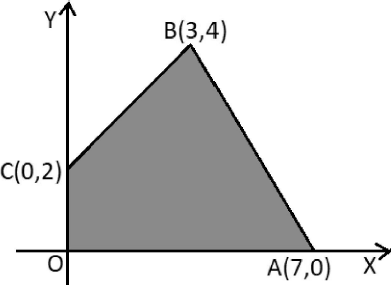
Answer each of the following :
(i) Let Z = 5x + 7y be the objective function. Find the maximum value of Z and, also the
corresponding point at which the maximum value occurs.
(ii) Let A C Z = px + y and Z = Z then, determine the value of p.
Also, what will be the change in the value of p, if A C Z = px + y and Z = 2Z ?
Answer: 

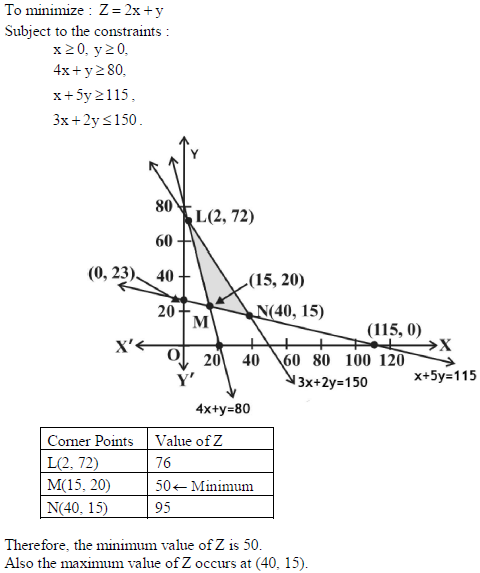
OR

Use graphical method to solve the following linear programming :
To minimize : Z = 2x + y
Subject to the constraints :
x ≥ 0,
y ≥ 0,
4x + y ≥ 80,
x + 5y ≥115 ,
3x + 2y ≤150 .
Also write the point at which maximum value of Z occurs.
Answer: 

38. A variable plane which remains at a constant distance 3p from the origin, cuts the coordinate
axes at A, B and C respectively. Find the locus of the centroid of triangle ABC.
Answer: 

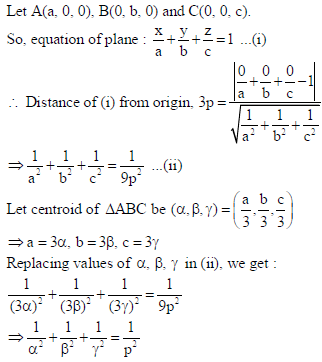
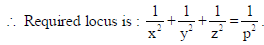
OR

Find the distance of the point 3î − 2ĵ+ k̂ from the plane 3x + y − z + 2 = 0 measured parallel to
the x−1/2 = y+2/−3 = z−1/1 . Also find the foot of perpendicular from the given point upon the given
plane.
Answer: