MCQ Question for Class 12 Mathematics Chapter 1 Relations and Functions Mathematics

Check the below NCERT MCQ Class 12 Mathematics Chapter 1 Relations and Functions Mathematics with Answers available with PDF free download. MCQ Questions for Class 12 Mathematics with Answers were prepared based on the latest syllabus and examination pattern issued by CBSE, NCERT and KVS. Our teachers have provided below Relations and Functions Mathematics Class 12 Mathematics MCQs Questions with answers which will help students to revise and get more marks in exams

Relations and Functions Class 12 Mathematics MCQ Questions with Answers

Refer below for MCQ Class 12 Mathematics Chapter 1 Relations and Functions Mathematics with solutions. Solve questions and compare with the answers provided below

Question. The maximum number of equivalence relations on the set A = {2, 3, 4} are
(a) 1
(b) 27
(c) 3
(d) 5

Question. If a relation R on the set {1, 2, 3} be defined by R = {(1, 2)}, then R is
(a) reflexive
(b) transitive
(c) symmetric
(d) none of these

Question. If the set A contains 7 elements and the set B contains 8 elements, then number of one-one and onto mappings from A to B is
(a) 24
(b) 120
(c) 0
(d) none of these

Question. Let A = {1, 2, 3, 4}. Let R be the equivalence relation on A × A defined by (a, b) R (c, d) if a + d = b + c. Then the equivalence class [(1, 3)] is
(a) {(1, 3)}
(b) {(2, 4)}
(c) {(1, 8), (2, 4), (1, 4)}
(d) {(1, 3) (2, 4)}

Question. If f : R → R be defined by f(x) = 2/x, x ∀ R, then f is
(a) one-one
(b) onto
(c) bijective
(d) f is not defined

Question. Which of the following functions form Z into Z bijections?
(a) f (x) = x³ (b) f
(x) = x + 2
(c) f (x) = 2x + 1
(d) f (x) = x² + 1

Question. If f : R → R be the function defined by f (x) = x³ + 5, then f^–1(x) is
(a) (x + 5)^1/3
(b) (x – 5)^1/3
(c) (5 – x)^1/3
(d) (5 – x)

Question. If f : A → B and g : B → C be the bijective functions, then (gof)^–1 is
(a) f^–1og^–1
(b) fog
(c) g^–1of^–1
(d) gof

Question. If f : R – {3/5} → R be defined by f (x) = 3x + 2 / 3x – 3 then
(a) f^–1(x) = f (x)
(b) f^–1(x) = – f (x)
(c) fof (x) = – x
(d) f^–1(x) = 1/19 f (x)

Question. Let f : N → R be the function defined by f (x) = 2x – 1/2 and g : Q → R be another function defined by g (x) = x + 2. Then (gof) 3/2 is
(a) 1
(b) – 1
(c) 7/2
(d) 3

Question. The function f : R → R defined by f(x) = x² + x is.
(a) one-one
(b) onto
(c) many-one
(d) None of the above

Question. Range of the function f(x) = x²+x+2/x²+x+1
(a) (1, ∞ )
(b) (1,11/7]
(c) (1, 7/3]
(d) (1, 7/5]

Question. The binary operation * defined on N by a * b = a + b + ab for all a, b ∈ N is
(a) commutative only
(b) associative only
(c) both commutative and associative
(d) None of these

Question. In the set N of natural numbers, define the binary operation * by m * n = GCD (m, n), m, n ∈ N. Then, which of the following is true?
I. * is not a binary operation
II. * is a binary operation
III. Inverse of each element of N exist
IV. Inverse of each element of N does not exist
(a) I and IV are true
(b) II and III are true
(c) Only I is true
(d) II and IV are true

Question. Consider the following statements I. For an arbitrary binary operation x on a set N, a x a = a ∀ a ∈ N.
II. If * is a commutative binary operation on N, then a x (b x c) = (c x b) x a.
(a) Only I is true
(b) Only II is true
(c) Both I and II are true
(d) Neither I nor II is true

Question. A function f : X → Y is said to be onto, if for every y ∈ Y, there exists an element x in X such that
(a) f(x) = y
(b) f(y) = x
(c) f(x) + y = 0
(d) f(y) + x = 0

Question. Which of the following functions from I to itself is a bijection?
(a) f(x) = x³
(b) f(x) = x + 2
(c) f (x) = 2x + 1
(d) f (x) = x² + x

Question. If f : R → R, f (x) = x³ + 2, then f –1 (x) is
(a) (x -1)^1/2
(b) x – 2
(c) (x – 2)^1/3
(d) (x – 2)1/2

Question. For real numbers x and y, we write x R y ⇔ x – y + √2 is an irrational number. Then, the relation R is
(a) Reflexive
(b) Symmetric
(c) Transitive
(d) None of these

Question. Let f : R → R be defined by

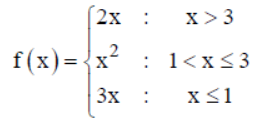
Then f (– 1) + f (2) + f (4) is
(a) 9
(b) 14
(c) 5
(d) None of these

Question. Let R be a relation on the set N be defined by {(x, y) l x, y ∈ N, 2x + y = 41}. Then, R is
(a) Reflexive
(b) Symmetric
(c) Transitive
(d) None of these

Question. The function f : R → R defined by f (x) = (x – 1) (x – 2) (x – 3) is
(a) one-one but not onto
(b) onto but not one-one
(c) both one-one and onto
(d) neither one-one nor onto

Question. Let f : (2, 3) → (0, 1) be defined by f(x) = x – [x]. Then, f–1(x) equals to
(a) x – 2
(b) x + 1
(c) x – 1
(d) x + 2

Question. Let f : N → R be the function defined by f(x) = 2x-1/2 and g : Q → R be another function defined by g(x) = x + 2. Then (gof) 3/2 is
(a) 1
(b) 0
(c) 7/2
(d) 3

Question. Consider the following statements on a set A = {1, 2, 3} I. R = {(1, 1), (2, 2)} is reflexive relation on A
II. R = {(3, 3)} is symmetric and transitive but not a reflexive relation on A
Which of the statements given above is/are correct ?
(a) Only I
(b) Only II
(c) Both I and II
(d) Neither I nor II

Question. Consider the following statements
Statement – I : An onto function f : {1, 2, 3} → {1, 2, 3} is always one-one.
Statement – II : A one-one function f :{1, 2, 3} → {1, 2, 3} must be onto.
(a) Only I is true
(b) Only II is true
(c) Both I and II are true
(d) Neither I nor II is true

Question. Let function f : R → R be defined by f (x) = 2x + sin x for x ∈ R , then f is
(a) one-one and onto
(b) one-one but NOT onto
(c) onto but NOT one-one
(d) neither one-one nor onto

Question. Let f : R → R be given by f(x) = tan x. Then f –1(1) is
(a) π/4
(b) {nπ + π/4 : n ∈ Z}
(c) does not exist
(d) None of these

Question. Let S be a finite set containing n elements. Then the total number of binary operations on S is:
(a) n²ⁿ
(b) nⁿ
(c) 2n²
(d) n²

Question. The function f : R → R defined by f (x) = sin x is :
(a) into
(b) onto
(c) one-one
(d) many one

Question. The domain of y = x/√|x|-x is
(a) [0, ∞)
(b) (– ∞, 0)
(c) (– ∞, 0]
(d) [1, ∞)

Question. Let X = {– 1, 0, 1}, Y = {0, 2} and a function f : X → Y defined by y = 2x⁴, is
(a) one-one onto
(b) one-one into
(c) many-one onto
(d) many-one into

Question. A binary operation x on the set {0, 1, 2, 3, 4, 5} is defined as

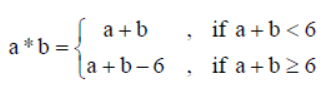
the identity element is
(a) 0
(b) 1
(c) 2
(d) 3

Question. The mapping f : N → N given by f(n) = 1 + n², n ∈ N when N is the set of natural numbers, is
(a) one-one and onto
(b) onto but not one-one
(c) one-one but not onto
(d) neither one-one nor onto

Question. Let f : R → R be a function defined by f(x) = x³ + 4, then f is
(a) injective
(b) surjective
(c) bijective
(d) None of these

Assertion Reason Type Questions :

(a) Assertion is correct, reason is correct; reason is a correct explanation for assertion.
(b) Assertion is correct, reason is correct; reason is not a correct explanation for assertion
(c) Assertion is correct, reason is incorrect
(d) Assertion is incorrect, reason is correct.

Question. Assertion : If f is even function, g is odd function, then f/g , (g ≠ 0) is an odd function .
Reason : If f(–x) = –f(x) for every x of its domain, then f(x) is called an odd function and if f(–x) = f(x) for every x of its domain, then f(x) is called an even function.

Question. Assertion : The binary operation * : R × R → R given by a * b → a + 2b is associative.
Reason : A binary operation*: A × A → A is said to be associative, if (a * b) * c = a * (b * c) for all a, b, c ∈ A.

Question. Assertion : If the relation R defined in A = {1, 2, 3} by aRb, if |a² – b²| ≤ 5, then R– 1 = R
Reason : For above relation, domain of R–1 = Range of R.

Question. Assertion : Let A = {–1, 1, 2, 3} and B = {1, 4, 9}, where f : A → B given by f(x) = x², then f is a many-one function.
Reason : If x₁ ≠ x₂ ⇒ f(x₁) ≠ f(x₂), for every x₁, x₂ ∈ domain, then f is one-one or else many-one.

Question. Assertion : f : R → R is a function defined by f(x) = 2x+1/3 . Then f–1(x) = 3x-1/2 .
Reason : f(x) is not a bijection.

Case Based Questions

A relation R on a set A is said to be an equivalence relation on A if it is
• Reflexive i.e., (a, a) ∈ R V a ∈ A.
• Symmetric i.e., (a, b) ∈ R ⇒ (b, a) ∈ R V a, b ∈ A.
• Transitive i.e., (a, b) ∈ R and (b, c) ∈ R
⇒ (a, c) ∈ R ∀ a, b, c ∈A.
Based on the above information, answer the following questions:

Question. If the relation R = {(1, 1), (1, 2), (1, 3), (2, 2),
(2, 3), (3, 1), (3, 2), (3, 3)} defined on the set A = {1, 2, 3}, then R is
(a) reflexive
(b) symmetric
(c) transitive
(d) equivalence

Question. If the relation R = {(1, 2), (2, 1), (1, 3), (3, 1)}
defined on the set A = {1, 2, 3}, then R is
(a) reflexive
(b) symmetric
(c) transitive
(d) equivalence

Question. If the relation R on the set N of all natural numbers defined as R = {(x, y) : y = x + 5 and (x < 4), then R is
(a) reflexive
(b) symmetric
(c) transitive
(d) equivalence

Question. If the relation R on the set A = {1, 2, 3, … 13, 14} defined as R = {(x, y) : 3x – y = 0}, then R is
(a) reflexive
(b) symmetric
(c) transitive
(d) equivalence

Question. If the relation R on the set A = {1, 2, 3} defined as R = {(1, 1), (1, 2), (1, 3), (2, 1), (2, 2), (2, 3), (3, 1), (3, 2), (3, 3)}, then R is
(a) reflexive only
(b) symmetric only
(c) transitive only
(d) equivalence

Consider the mapping f : A → B is defined by f(x) = x – 1/X -2 such that f is a bijection.
Based on the above information, answer the following questions:

Question. Domain of f is
(a) R – {2} (b) R
(c) R – {1, 2}
(d) R – {0}

Question. Range of f is
(a) R
(b) R – {1}
(c) R – {0}
(d) R – {1, 2}

Question. If g : R – {2} → R – {1} is defined by g(x) = 2f(x) – 1, then g(x) in terms of x is

Question. The function g defined above, is
(a) One-one
(b) Many-one
(c) into
(d) None of these

Question. A function f(x) is said to be one-one if
(a) f(x₁) = f(x₂) ⇒ –x₁ = x₂
(b) f(–x₁) = f(–x₂) ⇒ –x₁ = x₂
(c) f(x₁) = f(x₂) ⇒ x₁ = x₂
(d) None of these

A general election of Lok Sabha is a gigantic exercise. About 911 million people were eligible to vote and voter turnout was about 67%, the highest ever

Let I be the set of all citizens of India who were eligible to exercise their voting right in general election held in 2019. A relation ‘R’ is defined on I as follows:
R = {(V₁, V₂)} : V₁, V₂ ∈ I and both use their voting right in general election — 2019}
Based on the above information answer the following:

Question. Two neighbours X and Y ∈ I. X exercised his voting right while Y did not cast her vote in general election — 2019. Which of the following is true?
(a) (X, Y) ∈ R
(b) (Y, X) ∈ R
(c) (X, X) ∉ R
(d) (X, Y) ∉ R

Question. Mr. ‘X’ and his wife ‘W’ both exercised their voting right in general election — 2019, which of the following is true?
(a) Both (X, W) and (W, X) ∈ R
(b) (X, W) ∈ R but (W, X) ∉ R
(c) Both (X, W) and (W, X) ∉ R
(d) (W, X) ∈ R but (X, W) ∉ R

Question. Three friends F₁, F₂ and F₃ exercised their voting right in general election — 2019, then which of the following is true?
(a) (F₁, F₂) ∈ R, (F₂, F₃) ∈ R and (F₁, F₃) ∈ R
(b) (F₁, F₂) ∈ R, (F₂, F₃) ∈ R and (F₁, F₃) ∉ R
(c) (F₁, F₂) ∈ R, (F₂, F₃) ∈ R but (F₁, F₃) ∉ R
(d) (F₁, F₂) ∉ R, (F₂, F₃) ∉ R and (F₁, F₃) ∉ R

Question. The above defined relation R is …………. .
(a) Symmetric and transitive but not reflexive
(b) Universal relation
(c) Equivalence relation
(d) Reflexive but not symmetric and transitive

Question. Mr. Shyam exercised his voting right in General Election — 2019, then Mr. Shyam is related to which of the following?
(a) All those eligible voters who cast their votes
(b) Family members of Mr. Shyam
(c) All citizens of India
(d) Eligible voters of India

Sherlin and Danju are playing Ludo at home during Covid-19. While rolling the dice, Sherlin’s sister Raji observed and noted the possible outcomes of the throw every time belongs to set {1, 2, 3, 4, 5, 6}. Let A be the set of players while B be set of all possible outcomes.
A = {S, D}, B = {1, 2, 3, 4, 5, 6

Based on the above information answer the following:

Question. Let R : B → B be defined by R = {(x, y) : y is divisible by x} is
(a) Reflexive and transitive but not symmetric
(b) Reflexive and symmetric but not transitive
(c) Not reflexive but symmetric and transitive
(d) Equivalence

Question. Raji wants to know the number of functions from A to B. How many number of functions are possible?
(a) 62
(b) 26
(c) 6!
(d) 212

Question. Let R be a relation on B defined by R = {(1, 2), (2, 2), (1, 3), (3, 4), (3, 1), (4, 3), (5, 5)}. Then R is
(a) Symmetric
(b) Reflexive
(c) Transitive
(d) None of these three

Question. Raji wants to know the number of relations possible from A to B. How many numbers of relations are possible?
(a) 62
(b) 26
(c) 6!
(d) 212

Question. Let R : B → B be defined by R = {(1, 1), (1, 2), (2, 2)(3, 3), (4, 4), (5, 5), (6, 6)}, then R is
(a) Symmetric
(b) Reflexive and Transitive
(c) Transitive and symmetric
(d) Equivalence

An organization conducted bike race under 2 different categories—boys and girls. Totally there was 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for this college project.
Let B = {b₁, b₂, b₃} and G = {g₁, g₂} where B
represents the set of boys selected and G the set of girls who were selected for the final race.

types of relations and functions.
Based on the above information answer the following:

Question. Ravi wishes to form all the relations possible from B to G. How many such relations are possible?
(a) 2⁶
(b) 2⁵
(c) 0
(d) 2³

Question. Let R : B → B be defined by R = {(x, y) : x and y are students of same sex}, then this relation R is …………. .
(a) Equivalence
(b) Reflexive only
(c) Reflexive and symmetric but not transitive
(d) Reflexive and transitive but not symmetric

Question. Ravi wants to know among those relations, how many functions can be formed from B to G?
(a) 2²
(b) 2¹²
(c) 3²
(d) 2³

Question. Let R : B → G be defined by R = {(b₁, g₁), (b₂, g₂), (b₃, g₁)}, then R is ______ .
(a) Injective
(b) Surjective
(c) Neither Surjective nor Injective
(d) Surjective and Injective

Question. Ravi wants to find the number of injective functions from B to G. How many numbers of injective functions are possible?
(a) 0
(b) 2!
(c) 3!
(d) 0!

Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x – 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

Answer the following using the above information:

Question. Let relation R be defined by R = {(L₁, L₂) : L₁ || L₂ where L₁, L₂ ∈ L}, then R is ____ relation.
(a) Equivalence (b) Only reflexive
(c) Not reflexive
(d) Symmetric but not transitive

Question. Let R = {(L₁, L₂) : L₁ ⊥ L₂ where L₁, L₂ ∈ L} which of the following is true?
(a) R is Symmetric but neither reflexive nor transitive.
(b) R is Reflexive and transitive but not symmetric
(c) R is Reflexive but neither symmetric nor transitive.
(d) R is an Equivalence relation.

Question. The function f : R → R defined by f(x) = x – 4 is _______ .
(a) Bijective
(b) Surjective but not injective
(c) Injective but not Surjective
(d) Neither Surjective nor Injective

Question. Let f : R → R be defined by f(x) = x – 4. Then the range of f(x) is ______ .
(a) R
(b) Z
(c) W
(d) Q

Question. Let R = {(L₁, L₂) : L₁ is parallel to L₂ and L₁ : y = x – 4} then which of the following can be taken as L₂?
(a) 2x – 2y + 5 = 0
(b) 2x + y = 5
(c) 2x + 2y + 7 = 0
(d) x + y = 7

Raji visited the Exhibition along with her family.
The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x².

Answer the following questions using the above information:

Question. Let f : R → R be defined by f(x) = x² is ____
(a) Neither Surjective nor Injective
(b) Surjective
(c) Injective
(d) Bijective

Question. Let f : N → N be defined by f(x) = x² is _____
(a) Surjective but not Injective
(b) Surjective
(c) Injective
(d) Bijective

Question. Let f: {1, 2, 3,…} → {1, 4, 9, …} be defined by f(x) = x² is _______ .
(a) Bijective
(b) Surjective but not injective
(c) Injective but Surjective
(d) Neither Surjective nor Injective

Question. Let : N → R be defined by f(x) = x². Range of the function among the following is ______
(a) {1, 4, 9, 16, …}
(b) {1, 4, 8, 9, 10,…}
(c) {1, 4, 9, 15, 16,…}
(d) {1, 4, 8, 16,…}

Question. The function f : Z → Z defined by f(x) = x² is ________
(a) Neither Injective nor Surjective
(b) Injective
(c) Surjective
(d) Bijective