Measures of Statistical Data MCQ Questions

Please go through Measures of Statistical Data MCQ with Answers for Environment Economics provided below. Students should have strong knowledge about the Measures of Statistical Data as in various competition exams, MCQ questions are asked from this topic. We have provided below the biggest collection of Measures of Statistical Data MCQ with Answers. These Business Statistics MCQ and objective questions will improve your performance in exams and help you to get good scores.

Multiple Choice Questions for Measures of Statistical Data with Answers

Question. The mean deviation from the median is:
(a) Equal to that measured from another value
(b) Maximum if all the observations are positive
(c) Greater than that measured from any other value
(d) Less than that measured from any value

Question. The mean deviation of the series a, a + d, a +2d……., a + 2n from its mean is
(a) (n + 1) d /2n +1
(b) nd /2n +1
(c) n (n +1) d /2n +1
(d) (2n +1) d /n (n+1)

Question. A,B, C are three sets of values of x:
A: 2, 3, 7, 1, 3, 2, 3
B: 7, 5, 9, 12, 5, 3, 8
C: 4, 4, 11, 7, 2, 3, 4
Which is true:
(a) Mean of A = Mode of C
(b) Mean of C = Median of B
(c) Median of B = Mode of A
(d) Mean, median, mode of A are equal

Question. The mean and variance of 7 observations are 8 and 16 . If 5 of the observations are 2, 4, 10, 12, 14 the remaining 2 observations are:
(a) x =6 , y = 8
(b) x=5, y=7
(c) x=7 , y=3
(d) None of these

Question. The mean deviation about median from the data: 340, 150, 210, 240, 300, 310, 320 is:
(a) 51.6
(b) 51.8
(c) 52
(d) 52.8

Question. For a frequency distribution mean deviation from mean is computed by
(a) ∑E f /∑ E f |d|
(b) ∑E d /∑Ef
(c) ∑E fd/ ∑E f
(d) ∑Ef | d | / ∑E f

Question. A batsman score runs in 10 innings as 38, 70, 48, 34, 42, 55, 63, 46, 54 and 44. The mean deviation about mean is
(a) 8.6
(b) 6.4
(c) 10.6
(d) 7.6

Question. The arithmetic mean height of 50 students of a college is 5’—8’. The height of 30 of these is given in the frequency distribution. Find the arithmetic mean height of the remaining 20 students.

(a) 5’ —-8.8”
(b) 5’ —- 8.0”
(c) 5’—– 7.8”
(d) 5’—– 7.0”

Question. The median of the data: 19, 25, 59, 48, 35, 31, 30, 32, 51. If 25 is replaced by 52, what will be the new median.
(a) 35
(b) 53
(c) 43
(d) 45

Question. If the median of the following frequency distribution is 46, find the missing frequencies.

(a) a = 32 b =40
(b) a =31 b = 45
(c) a = 33 b = 42
(d) a =34 b =45

Question. The standard deviation for 15, 22, 27, 11, 9, 21, 14, 9 is:
(a) 6.22
(b) 6.12
(c) 6.04
(d) 6.32

Question. A student obtained the mean and the standard deviation of 100 observations as 40 and 5.1. It was later found that one observation was wrongly copied as 50, the correct figure being 40. Find the correct mean and the S.D.
(a) Mean = 38.8, S.D =5
(b) Mean = 39.9, S.D =5
(c) Mean = 39.9, S.D = 4
(d) None

Question. Find the value of x, if the mode of the data is 25: 15, 20, 25, 18, 14, 15, 25, 15, 18, 16, 20, 25, 20, x,
(a) 15
(b) 18
(c) 25
(d) 20

Question. For the following grouped frequency distribution find the mode:

(a) 13.9
(b) 14.7
(c) 15.1
(d) 14.6

Question. Find the value of third quartile if the values of first quartile and quartile deviation are 104 and 108 respectively.
(a) 130
(b) 140
(c) 120
(d) 110

Question. Age distribution of 200 employees of a firm is given below and calculate semi inter quartile range = (Q3 – Q1 ) /2 of the distribution:

(a) 4.75 years
(b) 4.25 years
(c) 4 years
(d) None of these

Question. The table shows the age distribution of cases of a certain disease admitted during a year in a particular hospital.

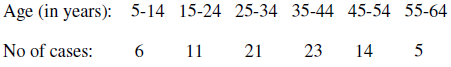
The average age for which maximum cases occurred is:
(a) 34.33
(b) 35.34
(c) 36.31
(d) 37.31

Question. Mean of 10 items is 50 and S.D is 14. Find the sum of squares of all items
(a) 26543
(b) 26960
(c) 27814
(d) 27453

Question. Find the range for the following data
14, 16, 16, 14, 22, 13, 15, 24, 12, 23, 14, 20, 17, 21, 22, 18, 18, 19, 20, 17, 16, 15, 11, 12, 21, 20, 17, 18, 19, 23.
(a) 13
(b) 12
(c) 14
(d) 16

Question. In a moderately symmetric distribution mean, median and mode are connected by:
(a) Mode = 2 median – 3 mean
(b) Mode = 3 median – 4 mean
(c) Mode = 3 median – 2 mean
(d) Mode = 2 median – 4 mean

Question. The algebraic sum of the deviations of a set of n values from their mean is
(a) 0
(b) n – 1
(c) n
(d) n + 1

Question. The variance of 15 observations is 4. If each observation is increased by 9, the variance of the resulting observation is:
(a) 2
(b) 3
(c) 4
(d) 5

Question. The mean of 5 observations is 4.4 and their variance is 8.24. If 3 of the observations are 1, 2, 6. The other 2 observations are:
(a) 9, 4
(b) 7, 8
(c) 6, 5
(d) 4, 8

Question. Initially there were 9 workers, all being paid a uniform wage. Later a 10th worker is added to the list whose wage rate is Rs. 20 less than for others. The standard deviations of wages for the group of 10 workers are:
(a) 5
(b) 4
(c) 7
(d) 6

Question. Twenty passengers were found ticketless on a bus. The sum of squares and the standard deviation of the amount found in their pockets were Rs.2,000 and Rs.6. If the total fine imposed on these passengers is equal to the total amount recovered from them and fine imposed is uniform, what is the amount each one has to pay as fine?
(a) 5
(b) 6
(c) 8
(d) 9

Question. The geometric mean of 10 observation s on a certain variable was calculated as 16.2. It was later discovered that one of the observations was wrongly recorded as 12.9; in fact it was 21.9. The correct G.M is:
(a) 17.12
(b) 18.43
(c) 17.08
(d) 18.15

Question. Find the quartile deviation for the data:

(a) 18.625
(b) 19.925
(c) 17.485
(d) None of these

Question. From the monthly income of 10 families find the coefficient of range is:

(a) 0.1
(b) 0.6
(c) 0.84
(d) 0.56

Question. Find the lower quartile for the distribution

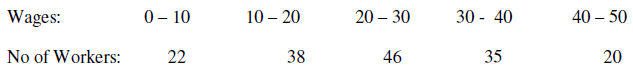
(a) 13.80
(b) 12.56
(c) 14.803
(d) None of the above

Question. The mean of n observations is X. If k is added to each observation then the new mean is
(a) X
(b) X + k
(c) X –k
(d) kX

Question. The mean of n observations is X. If each observation is multiplied by k, the mean of new observation is:
(a) kX
(b) X /k
(c) X +k
(d) X – k

Question. Find the Mean deviation from the Mean for the following

(a) 14
(b) 12
(c) 15
(d) 16

Question. Compute the modal value for

(a) 175
(b) 125
(c) 145
(d) 165

Question. Compute the mode for the following frequency distribution:

(a) 32.66
(b) 28.43
(c) 24.87
(d) 31.65

Question. Mean deviation which is calculated is minimum at:
(a) Mean
(b) Median
(c) Mode
(d) All the three

Question. For any discrete distribution standard deviation is not less than
(a) Mean deviation from mean
(b) Mean deviation from median
(c) Mode
(d) None of these

Question. Find the sum of the deviation of the variable values 3, 4, 6, 8, 14 from their mean
(a) 5
(b) 0
(c) 1
(d) 7

Question. The median of the observation 11, 12, 14, 18, x + 4, 30, 32, 35, 41 arranged in ascending order is 24, then x is
(a) 21
(b) 22
(c) 23
(d) 24

Question. Three groups of observations contain 8, 7 and 5 observations. Their geometric means are 8.52, 10.12 and 7.75. Find the geometric mean of the 20 observations in the single group formed by pooling the three groups is:
(a) 7.831
(b) 8.837
(c) 9.643
(d) 6.438

Question. Find the Quartile deviation for the distribution:

(a) 15.44
(b) 16.22
(c) 14.55
(d) 17.33