Students can read the important questions given below for Probability Class 9 Mathematics . All Probability Class 9 Notes and questions with solutions have been prepared based on the latest syllabus and examination guidelines issued by CBSE, NCERT and KVS. You should read all notes provided by us and Class 9 Mathematics Important Questions provided for all chapters to get better marks in examinations. Mathematics Question Bank Class 9 is available on our website for free download in PDF.
Important Questions of Probability Class 9
Very Short Answer Type Questions:
Question. In a single throw of two dice, find the probability that there will be a doublet.
Ans. Number of elements in sample space when two dice are thrown = 6 × 6 = 36
Doublets are {(1, 1), (2, 2), (3, 3), (4, 4), (5, 5), (6, 6)}
i.e., 6 in number
∴ P(getting doublet)
= Number of doublets/Number of elements in sample space
= 6/36 = 1/6
Question. The following table shows the birth months of 48 babies in a hospital:


Find the probability of months in which 6 babies were born.
Ans. Number of months = 12 ⇒ n(S) = 12
Let E be the event having months in which 6 babies were born i.e., July, Aug and Dec
⇒ n(E) = 3
∴ P E = n(E)/n(S) = 3/12 = 1/4
Question. A survey of 100 children of a locality shows their favourite sport

Out of these children, one is chosen at random.
What is the probability that the chosen child likes football?
Ans. Total number of students, n(S) = 100
Let E be the event that child likes football.
i.e., n(E) = 48
∴ P(E) = 48/100 = 12/25
Question. Based on the given information, find the probability of people with age 60, 61 & 64 who can drive.

Ans. Number of people with age 60, 61 and 64 who can drive = 16090 + 11490 + 3607 = 31187
Total number of people who can drive
= 16090 + 11490 + 8012 + 5448 + 3607 + 2320 = 46967
∴ Required probability = 31187/46967
Question. The following data represents the number of girls in a family.

A family is chosen at random. Find the probability of having exactly 2 girls in the chosen family.
Ans. Total number of families = 1000 = n(S)
Number of families having exactly 2 girls = 475 = n(E)
∴ P E = n(E)/n(S) = 475/1000 = 19/40
Short Answer Type Questions:
Question. The number of hours spent by Ashu, a school student, on various activities on a working day
are given below:

His friend Sonu came to his house to meet Ashu.
What is the probability that
(i) Ashu is available at home.
(ii) Ashu will play outdoor games.
Ans. Total number of hours = 24
(i) Number of hours during which Ashu is at home
= 7 + 2 + 2 = 11
∴ Probability that Ashu is available at home = 11/24
(ii) Number of hours during which Ashu plays outdoor games = 3
∴ Probability that Ashu will play outdoor games = 3/24 = 1/8
Question. An integer is chosen at random from the first 200 positive integers. Find the probability that the integer is divisible by 11.
Ans. Total number of integers in the sample space = 200 = n(S).
Among first 200 positive integers, we have 11, 22, 33, 44, 55, 66, 77, 88, 99, 110, 121, 132, 143, 154, 165, 176, 187, 198 are divisible by 11.
∴ Number of integers which are divisible by 11 = 18
P(integer is divisible by 11)
= Number of integers divisible by 11/Total number of integers in sample space
= 18/200 = 9/100
Question. In a locality of 5000 families a survey was conducted and the following data was collected.

Out of these families, a family is chosen at random. What is the probability that the chosen family has less than 5 members?
Ans. Total number of families, n(S) = 5000
Let E be the event that the chosen family has less than 5 members.
i.e., n(E) = 1060 + 1000 + 1020 = 3080
∴ P(E) = n(E)/n(S) = 3080/5000 = 77/125
Question. The percentage of attendance of different classes in a year in a school is given below:
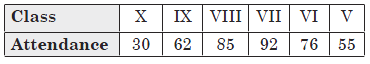
(i) What is the probability that the class attendance is more than 75%?
(ii) Find the probability that the class attendance is less than 50%.
Ans. Total number of classes = 6
(i) Number of classes in which attendance percentage is more than 75% = 3
∴ Required probability = 3/6=1/2
(ii) Number of classes in which attendance is less than 50% = 1
∴ Required probability = 1/6
Question. In 60 throws of a die, the outcomes were noted as below:

If die is thrown at random, then what is the probability that upper face of a die shows an even prime number? Also find the probability that upper face shows an odd number.
Ans. Total number of throws, n(S) = 60
Let E be the event that upper face shows an even prime number, i.e.,
n(E) = 10 [ 2 is the only even prime number]
∴ P(E) = n(E)/n(S) = 100/60 = 1/6
Let F be the event that upper face shows an odd number
= 8 + 15 + 7 = 30
∴ P(F) = n(F)/n(S) = 30/60 = 1/2
Question. There are 35 students in class IX–A, 34 in IX-B and 33 in IX–C. Some of them are allotted project on Chapter 2 (Polynomials) and some on Chapter-1 (Number system) as shown in the table.

Find the probability that the student chosen at random,
(i) prepares project on chapter 1
(ii) prepares project on chapter 2
Ans. Total number of students = 35 + 34 + 33 = 102
(i) Number of students prepare project on chapter-1 = 74
∴ Probability that the student prepares project on
chapter-1 = 74/102 = 37/51
(ii) Number of students prepare project on chapter-2 = 28
∴ Probability that the student prepares project on
chapter-2 = 28/102 = 14/51
Question. A die was rolled 100 times and the number of times, 6 came up was noted. If the experimental probability calculated from this information is 2/5 , then how many times 6 came up?
Ans. Here, total number of trials = 100
Let x be the number of times 6 came up.
We know, probability of an event
= Frequency of the event occuring/Total number of trials
⇒ x/100 = 2/5
⇒ x = 40
Question. The probability of guessing the correct answer to a certain question is x/5 . If the probability of not
guessing the correct answer is 2x/3 . Then, find the value of 26x.
Ans. We have
P(guessing correct answer) = x/5 and P(not guessing correct answer) = 2x/3
Clearly, P(guessing correct answer) + P(not guessing correct answer) = 1 [ P(E) + P (not E) = 1]
∴ x/5 + 2x/3 = 1
⇒ 3x + 10x = 15 ⇒ x = 15/13 ⇒ 26x = 26 x 15/13 = 30
Question. A die is thrown 100 times and following observations were recorded:

Find the probability that the die shows
(i) a number less than 3.
(ii) a number greater than 4.
Ans. n(S) = 100
(i) Let E be the event that the die shows a number less than 3, i.e., n(E) = 12 + 18 = 30
∴ P(E) = n(E)/n(S) = 30/100 = 3/10
(ii) Let F be the event that the die shows a number greater than 4, i.e., n(F) = 14 + 16 = 30
∴ P(F) = n(F)/n(S) = 30/100 = 3/10
Question. If the difference between the probability of success and failure (i.e., not success) of an event
is 5/19 (assuming probability of failure is greater than that of success). Find the probability of success and failure of the event respectively.
Ans. Let the probability of success be x
Then, probability of failure = 1 – probability of success
⇒ Probability of failure = 1 – x
According to question, we have,
Probability of failure – Probability of success = 5/19
⇒ 1 − x − x = 5/19 ⇒ 1−2x = 5/19
⇒ 2x = 1 − 5/19 = 14/19 ⇒ x=7/19
∴ Probability of success = 7/19
and probability of failure = 1 − 7/19 = 12/19
Long Answer Type Questions:
Question. Sixty seeds were selected at random from each of 8 bags of seeds, and were kept under standardised conditions favourable to germination. After 1 month, the number of seeds which had germinated in each collection were counted and recorded as follows :
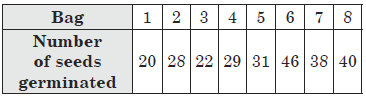
What is the probability of germination of
(i) more than 30 seeds in a bag ?
(ii) 50 seeds in a bag ?
(iii) less than 30 seeds in a bag ?
Ans. Total number of bags = 8
(i) Number of bags in which more than 30 seeds germinated = 4
∴ P(germination of more than 30 seeds in a bag) = 4/8 = 1/2
(ii) Number of bags in which 50 seeds germinated = 0
∴ P(germination of 50 seeds in a bag) = 0/8 = 0
(iii) Number of bags in which less than 30 seeds germinated = 4
∴ P(germination of less than 30 seeds in a bag) = 4/8 = 1/2
Question. Given below is the frequency distribution of daily wages (in Rs) of 30 workers in a certain factory.

A worker is selected at random. Find the probability that his wages is
(i) less than Rs 150
(ii) atleast Rs 210
(iii) more than or equal to Rs 150 but less than Rs 210
(iv) in the interval 190-250
Ans. The total number of workers = 30
(i) Number of workers whose wages are less than Rs 150
= 3 + 4 = 7
∴ P(that worker get wages less than Rs 150) = 7/30
(ii) Number of workers whose wages are atleast Rs 210
= 4 + 3 = 7
∴ P(that worker get wages atleast Rs 210) = 7/30
(iii) Number of workers whose wages are more than or equal to Rs 150 but less than Rs 210 = 5 + 6 + 5 = 16
∴ P(that worker get wages more than or equal to Rs 150 but less than Rs 210) = 16/30 = 8/15
(iv) Number of workers whose wages lie in the interval 190-250 = 5 + 4 + 3 = 12
∴ P(that worker get wages lie in the interval 190-250) = 12/30 = 2/5.



