Students can read the important questions given below for Quadrilaterals Class 9 Mathematics . All Quadrilaterals Class 9 Notes and questions with solutions have been prepared based on the latest syllabus and examination guidelines issued by CBSE, NCERT and KVS. You should read all notes provided by us and Class 9 Mathematics Important Questions provided for all chapters to get better marks in examinations. Mathematics Question Bank Class 9 is available on our website for free download in PDF.
Important Questions of Quadrilaterals Class 9
Very Short Answer Type Questions:
Question. Two consecutive angles of a parallelogram are (x + 60°) and (2x + 30°). What special name can you give to this parallelogram?
Ans. We know that consecutive interior angles of a parallelogram are supplementary.
∴ (x + 60°) + (2x + 30°) = 180°
∴ 3x + 90° = 180° ⇒ 3x = 90° ⇒ x = 30°
Thus, two consecutive angles are (30° + 60°), (2 × 30° + 30°) i.e., 90° and 90°.
Hence, the special name of the given parallelogram is rectangle.
Question. ABCD is a trapezium in which AB || DC and ∠A = ∠B = 45°. Find angles C and D of the trapezium.
Ans. We have given, a trapezium ABCD, whose parallel sides are AB and DC.
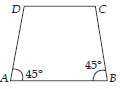
Since, AB || CD and AD is a transversal.
∴ ∠A + ∠D = 180° [Angles on same side of transversal]
⇒ ∠D = 180° – ∠A = 180° – 45° = 135°
Similarly, ∠C = 135°
Question. In the figure, it is given that QLMN and NLRM are parallelograms. Can you say that QL = LR? Why or why not?
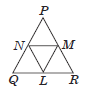
Ans. Yes, QL = LR
As, opposite sides of a parallelogram are equal.
∴ In parallelogram QLMN, QL = NM …(i)
In parallelogram NLRM, NM = LR …(ii)
From (i) and (ii), QL = LR
Question. In the given figure, PQRS is a parallelogram in which ∠PSR = 125°. Find the measure of ∠RQT.
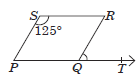
Ans. ∠PQR = ∠PSR = 125°
(∵Opposite angles of a parallelogram are equal)
Now, ∠PQR + ∠RQT = 180° (Linear pair)
⇒ 125° + ∠RQT = 180° ⇒ ∠RQT = 55°
Question. If one angle of a rhombus is a right angle, then it is necessarily a _______ .
Ans. If one angle of a rhombus is a right angle, then it is necessarily a square.
Short Answer Type Questions:
Question. PQRS is a rhombus with ∠QPS = 50°. Find ∠RQS.
Ans. Since a rhombus satisfies all the properties of a parallelogram.
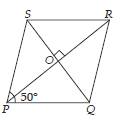
∴ ∠QPS = ∠QRS [Opposite angles of a parallelogram]
⇒ ∠QRS = 50° [∠QPS = 50° (Given)]
Diagonals of a rhombus bisect the opposite angles.
∴ ∠ORQ = 1/2 ∠QRS ⇒ ∠ORQ = 25°
Now, in ΔORQ, we have
∠OQR + ∠ORQ + ∠ROQ = 180°
⇒ ∠OQR + 25° + 90° = 180° [Diagonals of a rhombus are perpendicular to each other ⇒ ∠ROQ = 90°]
⇒ ∠OQR = 180° – 115° = 65°
∴ ∠RQS = 65°
Question. In ΔABC, AD is the median and DE || AB, such that E is a point on AC. Prove that BE is another median.
Ans. In ΔABC, DE || AB and AD is the median.
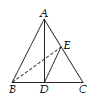
So, D is the mid-point of BC.
By converse of mid-point theorem,
E is the mid-point of AC.
Hence, BE is median.
Question. In ΔABC, ∠A = 50°, ∠B = 60° and ∠C = 70°. Find the measures of the angles of the triangle formed by joining the mid-points of the sides of this triangle.
Ans. Let D, E and F be the mid-points of sides BC, CA and AB respectively.
In ΔABC, F and E are mid-points of AB and AC.
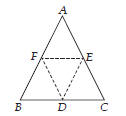
∴ FE || BC and FE = 1/2 BC
∴ FE || BD and FE = BD
∴ FEDB is a parallelogram.
Similarly, CDFE and AFDE are also parallelograms.
∴ ∠B = ∠DEF, ∠C = ∠DFE and ∠FDE = ∠A
⇒ ∠DEF = 60°, ∠DFE = 70° and ∠FDE = 50°
Question. In the given parallelogram ABCD, the sum of any two consecutive angles is 180° and opposite angles are equal. Find the value of ∠A.
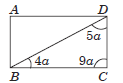
Ans. In ΔBCD, we have
∠BDC + ∠DCB + ∠CBD = 180° [Angle sum property of a triangle]
⇒ 5a + 9a + 4a = 180°
⇒ 18a = 180° ⇒ a = 10°
∴ ∠C = 9 × 10° = 90°
Since, opposite angles of a parallelogram are equal
Therefore, ∠A = ∠C ⇒ ∠A = 90°
Question. In given figure, ABCD is a parallelogram in which P is the midpoint of DC and Q is a point on AC such that CQ = 1/4 AC. If PQ produced meet BC at R, then prove that R is a midpoint of BC.
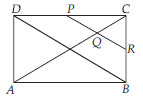
Ans. Suppose AC and BD intersect at O.
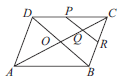
Then, OC = 1/2 AC
Now, CQ = 1/4 AC [Given]
⇒ CQ = 1/2 OC
In ΔCOD, P and Q are the midpoints of DC and OC respectively.
∴ PQ || DO [By mid-point theorem]
Also, in ΔCOB, Q is the midpoint of OC and QR || OB
∴ R is the midpoint of BC. [By converse of mid-point theorem]
Question. In the following figure, AL and CM are medians of ΔABC and LN || CM. Prove that BN = 1/4 AB.
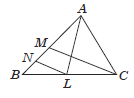
Ans. We have, AL and CM are medians of ΔABC, i.e., L and M are the mid-points of BC and AB respectively.
∴ LC = BL = 1/2 BC and BM = AM = 1/2 AB …(i)
In ΔBMC, L is the mid-point of BC and LN || CM. So, by converse of mid-point theorem, N is mid-point of BM.
i.e., BN = NM = 1/2 BM …(ii)
From (i) and (ii), we get
BN = 1/2 (1/2AB) ⇒ BN = 1/4 AB
Question. PQRS is a parallelogram and ∠SPQ = 60°. If the bisectors of ∠P and ∠Q meet at point A on RS, prove that A is the mid-point of RS.
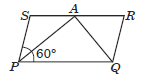
Ans. ∠P + ∠Q = 180° (Adjacent angles of parallelogram)
⇒ 60° + ∠Q = 180° ⇒ ∠Q = 120°
Since, PA and QA are bisectors of angles P and Q
∴ ∠SPA = ∠APQ = 1/2 ∠P = 1/2 × 60° = 30°
And ∠RQA = ∠AQP = 1/2 ∠Q = 1/2 × 120° = 60°
Now, SR || PQ and AP is transversal.
∴ ∠SAP = ∠APQ = 30° [Alternate interior angles]
In ΔASP, we have
∠SAP = ∠APS = 30°
⇒ SP = AS …(i)
(Sides opposite to equal angles are equal)
Similarly, QR = AR …(ii)
But, QR = SP [Opposite sides of parallelogram] …(iii)
From (i), (ii) and (iii), we have AS = AR
⇒ A is the mid-point of SR.
Question. In the given quadrilateral ABCD, X and Y are points on diagonal AC such that AX = CY and BXDY is a parallelogram. Show that ABCD is a parallelogram.
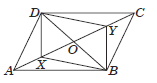
Ans. Since BXDY is a parallelogram.
∴ XO = YO …(i)
and DO = BO …(ii) [Diagonals of a parallelogram bisect each other]
Also, AX = CY (Given)…(iii)
Adding (i) and (iii), we have XO + AX = YO + CY
⇒ AO = CO …(iv)
From (ii) and (iv), we have
AO = CO and DO = BO
Thus, ABCD is a parallelogram, because diagonals AC and BD bisect each other at O.
Question. Rima has a photo-frame without a photo in the shape of a triangle with sides a, b, c in length. She wants to find the perimeter of a triangle formed by joining the mid-points of the sides of the photo-frame. Find the perimeter of the triangle formed by joining the mid-points of the frame.
Ans. Let the photo-frame be ABC such that BC = a,
CA = b and AB = c and the mid-points of AB, BC and CA are D, E and F respectively.
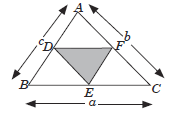
We have to determine the perimeter of ΔDEF.
In ΔABC, DF is the line-segment joining the mid-points of sides AB and AC.
By mid-point theorem, DF || BC and DF = BC/2 = a/2
Similarly, DE = AC/2 = b/2 and EF = AB/2 = c/2
∴ Perimeter of ΔDEF = DF + DE + EF
= a/2 + b/2 + c/2 = a + b + c/2
Question. In the given figure, ABCD is a square, side AB is produced to points P and Q in such a way that PA = AB = BQ. Prove that DQ = CP.
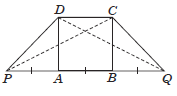
Ans. Since, ABCD is a square.
∴ AB = BC = CD = DA
Also, PA = AB = BQ
∴ AB = BC = CD = DA = PA = BQ
In ΔPDA and ΔQCB, PA = BQ (Given)
AD = BC (Sides of a square)
∠A = ∠B (Each 90°)
ΔPDA ≅ ΔQCB (By SAS congruency rule)
⇒ PD = QC (By C.P.C.T.) …(i)
∠PDA = ∠QCB (By C.P.C.T.) …(ii)
Now, ∠PDC = ∠PDA + ∠ADC
= ∠PDA + 90° …(iii)
∠QCD = ∠QCB + ∠BCD
= ∠QCB + 90° …(iv)
From (ii), (iii) and (iv), we have ∠PDC = ∠QCD
Now, in ΔPDC and DQCD, PD = QC (Given)
DC = DC (Common)
∠PDC = ∠QCD (Proved above)
ΔPDC ≅ DQCD (By SAS congruency rule)
∴ DQ = CP [By C.P.C.T.)
Question. In the given figure, M and P are the midpoints of AB, AC and BC respectively. If MN = 3 cm, NP = 3.5 cm and MP = 2.5 cm, then find (BC + AC) – AB.
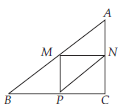
Ans. We have,
MN = 1/2 BC, MP = 1/2 AC and NP = 1/2 AB [By midpoint theorem]
⇒ BC = 6 cm, AC = 5 cm and AB = 7 cm.
The value of (BC + AC) – AB
= (6 + 5) – 7 = 4 cm.
Question. In a quadrilateral ABCD, CO and DO are the bisectors of ∠C and ∠D respectively. Prove that
∠COD = 1/2 (∠A + ∠B).
Ans. In ΔCOD, we have
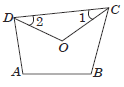
∠COD + ∠1 + ∠2 = 180°
⇒ ∠COD = 180° – (∠1 + ∠2)
⇒ ∠COD = 180° – (1/2∠C + 1/2∠D)
⇒ ∠COD = 180° – 1/2(∠C + ∠D)
⇒ ∠COD = 180° – 1/2 {360° – (∠A + ∠B)} [∵ ∠A + ∠B + ∠C + ∠D = 360°]
⇒ ∠COD = 1/2 (∠A + ∠B)
Question. In a parallelogram PQRS, if ∠QRS = 2x, ∠PQS = 4x and ∠PSQ = 4x, then find the angles of the parallelogram.
Ans. ∠SPQ = ∠QRS = 2x (∵ Opposite angles of a parallelogram are equal)
In ΔPSQ, ∠PSQ + ∠PQS + ∠SPQ = 180°
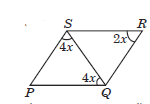
⇒ 4x + 4x + 2x = 180°
⇒ 10x = 180°
⇒ x = 18°
Now, ∠PSR = ∠PQR (∵ Opposite angles of a parallelogram are equal)
⇒ 4x + ∠QSR = 4x + ∠SQR
⇒ ∠QSR = ∠SQR …(i)
In DSRQ, ∠SRQ + ∠RSQ + ∠SQR = 180°
⇒ 2 × 18° + 2 ∠RSQ = 180° [From (i)]
⇒ 2 ∠RSQ = 180° – 36° = 144° ⇒ ∠RSQ = 72°
Hence, ∠P = ∠R = 2 × 18° = 36°,
∠Q = ∠S = 4x + 72° = 4 × 18° + 72° = 144°
Long Answer Type Questions:
Question. P, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC = BD and AC ^ BD. Prove that PQRS is a square.
Ans. In quadrilateral ABCD, AC ⊥ BD and AC = BD.
In ΔADC, S and R are the midpoints of the sides AD and DC respectively.
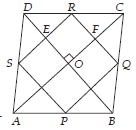
∴ SR || AC and SR = 1/2 AC …(i) [By mid-point theorem]
In ΔABC, P and Q are the midpoints of AB and BC respectively.
∴ PQ|| AC and PQ = 1/2 AC …(ii) [By mid-point theorem]
From (i) and (ii), PQ || SR
and PQ = SR = 1/2 AC …(iii)
Similarly, in ΔABD,
SP || BD and SP = 1/2 BD [By mid-point theorem]
∴ SP = 1/2 AC [∴ AC = BD] …(iv)
Now in ΔBCD, RQ || BD and RQ = 1/2 BD [By mid-point theorem]
∴ RQ = 1/2 AC [∴ BD = AC] …(v)
From (iv) and (v), SP = RQ = 1/2 AC …(vi)
From (iii) and (vi), PQ = SR = SP = RQ …(vii)
∴ All four sides are equal.
Now, in quadrilateral OERF,
OE || FR and OF || ER
∴ ∠EOF = ∠ERF = 90° [AC ⊥ DB]
∴ ∠QRS = 90° …(viii)
From (vii) and (viii), we get PQRS is a square.
Question. Prove that the line segment joining the midpoints of the diagonals of a trapezium is parallel to each of the parallel sides and is equal to half the difference of these sides.
Ans. Let, trapezium ABCD in which, AB || DC and P and Q are the mid-points of its diagonals AC and BD respectively.
We have to prove (i) PQ || AB and PQ || DC
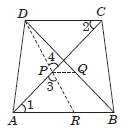
(ii) PQ = 1/2( AB − DC)
Join D and P and produce DP to meet AB at R.
(i) Since AB || DC and transversal
AC cuts them at A and C respectively.
∴ ∠1 = ∠2 (Alternate angles) ….(1)
In ΔAPR and ΔCPD,
∠1 = ∠2 (From (1))
AP = CP (Q P is the mid-point of AC)
∠3 = ∠4 (Vertically opposite angles)
∴ ΔAPR ≅ ΔCPD (By ASA congruence rule)
⇒ AR = DC and PR = DP (By C.P.C.T.)
In ΔDRB, P and Q are the mid-points of side DR and DB respectively.
∴ PQ || RB (By mid-point theorem)
⇒ PQ || AB (Q RB is a part of AB)
⇒ PQ || AB and PQ || DC (Q AB || CD)
(ii) In ΔDRB, P and Q are the mid-points of side DR and DB respectively.
∴ PQ = 1/2 RB (By mid-point theorem)
⇒ PQ = 1/2 (AB − AR) ⇒ PQ = 1/2(AB −DC) [From part (i), AR = DC]



