Check the below NCERT MCQ Class 12 Mathematics Chapter 13 Probability with Answers available with PDF free download. MCQ Questions for Class 12 Mathematics with Answers were prepared based on the latest syllabus and examination pattern issued by CBSE, NCERT and KVS. Our teachers have provided below Probability Mathematics Class 12 Mathematics MCQs Questions with answers which will help students to revise and get more marks in exams
Probability Class 12 Mathematics MCQ Questions with Answers
Refer below for MCQ Class 12 Mathematics Chapter 13 Probability with solutions. Solve questions and compare with the answers provided below
Question. Two dice are rolled one after the other. The probability that the number on the first is smaller than the number on the second is
(a) 1/2
(b) 7/18
(c) 3/4
(d) 5/12
Answer
D
Question. On hundred cards are numbered from 1 to 100. The probability that a randomly chosen card has a digit 5 is
(a) 1/100
(b) 9/100
(c) 19/100
(d) None of these
Answer
C
Question. Odds in favour of an event A are 2 to 1 and odds in favour of A∪ B are 3 to 1. Consistent with this information the smallest and largest values for the probability of event B are given by
(a)1/6≤P(B) ≤ 1/3
(b) 1/3≤p(B) ≤ 1/2
(c) 1/12≤p(B)≤ 3/4
(d) None of these
Answer
C
Question. A car is parked by a driver amongst 25 cars in a row, not at either end. When he returns he finds that 10 places are empty. The probability that both the neighbouring places of driver’s car are vacant, is
(a) 9/92
(b) 15/92
(c) 21/92
(d) 27/92
Answer
B
Question. If two events A and B are such that P (Ac)=0.3 p(B)=0.4, ((A∩Bc)= 0.5, then P(B/A∪Bc) is equal to
(a) 0.20
(b) 0.25
(c) 0.30
(d) 0.35
Answer
B
Question. The probability that a teacher will give an unannounced test during any class meeting is 1/5. If a student is absent twice, the probability that he will miss at least one test, is
(a) 7/25
(b) 9/25
(c) 16/25
(d) 24/25
Answer
B
Question. The probability of getting 10 in a single throw of three fair dice is
(a) 1/6
(b) 1/8
(c) 1/9
(d) None of these
Answer
B
Question. Probabilities of teams A ,B and C winning are 1/4, 1/6 and 1/8 respectively. Probability that one of these teams will win, is
(a) 13/24
(b) 11/24
(c) 23/24
(d) None of these
Answer
A
Question. A die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event, ‘number is even’ and B be the event, ‘number is red’, then A and B are
(a) mutually exclusive
(b) dependent
(c) independent
(d) None of these
Answer
C
Question Two balls are drawn at random with replacement from a box containing 10 black and 8 red balls. The probability that
(i) both balls are red
(ii) first ball is black and second is red
(iii) one of them is black and other is red are respectively
(a) 16/81,20/81 and 40/81
(b) 40/81,20/81 and 16/81
(c) 20/81,16/81 and 40/81
(d) None of these
Answer
A
Question. Events A and B are such that P (A )=1/2,P (B) =7/12 and
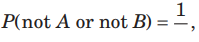
then A and B are
(a) independent
(b) not independent
(c) mutually exclusive
(d) None of these
Answer
B
Question. A box of oranges is inspected by examining three randomly selected oranges drawn without replacement. If all the three oranges are good, the box is approved for sale otherwise it is rejected. The probability that a box containing 15 oranges out of which 12 are good and 3 are bad one will be approved for sale, is
(a) 12/15
(b) 11/14
(c) 10/13
(d) 44/91
Answer
D
Question. Two events A and B are said to be independent, if
(a) A and B are mutually exclusive
(b) P (A’ ∩ B’) = [1-P(A)] [1-P(B)]
(c) P (A) = P (B)
(d) P (A) + P (B) = 1
Answer
B
Question. Probability of solving specific problem independently by A and B are 1/2 and /3, respectively.
If both try to solve the problem independently, the probability that
(i) the problem is solved
(ii) exactly one of them solves the problem are respectively
(a) 1/2 and 2/3
(b) 2/3 and 1/2
(b) 1/4 and 3/4
(d) None of these
Answer
B
Question. A bag contains 4 red and 4 black balls, another bag contains 2 red and 6 black balls. One of the two bags is selected at random and a ball is drawn from the bag which is found to be red. The probability that the ball is drawn from the first bag.
(a) 1/2
(b) 1/3
(c) 3/4
(d) 2/3
Answer
D
Question. A and B are two candidates seeking admission in a college. The probability that A is seleted is 0.7 and the probability that exactly one of them is selected is 0.6. The probability that B is selected, is
(a) 0.1
(b) 0.3
(c) 0.5
(d) 0.25
Answer
D
Question. An electronic assembly consists of two subsystems, say A and B. From previous testing procedures, then the following probabilities are assumed to be known P (A fails) = 0.2, P (B fails alone) = 0.15, P(A and B fail) = 0.15 The probabilities of (i) P (A fails/B has failed) (ii) P (A fails alone) are respectively
(a) 0.2 and 0.02
(b) 0.2 and 0.03
(c) 0.5 and 0.03
(d) 0.5 and 0.05
Answer
D
Question. If each element of a second order determinant is zero or one, what is the probability that the values of the determinant is positive?
(a) 3/16
(b) 5/16
(c) 15/16
(d) None of these
Answer
A
Question.
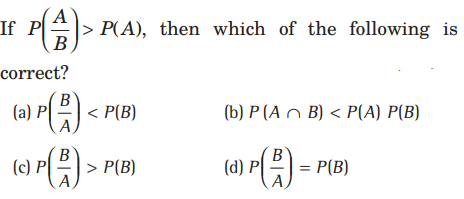
Answer
C
Question. Ten coins are tossed. Then, the probability of getting atleast 8 heads is
(a) 1/128
(b) 1/256
(c) 7/128
(d) 3/256
Answer
C
Question. Two dice are tossed. The following two events A and B are A x y = {(x,y) : x+y = 11},B= {(x,y): x≠5} where (x,y) denotes a typical sample point.
(a) Not independent
(b) independent
(c) Mutually exclusive
(d) None of the above
Answer
A
Question. Four cards are successively drawn without replacement from a deck of 52 playing cards. What is the probability that all the four cards are kings?
(a) 1/725
(b) 1/125
(c) 1/2025
(d) None of these
Answer
D
Question. A natural number is chosen at random from the first one hundred natural numbers. The probability that
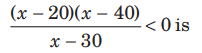
(a) 1/50
(b) 3/50
(c) 3/25
(d) 7/25
Answer
D
Question.

(a) n/(n+1)
(b) (n-1)/n+1)
(c) 1/(n+1)
(d) n+ (1/n+1)
Answer
C
Question. In a series of three trials the probability of exactly two successes in nine times as large as the probability of three successes. Then, the probability of success in each trial is
(a) 1/2
(b) 1/3
(c) 1/4
(d) 3/4
Answer
C
Question. Let E and F be two independent events such that P(E)>P(F).The probability that both E and F happen is 1/2, and the probability that neither E nor F happens is 1/2 , then
(a) P (E) = 1/3, P (F)=1/4
(b) P (E) = 1/2, P(F) = 1/6
(c) P (E) = 1, P (F) =1/12
(d) P (E) =1/3, p(F) = 1/2
Answer
B
Question. If two events A and B are such that P(A′)= 0.3, P (B) = 0.4 and (A ∩ B′ )= 0.5, then P (B/A∪B’) is equal to
(a) 1/4
(b) 1/5
(c) 3/5
(d) 2/5
Answer
A
Question. If the integers m and n are chosen at random from 1 to 100, then the probability that a number of the form 7n+ 7m is divisible by 5 equals
(a) 1/4
(b) 1/2
(c) 1/8
(d) 1/3
Answer
A
Question. The probability that in a group of N (< 365 ) people, at least two will have the same birthday is
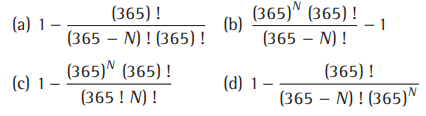
Answer
D
Question. If 2P (A) = P(B)= 5/13 and P(A/B) = 2/5, then P(A∪B) is
(a) 11/26
(b) 3/26
(c) 5/26
(d) None of these
Answer
A
Question. A coin is tossed three times E : at most two tails F : at least one tail, then P(E/F) is
(a) 2/7
(b) 6/7
(c) 1/7
(d) 3/7
Answer
B
Question. Three numbers are chosen at random without replacement from the set A {x|1≤ x ≤ 10,x ∈ N}. The probability that the minimum of the chosen numbers is 3 and maximum is 7, is
(a) 1/12
(b) 1/15
(c) 1/40
(d) 39/40
Answer
C
Question. A black and a red die are rolled, then the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4, is
(a) 1 /3
(b) 1/9
(c) 2/3
(d) 0
Answer
B
Question.

Answer
A
Question. A die is thrown three times,
E : 4 appears on the third toss
F : 6 and 5 appears, respectively on first two tosses then P(E/F) is
(a) 1/36
(b) 5/6
(c) 5/36
(d) 1/6
Answer
D
Question. The probability of obtaining an even prime number on each die, when a pair of dice is rolled is
(a) 0
(b) 1/3
(c) 1/12
(d) 1/36
Answer
D
Question. A and B are events such that P(A) = 0.4, P(B) = 0.3 and P(A∪B) = 0.5. Then P(B¢ ∩ A¢) equals
(a) 2/3
(b) 1/2
(c) 3/10
(d) 1/5
Answer
D
Question. If A and B are two events such that P(A) ≠ 0 and P(B|A) = 1, then
(a) A ⊂ B
(b) B ⊂ A
(c) B = j
(d) A = j
Answer
A
Question. Let P(A) = 7/13 = 9/13 , P(B) and P(A∩B) = . 4/13 Then P(A’/ B) is equal to
(a) 6/13
(b) 4/13
(c) 4/9
(d) 5/9
Answer
B
Question. If P(A|B) > P(A), then which of the following is correct:
(a) P(B|A) < P(B)
(b) P(A∩B) < P(A).P(B)
(c) P(B|A) > P(B)
(d) P(B|A) = P(B)
Answer
C
Question. If P(A) = 0.4, P(B) = 0.8 and P(B|A) = 0.6, then P(A∪B) is equal to
(a) 0.24
(b) 0.3
(c) 0.48
(d) 0.96
Answer
D
Question. A and B are two students. Their chances of solving a problem correctly are 1/3 and 1/4 , respectively. If the probability of their making a common error is 1/20 and they obtain the same answer, then the probability of their answer to be correct is
(a) 1/12
(b) 1/40
(c) 13/120
(d) 10/13
Answer
D
Question. If P(A) = 4/5 and P(A∩B)= 7/10 , then (P(B|A) is equal to
(a) 1/10
(b) 1/8
(c) 7/8
(d) 17/20
Answer
C
Question. In a college, 30% students fail in physics, 25% fail in mathematics and 10% fail in both. One student is chosen at random. The probability that she fails in physics if she has failed in mathematics is
(a) 1/10
(b) 2/5
(c) 9/20
(d) 1/3
Answer
B
Question. Two cards are drawn from a well shuffled deck of 52 playing cards with replacement. The probability, that both cards are queens, is
(a) 1/13 x 1/13
(b) 1/13 + 1/13
(c) 1/13 x 1/17
(d) 1/13 x 4/51´
Answer
A
Question. Two dice are thrown. If it is known that the sum of numbers on the dice was less than 6, the probability of getting a sum 3, is
(a) 1/18
(b) 5/18
(c) 1/5
(d) 2/5
Answer
C
Question. Eight coins are tossed together. The probability of getting exactly 3 heads is
(a) 1/256
(b) 7/32
(c) 5/32
(d) 3/32
Answer
B
Question. If A and B are such events that P(A) > 0 and P(B) ≠ 1, then P (A|B) equals
(a) 1 – P(A|B)
(b) 1 – P(A|B)
(c) 1- P(A∪B) / P(B)
(d) P(A)|P(B)
Answer
C
Question. A box contains 3 orange balls, 3 green balls and 2 blue balls. Three balls are drawn at random from the box without replacement. The probability of drawing 2 green balls and one blue ball is
(a) 3/28
(b) 2/21
(c) 1/28
(d) 167/168
Answer
A
Question. A die is thrown and a card is selected at random from a deck of 52 playing cards. The probability of getting an even number on the die and a spade card is
(a) 1/2
(b) 1/4
(c) 1/8
(d) 3/4
Answer
C
Question. Assume that in a family, each child is equally likely to be a boy or a girl. A family with three children is chosen at random. The probability that the eldest child is a girl given that the family has at least one girl is
(a) 1/2
(b) 1/3
(c) 2/3
(d) 4/7
Answer
D
Question. If A and B are any two events such that P(A) + P(B) – P(A and B) = P(A), then
(a) P(B|A) = 1
(b) P(A|B) = 1
(c) P(B|A) = 0
(d) P(B|A) = 0
Answer
B
Question. In a box containing 100 bulbs, 10 are defective. The probability that out of a sample of 5 bulbs, none is defective is
(a) 10–1
(b) (1/2)5
(c) (9/10)5
(d) 9/10
Answer
C
Question. Two events E and F are independent. If P(E) = 0.3, P(E ∪ F) = 0.5, then P(E|F) – P(F|E) equals
(a) 2/7
(b) 3/35
(c) 1/70
(d) 1/7
Answer
C
Question. If A and B are two independent events with P(A) = 3/5 and P(B) = 4/5 , then P(A’∩B’) equals
(a) 4/15
(b) 8/45
(c) 1/3
(d) 2/9
Answer
D
Question. If P(A) = 2/5 , P(B) = 3/10 and P(A∩B) = 1/5 , then P(A¢|B¢).P(B’|A’) is equal to
(a) 5/6
(b) 5/7
(c) 25/42
(d) 1
Answer
C
Case-based MCQs
I. Read the following text and answer the following questions on the basis of the same:
A coach is training 3 players. He observes that the player A can hit a target 4 times in 5 shots, player B can hit 3 times in 4 shots and the player C can hit 2 times in 3 shots.
Question. Let the target is hit by A, B: the target is hit by B and, C: the target is hit by A and C. Then, the probability that A, B and, C all will hit, is
(a) 4/5
(b) 3/5
(c) 2/5
(d) 1/5
Answer
C
Question. What is the probability that B, C will hit and A will lose?
(a) 1/10
(b) 3/10
(c) 7/10
(d) 4/10
Answer
A
Question. What is the probability that ‘any two of A, B and C will hit?
(a) 1/30
(b) 11/30
(c) 17/30
(d) 13/30
Answer
D
Question. What is the probability that ‘none of them will hit the target’?
(a) 1/30
(b) 1/60
(c) 1/15
(d) 2/15
Answer
B
Question. What is the probability that at least one of A, B or C will hit the target?
(a) 59/60
(b) 2/5
(c) 3/5
(d) 1/60
Answer
A
II. Read the following text and answer the following questions on the basis of the same:
The reliability of a COVID PCR test is specified as follows:
Of people having COVID, 90% of the test detects the disease but 10% goes undetected. Of people free of COVID, 99% of the test is judged COVID negative but 1% are diagnosed as showing COVID positive.
From a large population of which only 0.1% have COVID, one person is selected at random, given the COVID PCR test, and the pathologist reports him/her as COVID positive. (Image 206)
Question. What is the probability of the ‘person to be tested as COVID positive’ given that ‘he is actually having COVID’?
(a) 0.001
(b) 0.1
(c) 0.8
(d) 0.9
Answer
D
Question. What is the probability of the ‘person to be tested as COVID positive’ given that ‘he is actually not having COVID’?
(a) 0.01
(b) 0.99
(c) 0.1
(d) 0.001
Answer
A
Question. What is the probability that the ‘person is actually not having COVID’?
(a) 0.998
(b) 0.999
(c) 0.001
(d) 0.111
Answer
B
Question. What is the probability that the ‘person is actually having COVID given that ‘he is tested as COVID positive’?
(a) 0.83
(b) 0.0803
(c) 0.083
(d) 0.089
Answer
C
Question. What is the probability that the ‘person selected will be diagnosed as COVID positive’?
(a) 0.1089
(b) 0.01089
(c) 0.0189
(d) 0.189
Answer
B
III. Read the following text and answer the following questions on the basis of the same:
In answering a question on a multiple choice test for class XII, a student either knows the answer or guesses. Let 3/5 be the probability that he knows the answer and 2/5 be the probability that he guesses.
Assume that a student who guesses at the answer will be correct with probability 1/3 . Let E1, E2, E be the events that the student knows the answer, guesses the answer and answers correctly respectively. (Image 207)
Question. What is the value of P(E1)?
(a) 2/5
(b) 1/3
(c) 1
(d) 3/5
Answer
D
Question. Value of P(E|E1) is
(a) 1/3
(b) 1
(c) 2/3
(d) 4/5
Answer
B
Question. (Image 207)
(a) 11/15
(b) 4/15
(c) 1/5
(d) 1
Answer
A
Question. Value of
(a) 1/3
(b) 1/5
(c) 1
(d) 3/5
Answer
C
Question. What is the probability that the student knows the answer given that he answered it correctly?
(a) 2/11
(b) 5/3
(c) 9/11
(d) 13/3
Answer
C
IV. Read the following text and answer the following questions on the basis of the same:
In an office three employees Vinay, Sonia and Iqbal process incoming copies of a certain form. Vinay process 50% of the forms. Sonia processes 20% and Iqbal the remaining 30% of the forms. Vinay has an error rate of 0.06, Sonia has an error rate of 0.04 and Iqbal has an error rate of 0.03. (Image 207)
Question. The conditional probability that an error is committed in processing given that Sonia processed the form is:
(a) 0.0210
(b) 0.04
(c) 0.47
(d) 0.06
Answer
B
Question. The probability that Sonia processed the form and committed an error is:
(a) 0.005
(b) 0.006
(c) 0.008
(d) 0.68
Answer
C
Question. The total probability of committing an error in processing the form is:
(a) 0
(b) 0.047
(c) 0.234
(d) 1
Answer
B
Question. The manager of the company wants to do a quality check. During inspection he selects a form at random from the days output of processed forms.
If the form selected at random has an error, the probability that the form is NOT processed by Vinay is:
(a) 1
(b) 30/47
(c) 20/47
(d) 17/47
Answer
D
Question. Let A be the event of committing an error in processing the form and let E1, E2 and E3 be the events that Vinay, Sonia and Iqbal processed the form. The value of (Image 208)
(a) 0
(b) 0.03
(c) 0.06
(d) 1
Answer
D
V. Read the following text and answer the following questions on the basis of the same:
A group of people start playing cards. And as we know a well shuffled pack of cards contains a total of 52 cards. Then 2 cards are drawn simultaneously (or successively without replacement). (Image 208)
Question. If x = no. of kings = 0, 1, 2. Then P(x = 0) = ?
(a) 188/221
(b) 198/223
(c) 197/290
(d) 187/221
Answer
A
Question. If x = no. of kings = 0, 1, 2. Then P(x = 1) = ?
(a) 32/229
(b) 32/227
(c) 32/221
(d) 32/219
Answer
C
Question. If x = no. of kings = 0, 1, 2. Then P(x = 2) = ?
(a) 2/219
(b) 1/221
(c) 3/209
(d) 1/209
Answer
B
Question. Find P(X = 1) + P(X = 2).
(a) 188/221
(b) 1/221
(c) 189/221
(d) 220/221
Answer
C
Question. Find P(x ≥ 1).
(a) 188/221
(b) 1/221
(c) 189/221
(d) 220/221
Answer
D
VI. Read the following text and answer the following questions on the basis of the same:
Anand, Samanyu and Shah of SHORTCUTS classes were given a problem in Mathematics whose respective probabilities of solving it are 1/2 , 1/3 and 1/4. They were asked to solve it independently. (Image 209)
Based on the above data, answer any four of the following questions.
Question. The probability that Anand alone solves it is _______.
(a) 1/4
(b) 3/4
(c) 11/24
(d) 17/24
Answer
A
Question. The probability that the problem is not solved is _______.
(a) 1/4
(b) 3/4
(c) 0
(d) 11/24
Answer
A
Question. The probability that the problem is solved is _______.
(a) 1/4
(b) 3/4
(c) 17/24
(d) 11/24
Answer
B
Question. The probability that exactly one of them solves it is _______.
(a) 1/4
(b) 3/4
(c) 17/24
(d) 11/24
Answer
D
Question. The probability that exactly two of them solves it is _______.
(a) 1/4
(b) 3/4
(c) 17/24
(d) 11/24
Answer
A
