Sequence and Series Class 11 MCQ Questions have been gathered for students to rehearse. Students can prepare these MCQ on Sequence and Series Class 11. Each question has four choices with answers. Firstly, Solve all these Questions and check your answer with the given answer. If your answers do not match with the right answer, Don’t worry try again because You need to prepare daily to score higher marks in the Class 11 Maths Exam.
Students can refer to the following Sequences and Series Class 11 MCQ Question with Answers provided below based on the latest curriculum and examination pattern issued by CBSE and NCERT. Our teachers have provided here a collection of multiple choice questions for Sequences and Series Class 11 covering all topics in your textbook so that students can assess themselves on all important topics and thoroughly prepare for their exams
Sequence and Series Class 11 MCQ Question with Answers
We have provided below Sequences and Series Class 11 MCQ Question with answers which will help the students to go through the entire syllabus and practice multiple choice questions provided here with solutions. As Class 11 Sequence and Series MCQ pdf download can be really scoring for students, you should go through all problems provided below so that you are able to get more marks in your exams.
Question. The product of first nine terms of a GP is, in general, equal to which one of the following?
(a) The 9th power of the 4th term
(b) The 4th power of the 9th term
(c) The 5th power of the 9th term
(d) The 9th power of the 5th term
Answer
D
Question. In a G.P. if (m + n)th terms is p and (m – n)th term is q, then mth term is:
(a) p/q
(b) q/p
(c) pq
(d) √pq
Answer
D
Question. A sequence containing a finite number of terms is called a……………….
(a) Finite sequence
(b) A.P. sequence
(c) G.P. sequence
(d) General sequence
Answer
A
Question. The third term of a geometric progression is 4.
The product of the first five terms is :
(a) 43
(b) 45
(c) 44
(d) 47
Answer
B
Question. In an AP. the pth term is q and the (p + q)th term is 0. Then the qth term is
(a) – p
(b) p
(c) p + q
(d) p – q
Answer
B
Question. If roots of the equation x3 – 12x2 + 39 x – 28 = 0 are in AP, then its common difference is
(a) ± 1
(b) ± 2
(c) ± 3
(d) ± 4
Answer
C
Question. If a, b, c, d, e, f are in A.P., then e – c is equal to:
(a) 2(c – a)
(b) 2(d – c)
(c) 2(f – d)
(d) (d – c)
Answer
B
Question. The fourth, seventh and tenth terms of a G.P. are p, q, r respectively, then :
(a) p2 = q2 + r2
(b) q2 = pr
(c) p2 = qr
(d) pqr + pq + 1 = 0
Answer
B
Question. A man saves ₹135/- in the first year, ₹150/- in the second year and in this way he increases his savings by ₹15/- every year. In what time will his total savings be ₹5550/-?
(a) 20 years
(b) 25 years
(c) 30 years
(d) 35 years
Answer
A
Question. The A. M. between two positive numbers a and b is twice the G. M. between them. The ratio of the numbers is
(a) (√2 + 3) : (√2 – 3)
(b) (2 + √3 ) : (2 – √3 )
(c) ( √3 + 1) : ( √3 – 1)
(d) None of these
Answer
B
Question. If 1, a and P are in A. P. and 1, g and P are in G. P., then
(a) 1 + 2a + g2 = 0
(b) 1 + 2a – g2 = 0
(c) 1 – 2a – g2 = 0
(d) 1 – 2a + g2 = 0
Answer
D
Question. For a, b, c to be in G.P. What should be the value of a-b /b-c ?
(a) ab
(b) bc
(c) a/b or b/c
(d) None of these
Answer
C
Question. What is the sum of terms equidistant from the beginning and end in an A.P. ?
(a) First term – Last term
(b) First term × Last term
(c) First term + Last term
(d) First term ¸ Last term
Answer
C
Question. Find 4th term from the end of the G.P. 3, 6, 12, 24., ………., 3072.
(a) 348
(b) 843
(c) 438
(d) 384
Answer
D
Question. If ax = by = cz, where a, b, c are in G.P. and a,b, c, x, y, z ¹ 0; then x, y, z are in:
(a) A.P.
(b) G..P.
(c) H.P
(d) none of these
Answer
C
Question. If a, b, c are in G.P. then 1/a2-b2 + 1/b2 is
(a) 1/c2 – b2
(b) 1/b2-c2
(c) 1/c2 – a2
(d) 1/b2-a2
Answer
B
Question. The value of 3 –1 + 1/3 – 1/9 +……….is equal to:
(a) 20/9
(b) 9/20
(c) 9/4
(d) 4/9
Answer
C
Question. If the pth, qth and rth terms of a G.P. are again in G.P., then which one of the following is correct?
(a) p, q, r are in A.P.
(b) p, q, r are in G.P.
(c) p, q, r are in H.P.
(d) p, q, r are neither in A.P. nor in G.P. nor in H.P.
Answer
A
Question. Let a1, a2, a3, ………..be the sequence, then the sum expressed as a1 + a2 + a3 + …… + an is called ……..
(a) Sequence
(b) Series
(c) Finite
(d) Infinite
Answer
B
Question. A sequence may be defined as a
(a) relation, whose range Í N (natural numbers)
(b) function whose range Í N
(c) function whose domain Í N
(d) progression having real values
Answer
C
Question. If 5 (3a – 1 + 1), (62a – 3 +2) and 7(5a – 2 + 5) are in AP, then what is the value of a?
(a) 7
(b) 6
(c) 5
(d) none
Answer
D
Question. If x + 1, 4x + 1, and 8x + 1 are in geometric progression, then what is the non-trivial value of x ?
(a) – 1
(b) 1
(c) 1/8
(d) 1/4
Answer
C
Question. The 10 th common term between the series 3 + 7 + 11 + …. and 1 + 6 + 11 + …. is
(a) 191
(b) 193
(c) 211
(d) None of these
Answer
A
Question. If p, q, r are in A.P., a is G.M. between p and q and b is G.M. between q and r, then a2, q2, b2 are in
(a) G.P.
(b) A.P.
(c) H.P
(d) None
Answer
B
Question. If pth term of an AP is q, and its qth term is p, then what is the common difference ?
(a) – 1
(b) 0
(c) 2
(d) 1
Answer
A
Question. Let x be one AM and g1 and g2 be two G.Ms between y and z. What is g13 + g23 equal to ?
(a) xyz
(b) xy2z
(c) xyz2
(d) 2xyz
Answer
D
Question. If 1, x, y, z, 16 are in geometric progression, then what is the value of x + y + z ?
(a) 8
(b) 12
(c) 14
(d) 16
Answer
C
Question. In a Geometric Progression with first term a and common ratio r, what is the Arithmetic Mean of the first five terms?
(a) a + 2r
(b) a r2
(c) a (r5 – 1)/[5(r – 1)]
(d) a (r4 – 1)/[5(r – 1)]
Answer
C
Question. What is the sum of the first 50 terms of the series (1 × 3) + (3 × 5) + (5 × 7) + …. ?
(a) 1,71,650
(b) 26,600
(c) 26,650
(d) 26,900
Answer
A
Question. If a, b, c are in geometric progression and a, 2b,3c are in arithmetic progression, then what is the common ratio r such that 0 < r < 1 ?
(a) 1/3
(b) 1/2
(c) 1/4
(d) 1/8
Answer
A
Question. Let bi > 1 for i = 1, 2,…,101. Suppos logeb1,logeb2,…logeb101 are in Arithmetic Progression (A.P.) with the common difference loge 2. Suppose a1,a2….a101 are in
A.P. such that a1=b1 and a51=b51
.If t = b1 + b2 +…+ b51 and
1 2 51 s = a + a +….+ a , then :
a. s > t anda101 > b101
b. s > t and a101 < b101
c. s < t and a101 > b101
d. s < t and a101 <b101
Answer
B
Question. Let
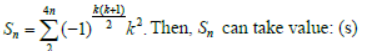
a. 1056
b. 1088
c. 1120
d. 1332
Answer
A,D
Question. 1+ 3+ 7 +15 + 31+………. to n terms = ?
a. 2n+1 − n
b. 2n+1 − n−2
c. 2n − n−2
d. None of these
Answer
B
Question. Let a =1 ⋅ 2 ⋅ 3 ⋅ 4 ⋅ 5. Then:
a. 55 ≥ a
b. 3 3 ≥ 5!
c. 55 ≥ 6a
d. none of these
Answer
A,B
Question. Let S1,S2…be squares such that for each n ≥1, the length of a side of Sn B,C,
equals the length of a diagonal of Sn+1. If the length of a side of S1is 10cm, then for which of the following values of n is the area of Sn less 1sq cm2 than:
a. 7
b. 8
c. 9
d. 10
Answer
D
Question. The sum of n terms of the series
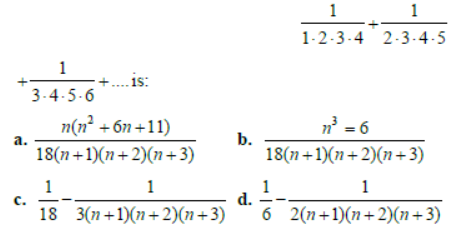
Answer
A,C
Assertion and Reason
Note: Read the Assertion (A) and Reason (R) carefully to mark the correct option out of the options given below:
a. If both assertion and reason are true and the reason is the correct explanation of the assertion.
b. If both assertion and reason are true but reason is not the correct explanation of the assertion.
c. If assertion is true but reason is false.
d. If the assertion and reason both are false.
e. If assertion is false but reason is true.
Question. The natural numbers are divided into rows as follows:

Assertion: Sum of the numbers in the 10th row is a number which can be written as sum of two cubes in two different ways.
Reason: Sum of the numbers in the rth row is
Answer
C
Question. Suppose a2,b2,c2 are in A.P.
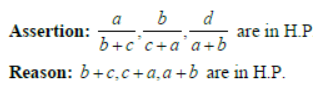
Answer
D
Question. Assertion:
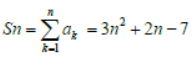
for each n, then, a1 ,a2 ,a3 … are in A.P D
Reason: Sum to n terms of an A.P. is always of the form an2 + bn.
Answer
D
Question. Assertion: The largest interval for which we can find the sum of the series +(2x−1) +(2x−1)2 +(2x−1)3 +… is (0, 1)
Reason: An infinite geometric series 2 a + ar + ar +… can be summed up if | r | <1.
Question. Assertion: If a, b, c > 0 and the expression (a + c)x2
√(a + c)2 + 4b2 )x + b > 0 ∀ x∈R, then a,b,c are in G.P.
Reason: 2 ax + bx + c > 0 ∀ x∈R if and only if a > 0 and b2 − 4ac < 0.
Answer
D
Question. Let α ,β be roots of 2 ax + 2bx + c = 0 and 2 ) = b − ac.
Assertion: If α +β ,α2 + β2 ,α3 +β3 are in G.P. then c) = 0
Reason: If α ,αβ ,β are in A.P. then b + c = 0.
Answer
B
Question. Suppose four distinct positive numbers a1 ,a2 ,a3 ,a4 are in G.P.
Let b1 = a1 ,b2 = b1 + a2 ,b3 = b2 + a3 and b4 = b3 + a4 .
Assertion: The numbers b1 ,b2 ,b3 ,b4 are neither in A.P. nor in G.P.
Reason: The numbers b1 ,b2 ,b3 ,b4 are in H.P.
Answer
C
Question. Let a, b, c, d, e be five non-zero numbers such that a, b, c are in A.P., b, c, d are in G.P., and c, d, e are in H.P.?
Assertion: If a = 2,e =18,b > 0, then b = 4 and c = 6.
Reason: If a = 2,e =18,c = −6, then b < 0.
Answer
B
Question. Suppose a, b, c, d are four real number and
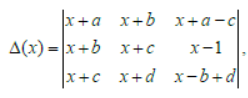
Assertion: If a, b, c, d are in A.P. and ∫02)△(x)dx = −4, then common difference of the A.P. is ± 1.
Reason: If a,b,c,d are in A.P., then △(x) is independent of x.
Answer
B
Question. Suppose four distinct positive numbers a1 , a2 ,a3 ,a4 are in
GP. Let b1 = a1 ,b2 = b1 + a2 ,b3 = b2 + a3 and b4 = b3 + a4
Assertion: The numbers b1 ,b2 ,b3 ,b4 are neither in AP nor in GP.
Reason: The numbers b1 ,b2 ,b3 ,b4 are in HP
Answer
C
Question. Assertion: The sum of the series 1 + (1 + 2 + 4) + (4 + 6 + 9) + (9 + 12 + 16) is 800.
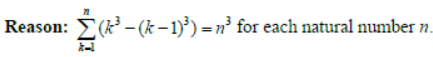
Answer
A
Paragraph –I
Let r V denotes the sum of the first r terms of an arithmetic progression (AP) whose first term is r and the common
difference is (2r – 1). Let Tr = Vr+1 − Vr − 2 = and Qr = Tr+1 − Tr = for r = 1, 2, …
Question. Tr is always:
a. an odd number
b. an even number
c. a prime number
d. a composite number
Answer
D
Question. Which one of the following is a correct statement?
a. Q1 , Q2, Q3,…are in AP with common difference 5
b. Q1 , Q2, Q3,…are in AP with common difference 6
c. Q1 , Q2, Q3,…are in AP with common difference 11
d. Q1 = Q2= Q3= …
Answer
B
Paragraph –II
Let A1, G1, H1 denote the arithmetic, geometric and harmonic means, respectively, of two distinct positive numbers. For n ≥ 2,
let An – 1 and Hn – 1 has arithmetic, geometric and harmonic means as An, Gn, Hn respectively.
Question. Which one of the following statements is correct ?
a. G1 > G2 > G3 > …
b. G1 < G2 , < G3 > …
c. G1 = G2 = G3 = …
d. G1 < G3 < G3 < … and G2 > G4 > G6 > …
Answer
A
Question. Which of the following statements is correct ?
a. A1 > A2 >…
b. A1 < A2 < A3 < ….
c. A1 > A3 > A5 > …. and A2 < A4 < A6 < ….
d. A1 < A3 < A5 < ….and A2 > A4 > A6 > ….
Answer
A
Question. Which of the following statements is correct ?
a. H1 > H2 > H3 > ….
b. H1 < H2 < H3 < ….
c. H1 > H3 > H5 > ….and H2 < H4 < H6 < ….
d. H1 < H3 < H5 < ….and H2 > H4 > H6 > ….
Answer
B
Paragraph –III
Let an denote the number of all n-digit positive integers formed by the digits 0, 1 or both such that no consecutive digits in them are 0. Let bn = The number of such n-digit integers ending with digit 1 and cn =
The number of such n-digit integers ending with digit 0.
Match the Column
Answer: If an+1 + bn+1/an + bn then:
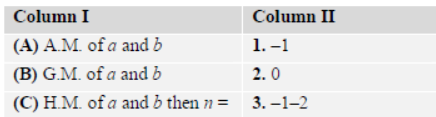
a. A→2, B→3, C→1
b. A→1, B→2, C→3
c. A→3, B→2, C→1
d. A→3, B→1, C→2
Answer
A
Question. Match the statement of Column I with those in Column II:
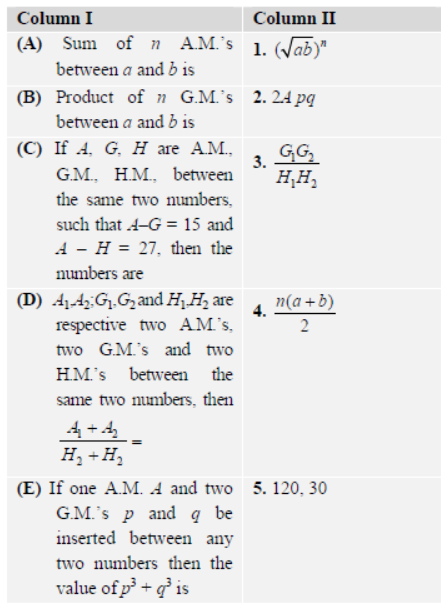
a. A→4, B→1, C→5, D→3, E→2
b. A→4, B→2, C→3, D→5, E→1
c. A→2, B→3, C→5, D→1, E→4
d. A→ 5, B→ 1, C→4, D→3, E→2
Answer
B
Question. Which of the following is correct ?
a. a17 = a16 + a15
b. a17 ≠ a16 + a15
c. b17 ≠ b16 + c16
d. a17 = c16 + b16
Answer
A
Question. The value of b6 is:
a. 7
b. 8
c. 9
d. 11
Answer
B
Question. a, b, c∈R and a, b, c are in A.P. math the entries of column I with those of column II:

a. A→1, B→1, C→1.2.3, D→4
b. A→1, B→2, C→1.4.3, D→3
c. A→2, B→3, C→1.2.3, D→4
d. A→1, B→3, C→1.2.3, D→2
Answer
A
Question. If

then the least value of n must be:
Answer
8
Question. If (1) (2010) + (2) (2009) + (3) (2008) + . . .+ (2010) (1) = (335) (2011) (λ), then the value of λ must be:
Answer
2021
Question. If
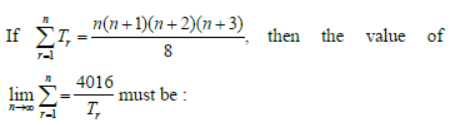
Answer
2008
Question. If a, b, c are in HP and if (a+2b/2a−b) + (C+b/2c−b) > √λ√λ√λ…..∞ then the value of λ must be :
Answer
4
Question. Let Sk, k = 1,2…100 denote the sum of the infinite geometric series whose first term is k–1/k! nd the common ratio is 1/k. Then the value of 1002/100!
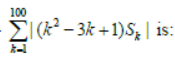
Answer
4
Question. The minimum value of the sum of real numbers a−5 , a−4 , 3a−3 ,1, a8 and a10 a with a > 0 is:
Answer
8
Question. A pack contains n card numbered from 1 to n. Two consecutive numbered card are removed from the pack and the sum of the numbers on the remaining cards is 1224. If the smaller of the numbers on the removed cards is k, then k – 20 is equal to:
Answer
5
Question. Let a, b, c be positive integers such that b/a is an integer. If a,b, c are in geometric progression and the arithmetic mean of a, b, c is b + 2, then the value of a2 + a−14/a+1 is:
Answer
4
Question. Let a1,a2,a3,…a11 be real numbers satisfying a1=15,27–2a2 > 0 and ak = 2ak−1−ak−2 for k = 3,4……11 IF a12 + a22 +…..a112 /11 = 90, then the value of a1+ a2+…..a11 / 11 is equal to:
Answer
0
Question. Let a1 , a2 , a3 , …, a100 be an arithmetic progression with a1 = 3 and
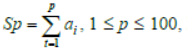
For any integer n with 1≤ n ≤ 20, let m = 5n. If Sm/Sn does not depend on n, then an is:
Answer
3,9
Question.
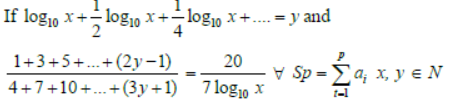
then the value of (logy x)5 must be :
Answer
3125
Our teachers have developed really good Multiple Choice Questions covering all important topics in each chapter which are expected to come in upcoming tests and exams, as MCQs are coming in all exams now therefore practice them carefully to get full understanding of topics and get good marks. Download the latest questions with multiple choice answers for Class 11 Sequences and Series in pdf or read online for free.
The above NCERT based Sequences and Series Class 11 MCQ Question has been designed by our teachers in such a way that it will help you a lot to gain an understanding of each topic. These CBSE NCERT Class 11 Sequences and Series Multiple Choice Questions have been developed and are available free for benefit of Class 11 students.
Advantages of Sequences and Series Class 11 MCQ Question with Answers
a) MCQs will help the kids to strengthen concepts and improve marks in tests and exams.
b) Multiple Choice Questions for Sequences and Series Class 11 have proven to further enhance the understanding and question-solving skills.
c) Regular reading topic wise questions with choices will for sure develop very good hold over each chapter which will help in exam preparations.
d) It will be easy to revise all Sequences and Series chapters and faster revisions prior to class tests and exams.
Free Printable MCQs in PDF of CBSE Class 11 Sequences and Series is designed by our school teachers and provide the best study material as per CBSE NCERT standards.
We hope this Class 11 Sequence and Series MCQ shared with you will help you to get good marks in your exam. We have also other study material like NCERT Solutions, NCERT Book, Exam Question, and simpler paper of Class 11. If You want to score higher in your exam, So practice all this study material and if you have any problem in regard to the MCQ on Sequence and Series Class 11 then, write it in the comment box and we will guide you as much as possible.

You can easily get MCQs for Sequences and Series from https://cbsencertsolutions.com
The MCQs for Class 11 Sequences and Series with Answers have been developed based on the current NCERT textbook issued by CBSE.
MCQs cover the topics of all chapters given in NCERT Book for Class 11 Sequences and Series.
Yes – These Multiple Choice Questions for Class 11 Sequences and Series with Answers are free to print and use them later.
No – All MCQs for Sequences and Series are free to read for all students.
Just scroll and read the free MCQs.
Yes – you can download free MCQs in PDF for Sequences and Series in standard MCQs format with Answers.
