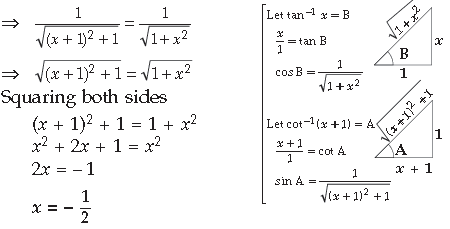Students can read the important questions given below for Inverse Trigonometric Functions Class 12 Mathematics. All Inverse Trigonometric Functions Class 12 Notes and questions with solutions have been prepared based on the latest syllabus and examination guidelines issued by CBSE, NCERT and KVS. You should read all notes provided by us and Class 12 Mathematics Important Questions provided for all chapters to get better marks in examinations. Mathematics Question Bank Class 12 is available on our website for free download in PDF.
Important Questions of Inverse Trigonometric Functions Class 12
Short Answer Type Questions
Question. Write the principal value of
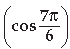
Answer.
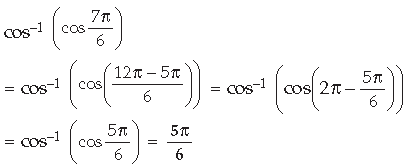
Question. Using the principal values, evaluate the following :
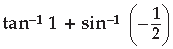
Answer.
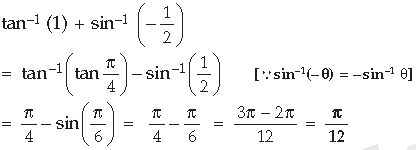
Question. Using principal values evaluate the following :
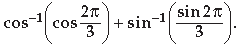
Answer,
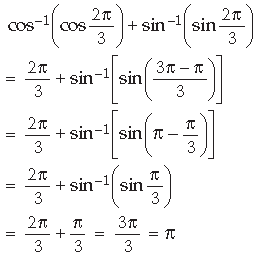
Question. What is the principal value of
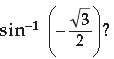
Answer.
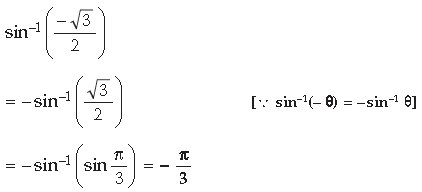
Question. What is the principal value of
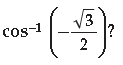
Answer.
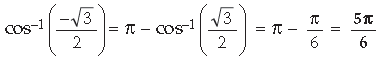
Question. Find the principal value of
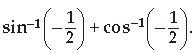
Answer.
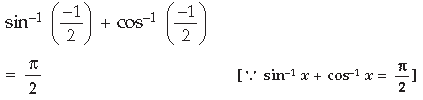
Question. Find the value of
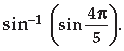
Answer.
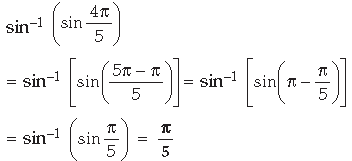
Question. Using principal value, find the value of
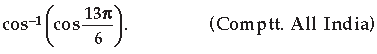
Answer.
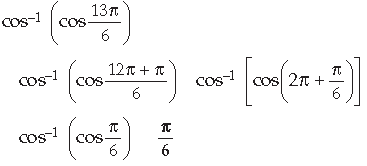
Question.
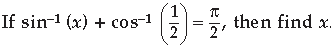
Answer.
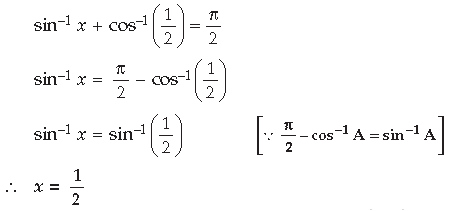
Question. If sec–1(2) + cosec–1(y) = π/2, then find y.
Answer. sec– 1 (2) + cosec – 1 y = π/2
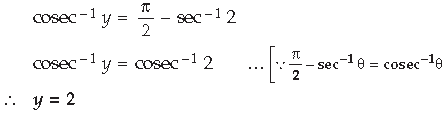
Question. What is the principal value of
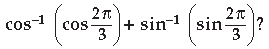
Answer.
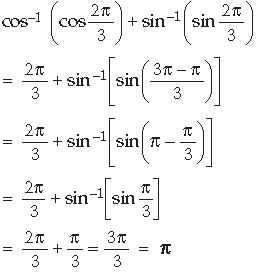
Question. Write the principal value of tan–1 (– 1). (Foreign)
Answer. tan–1 (– 1)
= – tan–1 (1) [tan–1 (– !) = – tan–1 !]
= – tan–1 (tan π/4) = –π/4
Question. Write the value of
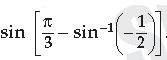
Answer.
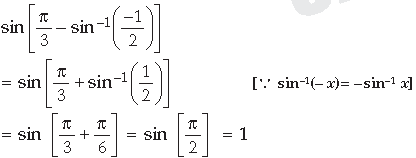
Question. Write the value of
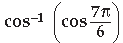
Answer.
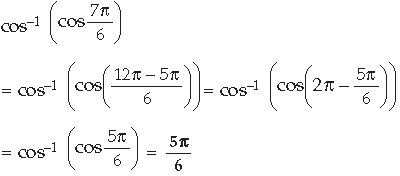
Question. Find the principal value of tan–1 √3 – sec–1 (– 2).
Answer. tan–1 (√3 ) – sec–1 (– 2)
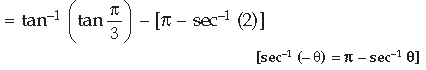
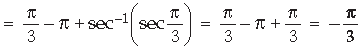
Question. Using principal values, write the value of
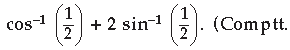
Answer.
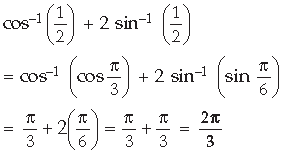
Question. If tan–1 x + tan–1 y = π/4, where xy < 1, find the value of x + y + xy.
Answer. tan–1 x + tan–1 y = π/4
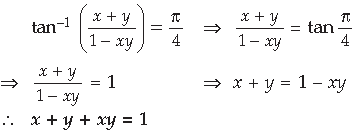
Question. Write the principal value of
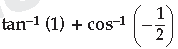
Answer.
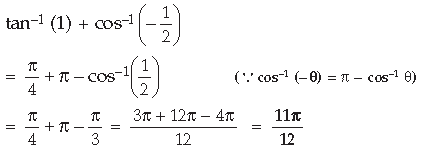
Question. Write the value of
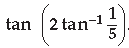
Answer.
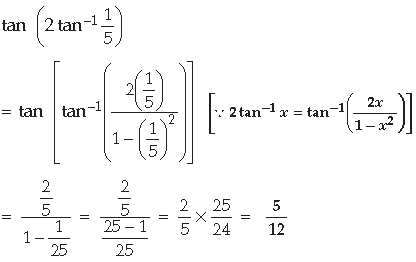
Question. Write the value of
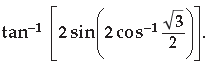
Answer.
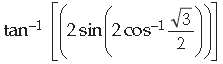
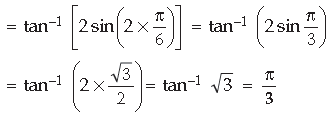
Question. Write the principal value of
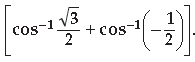
Answer.
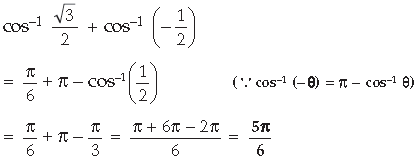
Question. Write the principal value of tan–1 (√3 ) – cot–1(–√3).
Answer. tan–1 (√3 ) – cot–1(–√3)
= tan–1√3 – (π – cot–1√3)
( ∴ cot–1 (– !) = π – cot–1 π)
= tan–1 √3 + cot–1 √3 – π
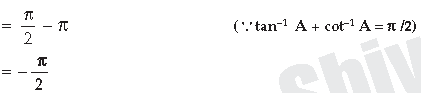
Question. Evaluate :
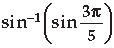
Answer.
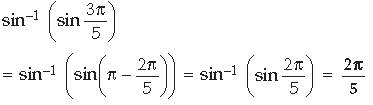
Question.
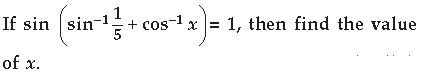
Answer.
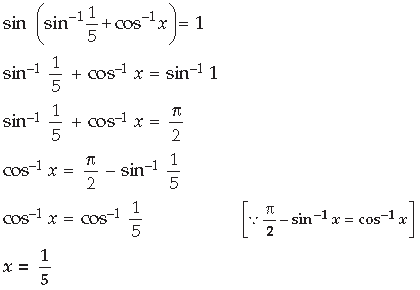
Question. If tan–1 x + tan–1 y = π/4, xy < 1, then write the value of x + y + xy.
Answer.
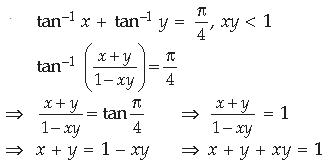
Question. Write the principal value of cos–1[cos(680º)].
Answer. cos–1 [cos (680°)]
= cos–1 [cos (2 × 360 – 40)] [ ∴ cos (n × 360 – !) = cos]
= cos–1 (cos 40°) = 40°
Question. Write the principal value of
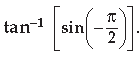
Answer.
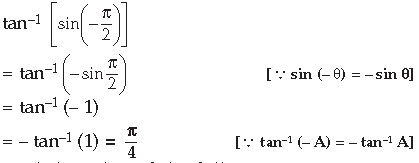
Question. Find the value of the following :
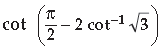
Answer.
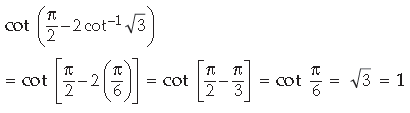
Question. Prove that :
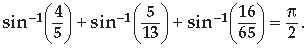
Answer.
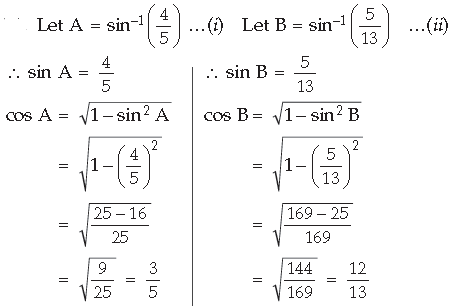
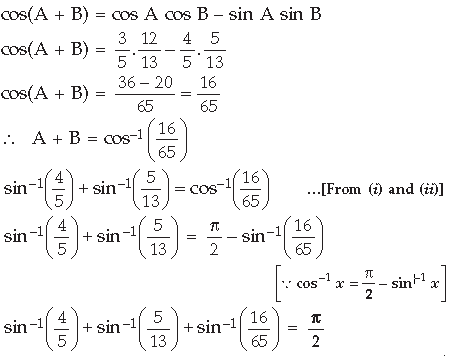
Question. Prove the following :
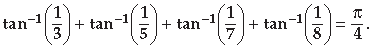
Answer.
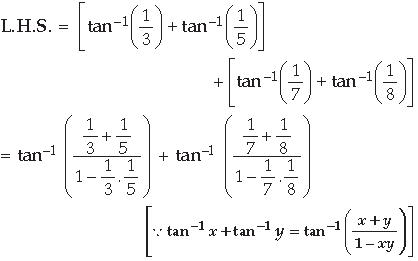
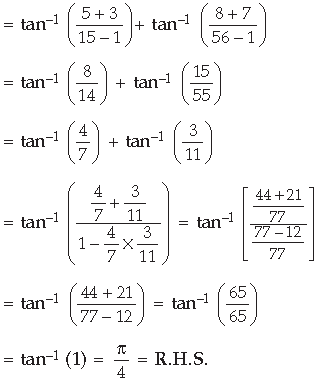
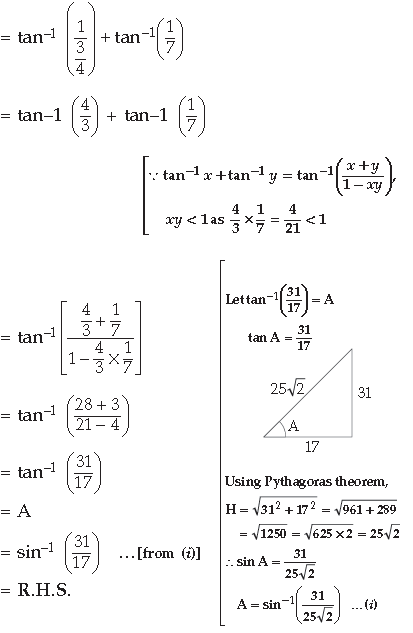
Question. Prove the following :
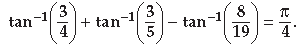
Answer. L.H.S.
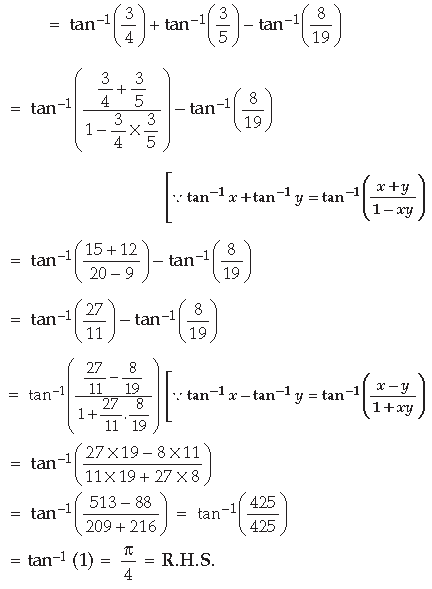
Question. Solve for x : tan–1 3x + tan–1 2x = π/4
Answer. tan–1(2x) + tan–1(3x) = π/4
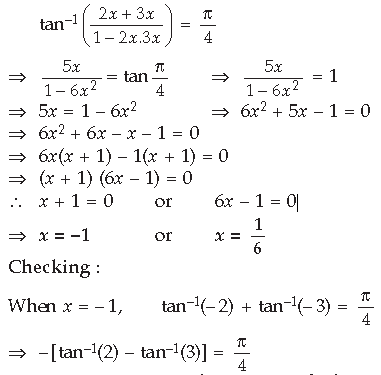
Rejecting x = – 1, it not does satisfy the equation as the L.H.S. of (i) is –ve. and R.H.S. of (i) is +ve Hence x = 1/6
Question. Solve the following for x :
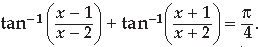
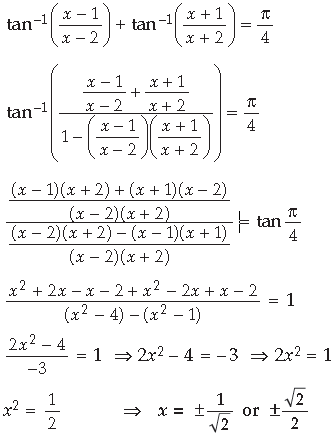
Question. Solve the following for x : tan–1 x + 2 cot–1 x = 2π/3
Answer. tan–1 x + 2 cot–1 x = 2π/3
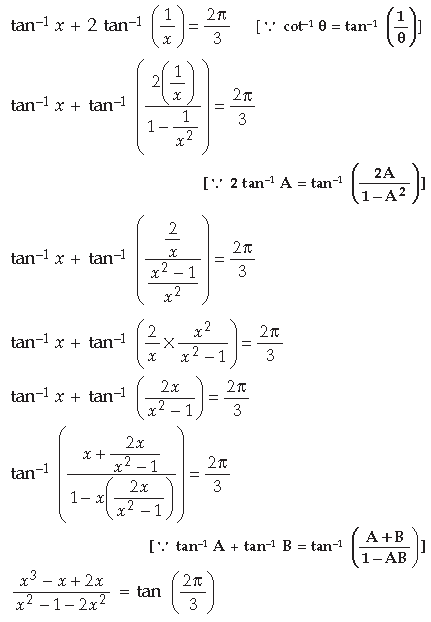
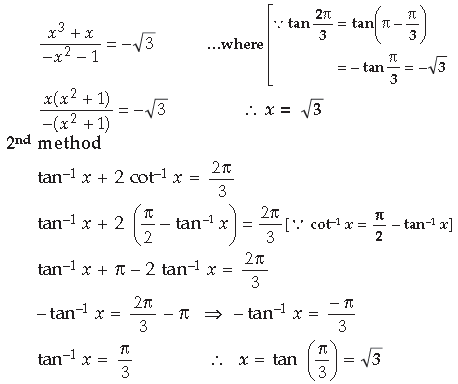
Question. Prove the following :
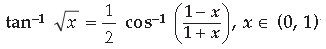
Answer.
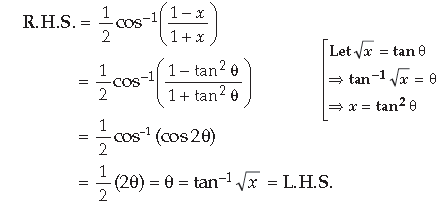
Question. Prove the following :
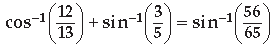
Answer.
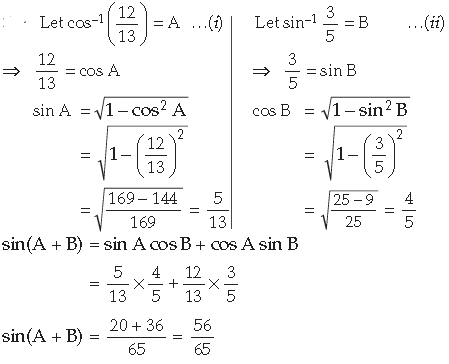
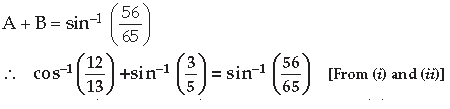
Question. Prove that : tan–1 (1) + tan–1 (2) + tan–1 (3) = π.
Answer. L.H.S. = tan–1 (1) + tan–1 (2) + tan–1 (3)
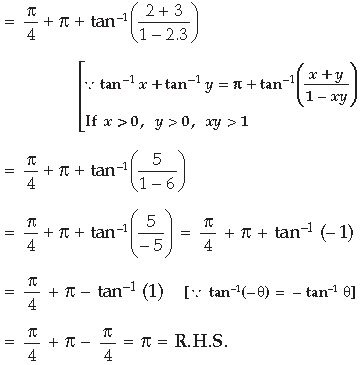
Question. Prove the following :
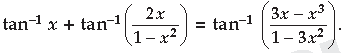
Answer.
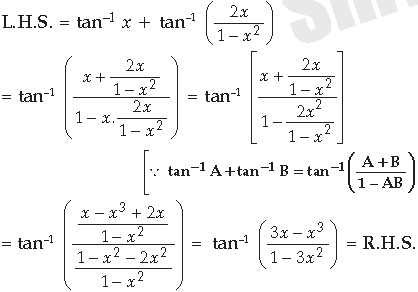
Question. Prove the following : cos [tan–1 {sin(cot–1 x)}]
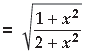
Answer. L.H.S. = cos[tan–1 {sin(cot–1 x)}]

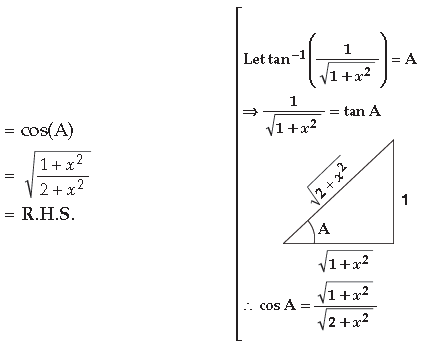
Question. Prove the following :
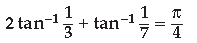
Answer.
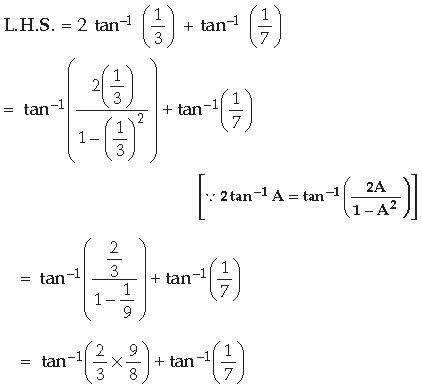
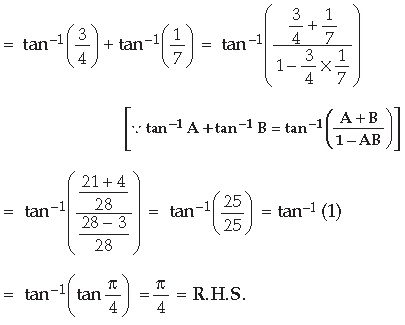
Question. Prove the following :
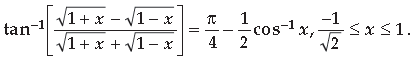
Answer.
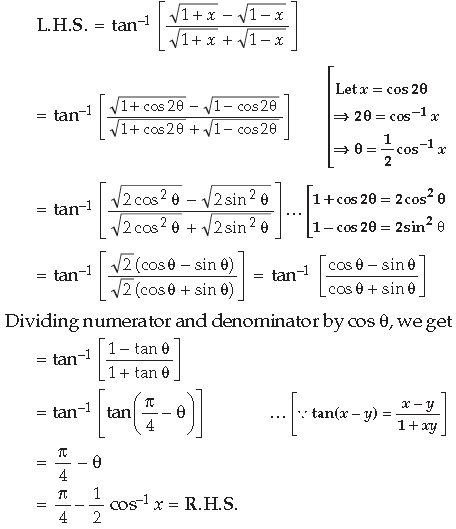
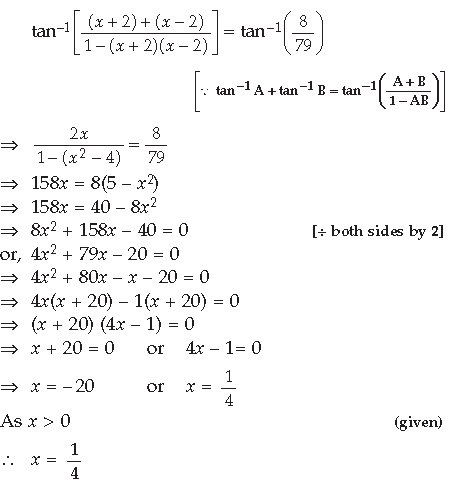
Question. Solve for x : tan–1 (x + 2) + tan–1 (x – 2) = tan–1 (8/79) x > 0.
Answer. tan–1 (x + 2) + tan–1 (x – 2) = tan–1 (8/79)
Question.
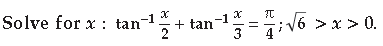
Answer.
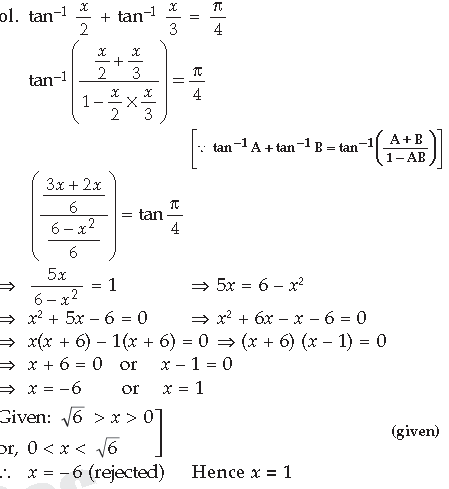
Question. Solve for x : 2 tan–1 (sin x) = tan–1(2 sec x)
Answer. 2 tan–1(sin x) = tan–1(2 sec x)
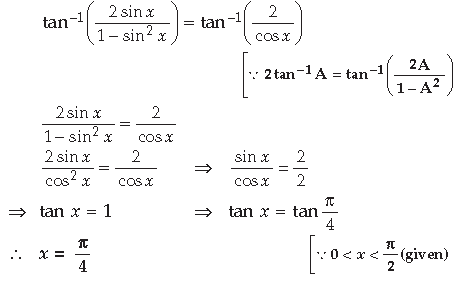
Question.
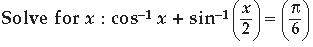
Answer.
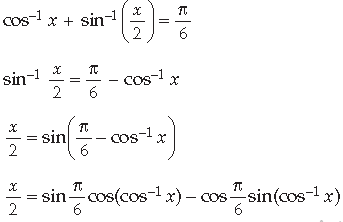
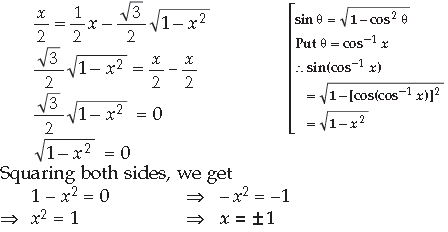
Question. Prove the following :
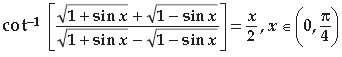
Answer. 1st method :
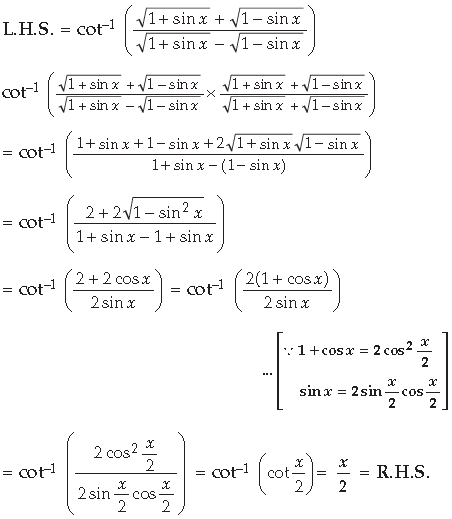
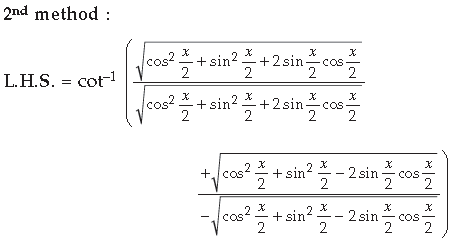
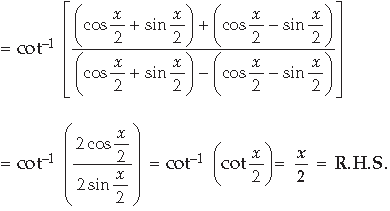
Question. Prove the following :
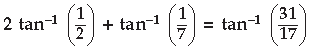
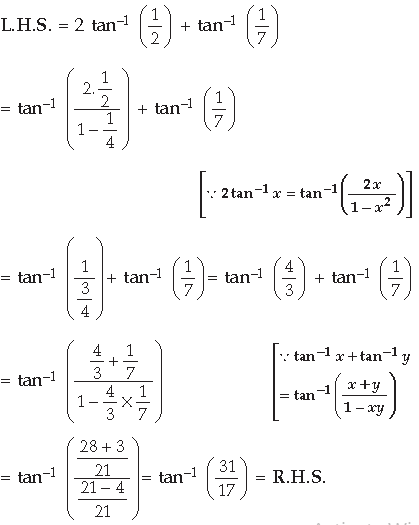
Question. Prove the following :
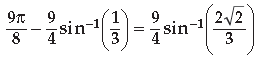
Answer.
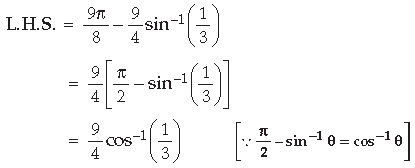
Question.
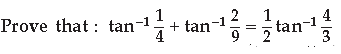
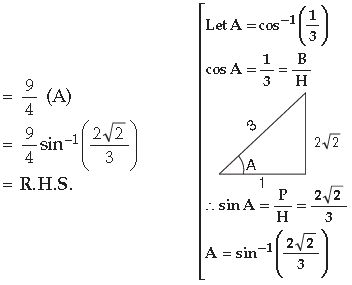
Answer.
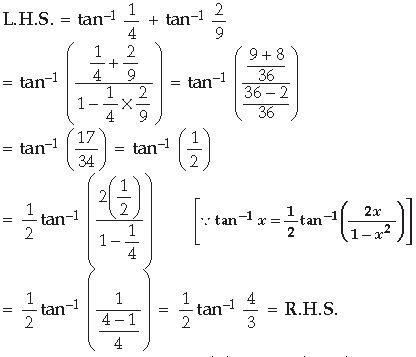
Question.
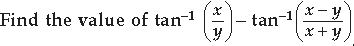
Answer. Ist method
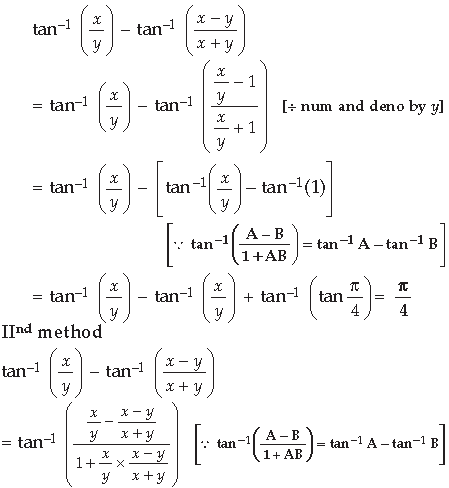
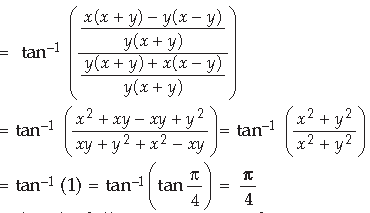
Question. Solve for x :
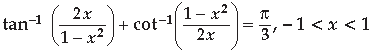
Answer.
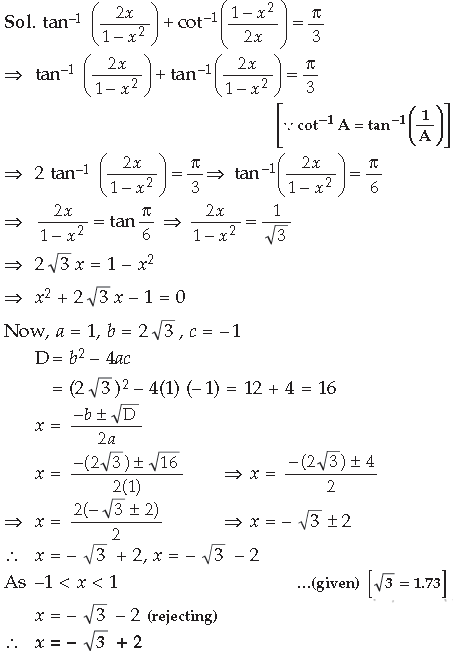
Question. Solve for x : cos(2 sin–1 x) = 1/9, x > 0
Answer. cos(2 sin–1 x) = 1/9
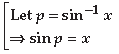
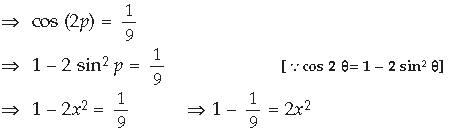
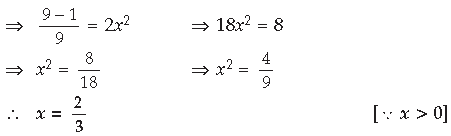
Question. Prove that
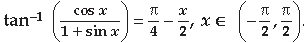
Answer.
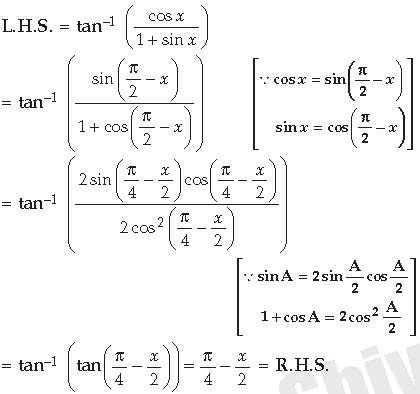
Question. Prove the following :
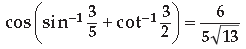
Answer.
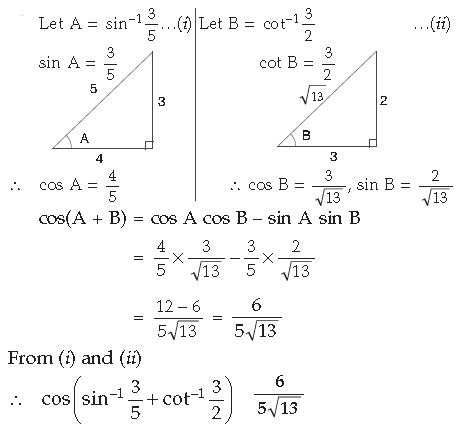
Question.
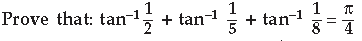
Answer.
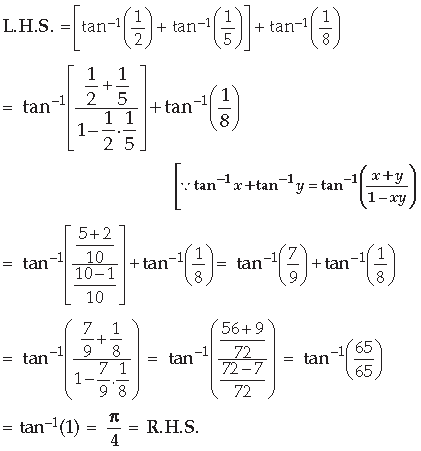
Question.
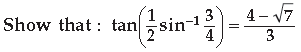
Answer.
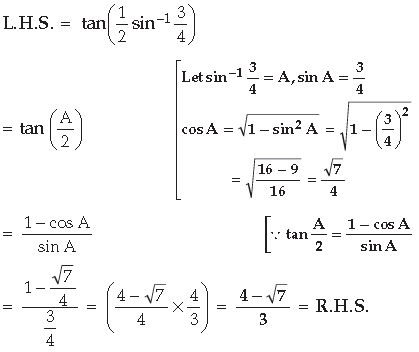
Question. Prove that :
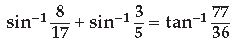
Answer.
Question. Find the value of the following :
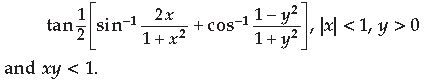
Answer.
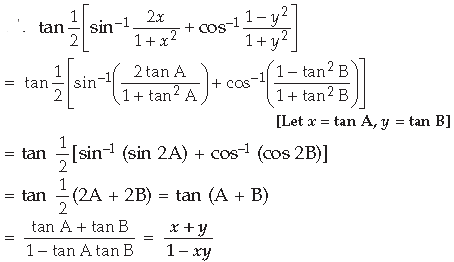
Question. Solve the following equation :
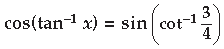
Answer.
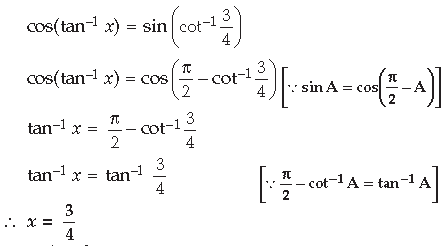
Question. Solve for x :
sin–1 (1 – x) – 2 sin–1 x = π/2
Answer. sin–1 (1 – x) – 2 sin–1 x = π/2
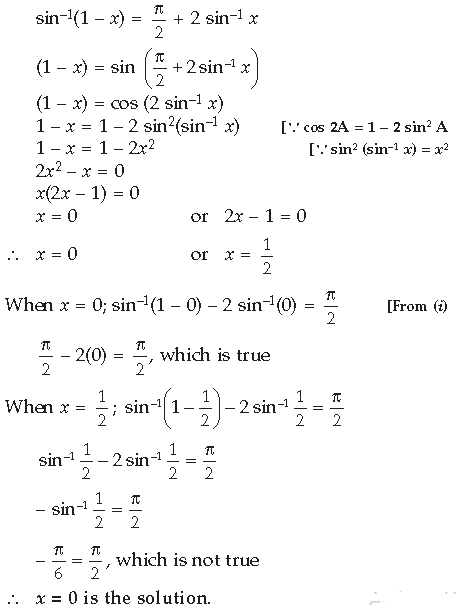
Question. Prove that :
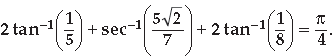
Answer.
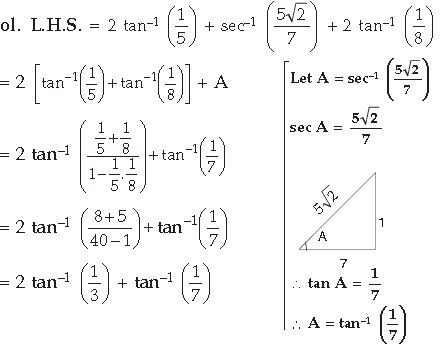
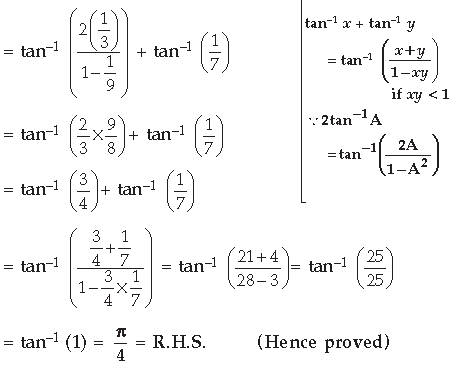
Question.
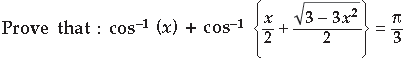
Answer.
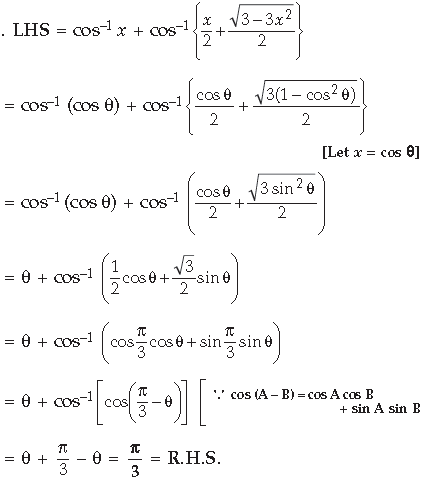
Question.
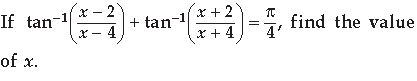
Answer.
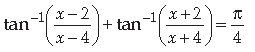
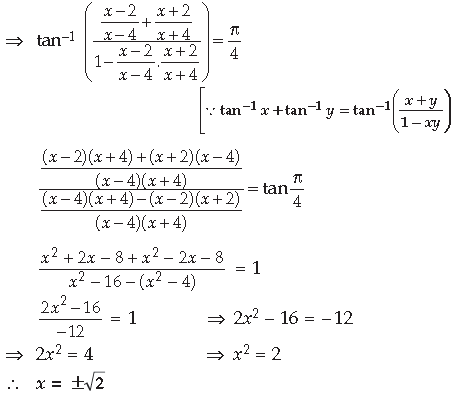
Question. Solve for x :
tan–1 x + 2 cot–1 x = 2π/3
Answer. tan–1 x + 2 cot–1 x = 2π/3
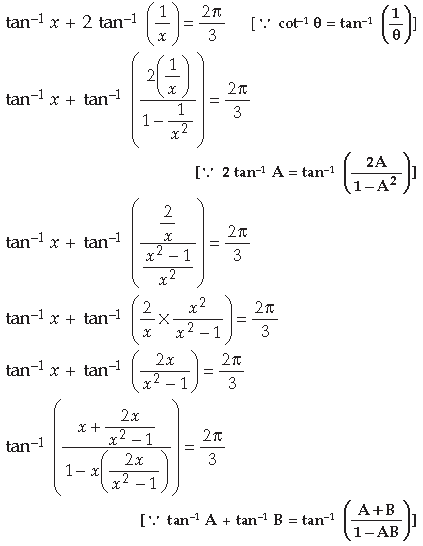
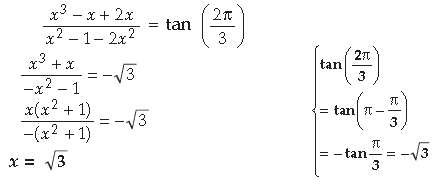
Question.
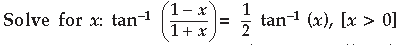
Answer.
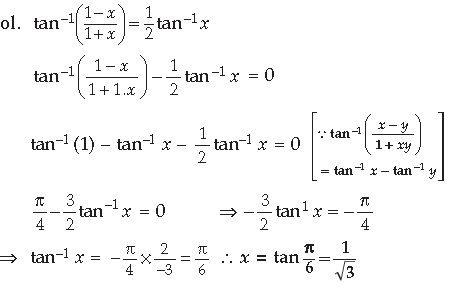
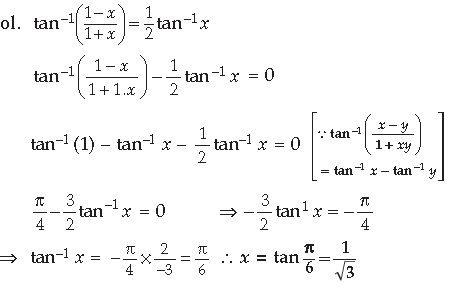
Question. Prove the following :
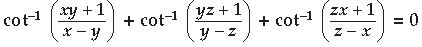
Answer.
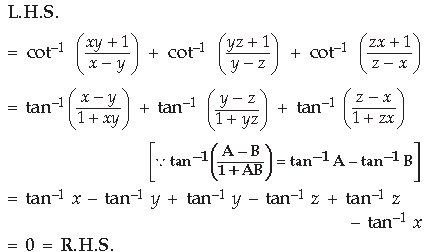
Question. Prove that :
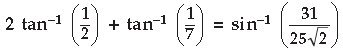
Answer.
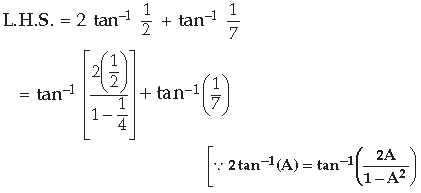
Question. If sin[cot–1 (x + 1)] = cos(tan–1 x), then find x.
Answer. sin[cot–1 (x + 1)] = cos(tan–1 x)
⇒ sin A = cos B