Check the below NCERT MCQ Class 12 Mathematics Chapter 12 Linear Programming with Answers available with PDF free download. MCQ Questions for Class 12 Mathematics with Answers were prepared based on the latest syllabus and examination pattern issued by CBSE, NCERT and KVS. Our teachers have provided below Linear Programming Mathematics Class 12 Mathematics MCQs Questions with answers which will help students to revise and get more marks in exams
Linear Programming Class 12 Mathematics MCQ Questions with Answers
Refer below for MCQ Class 12 Mathematics Chapter 12 Linear Programming with solutions. Solve questions and compare with the answers provided below
Multiple Choice Questions (MCQs)
Question. The region represented by the inequalities x ≥ 6, y ≥ 2, 2x + y ≤ 0, x ≥ 0, y ≥ 0 is
(a) unbounded
(b) a polygon
(c) exterior of a triangle
(d) None of these
Answer
D
Question. The minimum value of Z = 4x + 3y subjected to the constraints 3x + 2y ≥ 160, 5 + 2y ≥ 200, 2y ≥ 80; x, y ≥ 0 is
(a) 220
(b) 300
(c) 230
(d) None of these
Answer
A
Question. The maximum value of f = 4x + 3y subject to constraints x ≥ 0, y ≥ 0, 2x + 3y ≤ 18; x + y ≥ 10 is
(a) 35
(b) 36
(c) 34
(d) none of these
Answer
D
Question. Objective function of a L.P.P. is
(a) a constant
(b) a function to be optimised
(c) a relation between the variables
(d) none of these
Answer
B
Question. Z = 20x1 + 20x2, subject to x1 ≥ 0, x2 ≥ 0, x1 + 2x2 ≥ 8, 3x1 + 2x2 ≥ 15, 5x1 + 2x2 ≥ 20. The minimum value of Z occurs at
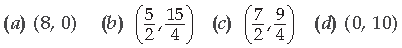
Answer
C
Question. Maximize Z = 11x + 8y, subject to x ≤ 4, y ≤ 6, x ≥ 0, y ≥ 0.
(a) 44 at (4, 2)
(b) 60 at (4, 2)
(c) 62 at (4, 0)
Answer
B
Question. The feasible, region for an LPP is shown shaded in the figure. Let Z = 3x – 4y be the objective function. A minimum of Z occurs at
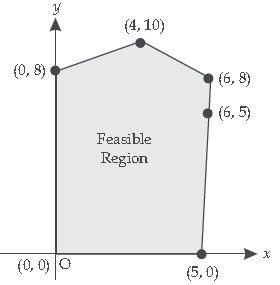
(a) (0, 0)
(b) (0, 8)
(c) (5, 0)
(d) (4, 10)
Answer
B
Question. The optimal value of the objective function is attained at the points
(a) on x-axis
(b) on y-axis
(c) which are corner points of the feasible region
(d) none of these
Answer
C
Question. Z = 7x + y, subject to 5x + y ≥ 5, x + y ≥ 3, x ≥ 0, y ≥ 0. The minimum value of Z occurs at
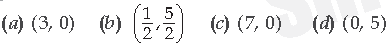
Answer
D
Question. Z = 4x1 + 5x2, subject to 2x1 + x2 ≥ 7, 2x1 + 3x2 ≤ 15, x2 ≤ 3, x1, x2 ≥ 0. The minimum value of Z occurs at
(a) (3.5, 0)
(b) (3, 3)
(c) (7.5, 0)
(d) (2, 3)
Answer
A
Question. Minimize Z = 20x1 + 9x2, subject to x1 ≥ 0, x2 ≥ 0, 2x1 + 2x2 ≥ 36, 6x1 + x2 ≥ 60.
(a) 360 at (18, 0)
(b) 336 at (6, 4)
(c) 540 at (0, 60)
(d) 0 at (0, 0)
Answer
B
Question. Z = 8x + 10y, subject to 2x + y ≥ 1, 2x + 3y ≥ 15, y ≥ 2, x ≥ 0, y ≥ 0. The minimum value of Z occurs at
(a) (4.5, 2)
(b) (1.5, 4)
(c) (0, 7)
(d) (7, 0)
Answer
B
Question. In solving the LPP: “minimize f = 6x + 10y subect to constraints x ≥ 6, y ≥ 2, 2x + y ≥ 10, x ≥ 0, y ≥ 0” redundant constraints are
(a) x ≥ 6, y ≥ 2
(b) 2x + y ≥ 10, x ≥ 0, y ≥ 0
(c) x ≥ 6
(d) none of these
Answer
B
Question. Region represented by x ≥ 0, y ≥ 0 is
(a) first quadrant
(b) second quadrant
(c) third quadrant
(d) fourth quadrant
Answer
A
Question. Maximize Z = 6x + 4y, subject to x ≤ 2, x + y ≤ 3, –2x + y ≤ 1, x ≥ 0, y ≥ 0.
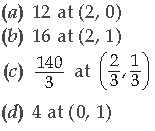
Answer
C
Question. The maximum value of Z = 3x + 2y, subjected to x + 2y ≤ 2, x + 2y ≥ 8; x, y ≥ 0 is
(a) 32
(b) 24
(c) 40
(d) None of these
Answer
D
Question. Maximize Z = 3x + 5y, subject to x + 4y ≤ 24, 3x + 4y ≤ 21, x + y ≤ 9, x ≥ 0, y ≥ 0.
(a) 20 at (1, 0)
(b) 30 at (0, 6)
(c) 37 at (4, 5)
(d) 33 at (6, 3)
Answer
C
Question. Maximize Z = 4x + 6y, subject to 3x + 2y ≤ 12, x + y ≥ 4, x, y ≥ 0.
(a) 16 at (4, 0)
(b) 24 at (0, 4)
(c) 24 at (6, 0)
(d) 36 at (0, 6) [Ans. (d)
Answer
D
Question. The feasible region for an LPP is shown shaded in the following figure. Minimum of Z = 4x + 3y occurs at the point
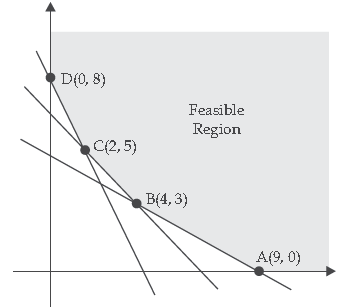
(a) (0, 8)
(b) (2, 5)
(c) (4, 3)
(d) (9, 0) [Ans. (b)
Answer
B
Case Based Questions
1. Linear programming is a method for finding the optimal values (maximum or minimum) of quantities subject to the constraints when relationship is expressed as linear equations or inequations.
Based on the above information, answer the following questions:
Question. The optimal value of the objective function is attained at the points
(a) on X-axis
(b) on Y-axis
(c) which are corner points of the feasible region
(d) none of these
Answer
C
Question. The graph of the inequality 3x + 4y < 12 is
(a) half plane that contains the origin
(b) half plane that neither contains the origin nor the points of the line 3x + 4y = 12.
(c) whole XOY-plane excluding the points on line 3x + 4y = 12
(d) None of these
Answer
D
Question. The feasible region for an LPP is shown in the figure. Let Z = 2x + 5y be the objective function.
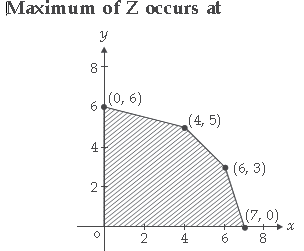
(a) (7, 0)
(b) (6, 3)
(c) (0, 6)
(d) (4, 5)
Answer
D
Question. The corner points of the feasible region determined by the system of linear constraints are (0, 10), (5, 5), (15, 15), (0, 20). Let Z = px + qy, where p, q > 0. Condition on p and q so that the maximum of Z occurs at both the points (15, 15) and (0, 20) is
(a) p = q
(b) p = 2q
(c) q = 2p
(d) q = 3p
Answer
D
Question. The corner points of the feasible determined by the system of linear constraints are (0, 0), (0, 40), (20, 40), (60, 20), (60, 0). The objective function is Z = 4x + 3y.
Compare the quantity in Column A & Column B.
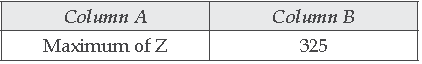
(a) The quantity in column A is greater
(b) The quantity in column B is greater
(c) The two quantities are equal
(d) The relationship cannot be determined on the basis of the information supplied.
Answer
B
2. Deepa rides her car at 25 km/hr. She has to spend ₹2 per km on diesel and if she rides it at a faster speed of 40 km/hr, the diesel cost increases to ₹5 per km. She has ₹100 to spend on diesel. Let she travels x kms with speed 25 km/hr and y kms with speed 40 km/hr. The feasible region for the LPP is shown below:
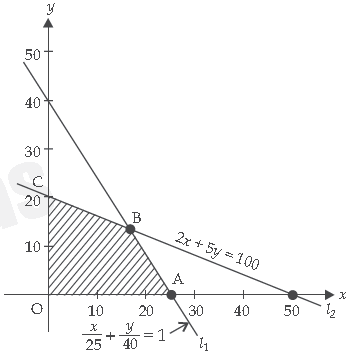
Based on the above information, answer the following questions:
Question. What is the point of intersection of line l1 and l2?
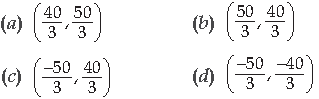
Answer
B
Question. The corner points of the feasible region shown in the above graph are
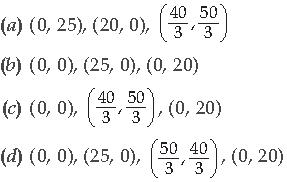
Answer
D
Question.) If Z = x + y be the objective function and max Z = 30. The maximum value occurs at point
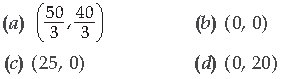
Answer
A
Question. If Z = 6x – 9y be the objective function, then maximum value of Z is
(a) –20
(b) 150
(c) 180
(d) 20
Answer
B
Question. If Z = 6x + 3y be the objective function, then what is the minimum value of Z?
(a) 120
(b) 130
(c) 0
(d) 150
Answer
C
Corner points of the feasible region for an LPP are (0, 3), (5, 0), (6, 8), (0, 8). Let Z = 4x – 6y be the objective function.
Based on the above information, answer the following questions:
Question. The minimum value of Z occurs at
(a) (6, 8)
(b) (5, 0)
(c) (0, 3)
(d) (0, 8)
Answer
D
Question. Maximum value of Z occurs at
(a) (5, 0)
(b) (0, 8)
(c) (0, 3)
(d) (6, 8)
Answer
A
Question. Maximum of Z – Minimum of Z =
(a) 58
(b) 68
(c) 78
(d) 88
Answer
B
Question. The corner points of the feasible region determined by the system of linear inequalities are
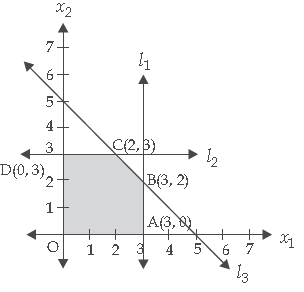
(a) (0, 0), (–3, 0), (3, 2), (2, 3)
(b) (3, 0), (3, 2), (2, 3), (0, –3)
(c) (0, 0), (3, 0), (3, 2), (2, 3), (0, 3)
(d) None of these
Answer
C
Question. The feasible solution of LPP belongs to
(a) first and second quadrant
(b) first and third quadrant
(c) only second quadrant
(d) only first quadrant
Answer
D
Suppose a dealer in rural area wishes to purpose a number of sewing machines. He has only ₹5,760 to invest and has space for at most 20 items for storage.

An electronic sewing machine costs him ₹360 and a manually operated sewing machine ₹240. He can sell an electronic sewing machine at a profit of ₹22 and a manually operated sewing machine at a profit of ₹18.
Based on the above information, answer the following questions:
Question. Let x and y denotes the number of electronic sewing machines and manually operated sewing machines purchased by the dealer. If it is assumed that the dealer purchased atleast one of the given machines, then
(a) x + y ≥ 0
(b) x + y < 0
(c) x + y > 0
(d) x + y ≤ 0
Answer
C
Question. Let the constraints in the given problem is represented by the following inequalities:
x + y ≤ 20
360x + 240y ≤ 5760
x, y ≥ 0
Then which of the following point lie in its feasible region.
(a) (0, 24)
(b) (8, 12)
(c) (20, 2)
(d) None of these
Answer
B
Question. If the objective function of the given problem is maximise z = 22x + 18y, then its optimal value occur at
(a) (0, 0)
(b) (16, 0)
(c) (8, 12)
(d) (0, 20)
Answer
C
Question. Suppose the following shaded region APDO, represent the feasible region corresponding to mathematical formulation of given problem. Then which of the following represent the coordinates of one of its corner points.
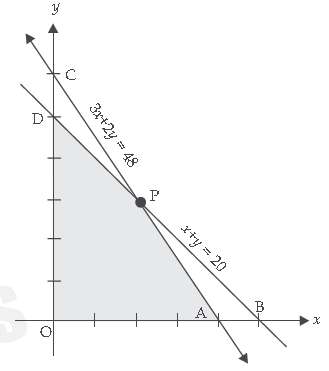
(a) (0, 24)
(b) (12, 8)
(c) (8, 12)
(d) (6, 14)
Answer
C
Question. If an LPP admits optimal solution at two consecutive vertices of a feasible region, then
(a) the required optimal solution is at the midpoint of the line joining two points.
(b) the optimal solution occurs at every point on the line joining these two points.
(c) the LPP under consideration is not solvable.
(d) the LPP under consideration must be reconstructed.
Answer
B
Let R be the feasible region (convex polygon) for a linear programming problem and let Z = ax + by be the objective function. When Z has an optimal value (maximum or minimum), where the variables x and y are subject to constraints described by linear inequalities, this optimal value must occur at a corner point (vertex) of the feasible region.
Based on the given information, answer the following questions:
Question. Objective function of a L.P.P. is
(a) a constant
(b) a function to be optimised
(c) a relation between the variables
(d) none of these
Answer
B
Question. Which of the following statement is correct?
(a) Every LPP has at least one optimal solution
(b) Every LPP has a unique optimal solution.
(c) If an LPP has two optimal solutions, then it has infinitely many solutions.
(d) None of these
Answer
C
Question. In solving the LPP : “minimize f = 6x + 10y subject to constraints x ≥ 6, y ≥ 2, 2x + y ≥ 10, x ≥ 0, y ≥ 0” redundant constraints are
(a) x ≥ 6, y ≥ 2
(b) 2x + y ≥ 10, x ≥ 0, y ≥ 0
(c) x ≥ 6
(d) none of these
Answer
B
Question. The feasible region for a LPP is shown shaded in the figure. Let Z = 3x – 4y be the objective function. Minimum of Z occurs at
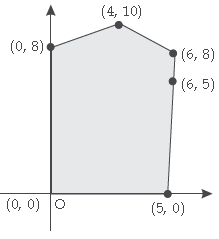
(a) (0, 0)
(b) (0, 8)
(c) (5, 0)
(d) (4, 10)
Answer
B
Question. The feasible region for a LPP is shown shaded in the figure. Let F = 3x – 4y be the objective function. Maximum value of F is
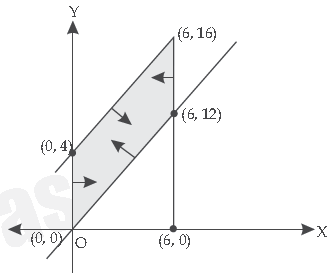
(a) 0
(b) 8
(c) 12
(d) –18
Answer
A

