Check the below NCERT MCQ Class 12 Mathematics Chapter 5 Continuity and Differentiability with Answers available with PDF free download. MCQ Questions for Class 12 Mathematics with Answers were prepared based on the latest syllabus and examination pattern issued by CBSE, NCERT and KVS. Our teachers have provided below Continuity and Differentiability Mathematics Class 12 Mathematics MCQs Questions with answers which will help students to revise and get more marks in exams
Continuity and Differentiability Class 12 Mathematics MCQ Questions with Answers
Refer below for MCQ Class 12 Mathematics Chapter 5 Continuity and Differentiability with solutions. Solve questions and compare with the answers provided below
Question.

Then the value of (gof)’ (0) is
(a) 1
(b) – 1
(c) 0
(d) None of these
Answer
C
Question.

(a) 0
(b) 1
(c) 2
(d) None of these
Answer
C
Question. If f (x ) is differentiable and strictly increasing function, then the value of
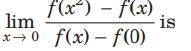
(a) 1
(b) 0
(c) −1
(d) 2
Answer
C
Question.
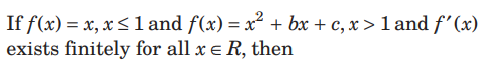
(a) b = −1, c ∈ R
(b) c = 1, b ∈ R
(c) b = 1, c = −1
(d) b = −1, c = 1
Answer
D
Question.
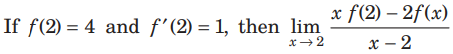
is
equal to
(a) 2
(b) −2
(c) 1
(d) 3
Answer
A
Question.

(a) 16
(b) 8
(c) 4
(d) 2
Answer
A
Question. Let f: R→ R : be a function defined by f (x)= max( x,x3).
The set of all points where f (x) is not differentiable, is
(a) {-1,1}
(b) {-1, 0}
(c) { 0,1 }
(d) {-1,0,1}
Answer
D
Question. f (x)= |x| = is
(a) discontinuous at x = 0
(b) not differentiable at x = 0
(c) differentiable at x = 0
(d) None of these
Answer
C
Question. The function f (x )= |x3| is
(a) differentiable everywhere
(b) continuous but not differentiable at x = 0
(c) not a continuous function
(d) None of the above
Answer
A
Question. If f (x)=
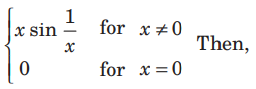
(a) f′(0+) and f′(0–) do not exist
(b) f′(0+) exists but f′(0–) does not exist
(c) f′(0+) =f′(0–)
(d) None of the above
Answer
A
Question. The function f (x)=
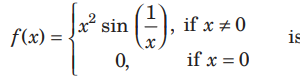
differentiable at x = 0, then f ′(0) is
(a) 1/2
(b) 2
(c) 1
(d) 0
Answer
D
Question. If f (x) =x/1+|x| for x ∈ R, then f ′(0 ) is equal to
(a) 0
(b) 1
(c) 2
(d) 3
Answer
B
Question. The set of points of differentiability of the function
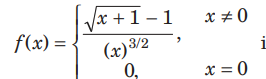
(a) R
(b) [0, ] ∞
(c) (0, ∞]
(d) R − {0}
Answer
C
Question. The number of points of non-differentiability for the function f (x)= | x|+ |cos|+ tan (x+π/4) in the interval (-2,2) is
(a) 1
(b) 2
(c) 3
(d) 4
Answer
C
Question. The function f(x)= |x-1|+|x- 2| is
(a) continuous and differentiable everywhere
(b) continuous at x = 1, 2 but differentiable anywhere
(c) continuous everywhere but not differentiable at x = 1, 2
(d) None of the above
Answer
C
Question. Let f (x)
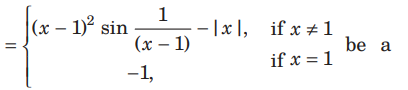
real-valued function. Then, the set of points where f (x ) is not differentiable is
(a) {0 }
(b) {0 ,1}
(c) {1}
(d) null set
Answer
A
Question. Let f (x)
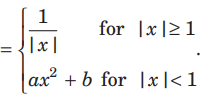
.If f(x) is continuous and differentiable everywhere, then
(a) a = 1/2, b=-3/2
(b) a= – 1/2, b=3/2
(c) a=1, b =-1
(d) a = b =1
Answer
B
Question. The function
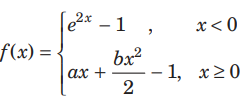
is continuous and differentiable for
(a) a= 1, b=2
, (b) a=2, b=1
(c) a = 2 , any b
(d) any a, b, = 4
Answer
C
Question. For the function
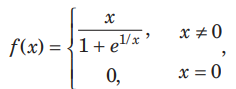
the derivative from the right, f ′(0+)…and the derivative from the left f ′(0–) are
(a) {0,1}
(b) {1,0}
(c) {1,1}
(d) 0 0,
Answer
A
Question. Let f (x)
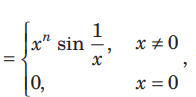
then f (x) is continuous but not differentiable at x = 0, if
(a) n ∈( 0, 1)
(b) n ∈ [1,∞)
(c) (-∞,0)
(d) n = 0
Answer
A
Question. The function y =|sin x| is continuous for any x but it is not differentiable at
(a) x = 0 only
(b) x = π only
(c) x= k π (k is an positive integer) only
(d) x = 0 and x= kπ (k is an integer)
Answer
D
Question.

is continuous at x = 4, then a =
(a) 2
(b) 4
(c) 6
(d) 8
Answer
B
Question. If y = exx , then . dy/dx =
(a) y(1+loge x)
(b) yxx (1+loge x)
(c) yex (1+loge x)
(d) None of these
Answer
B
Question. If x = sin t cos 2t and y = cos t sin 2t, then at t = π/4 , the value of dy/dx is equal to :
(a) – 2
(b) 2
(c) 1/2
(d) – 1/2
Answer
C
Question. If f(x) = 1/1– x , then the points of discontinuity of the function f [ f {f(x)}] are
(a) {0, –1}
(b) {0,1}
(c) {1, –1}
(d) None
Answer
B
Question. Let f : R → R be a function defined by f (x) = max {x, x3}. The set of all points where f (x) is NOT differentiable is
(a) {–1, 1}
(b) {–1, 0}
(c) {0, 1}
(d) {–1, 0, 1}
Answer
D
Question. Match the terms given in column-I with the terms given in column-II and choose the correct option from the codes given below.

Codes
A B C D
(a) 2 3 1 1
(b) 1 2 3 1
(c) 3 1 2 1
(d) 1 3 1 2
Answer
C
Question. The no. of points of discontinuity of the function f (x) = x – [x] in the interval (0, 7) are
(a) 2
(b) 4
(c) 6
(d) 8
Answer
C
Question. If y = 5x.x5, then dy/dx is
(a) 5x (x5 log5 –5x4)
(b) x5 log5 – 5x4
(c) x5 log5 + 5x4x4
(d) 5x (x5 log5 + 5x4)
Answer
D
Question. The relationship between a and b, so that the function f defined by

is continuous at x = 3 , is
(a) a = b + 2/3
(b) a – b = 3/2
(c) a + b = 2/3
(d) a + b = 2
Answer
A
Case Based Questions
Let f(x) be a real valued function, then its

Question. L.H.D. of f(x) at x = 1 is
(a) 1
(b) –1
(c) 0
(d) 2
Answer
B
Question. R.H.D. of f(x) at x = 1 is
(a) 1
(b) –1
(c) 0
(d) 2
Answer
B
Question. Find the value of f(2)
(a) 1
(b) 2
(c) 3
(d) –1
Answer
D
Question. f(x) is non-differentiable at
(a) x = 1
(b) x = 2
(c) x = 3
(d) x = 4
Answer
C
Question. The value of f′(–1) is
(a) 2
(b) 1
(c) –2
(d) –1
Answer
C
Let x = f(t) and y = g(t) be parametric forms with
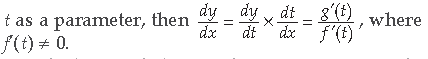
On the basis of above information, answer the following questions:
Question.
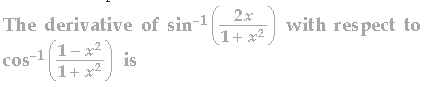
(a) –1
(b) 1
(c) 2
(d) 4
Answer
B
Question. The derivative of f(tan x) w.r.t. g(sec x) at x = π/4 , where f′(1) = 2 and g’(√2 ) = 4, is
(a) 1/√2
(b) √2
(c) 1
(d) 0
Answer
A
Question. The derivative of cos–1(2x2 – 1) w.r.t cos–1x is
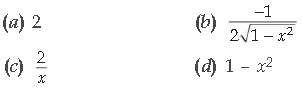
Answer
A
Question. The derivative of ex3 with respect to log x is
(a) ex2
(b) 3x22ex3
(c) 3x3ex3
(d) 3x2ex3+ 3x
Answer
C
Question.
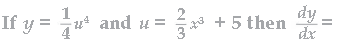
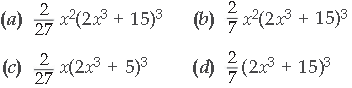
Answer
A
Let f : A → B and g : B → C be two functions defined on non-empty sets A, B, C then gof : A → C be is called the composition of f and g defined as, gof(x) = g[f(x)] ∀ x ∈ A
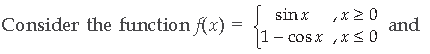
g(x) = ex and then answer the following questions:
Question.
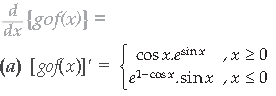
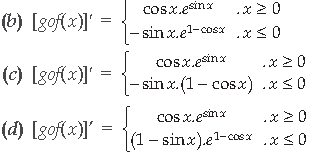
Answer
A
Question. The function gof(x) is defined as
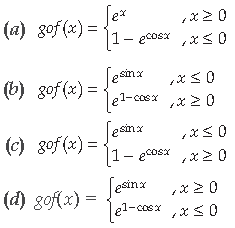
Answer
D
Question. L.H.D. of gof(x) at x = 0 is
(a) 0
(b) 1
(c) –1
(d) 2
Answer
A
Question. R.H.D. of gof(x) at x = 0 is
(a) 0
(b) 1
(c) –1
(d) 2
Answer
B
Question. The value of f′(x) at x = π/4 is
(a) 1/9
(b) 1/√2
(c) 1/2
(d) not defined
Answer
B
The function f(x) will be discontinuous at x = a if f(x) has
• Discontinuity of first kind:
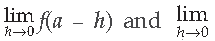
(a + h) both exist but are not equal. It is also known as irremovable discontinuity.
• Discontinuity of second kind: if none of the limits
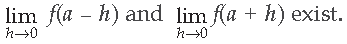
• Removable discontinuity:
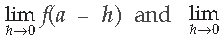
f(a + h) both exist and equal but not equal to f(a).
Based on the above information, answer the following questions:
Question.
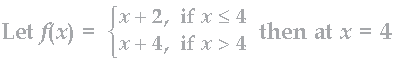
(a) f is continuous
(b) f has removable discontinuity
(c) f has irremovable discontinuity
(d) none of these
Answer
C
Question.
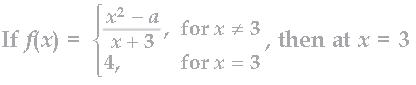
(a) f has removable discontinuity
(b) f is continuous
(c) f has irremovable discontinuity
(d) none of these
Answer
A
Question.
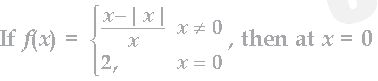
(a) f is continuous
(b) f has removable discontinuity
(c) f has irremovable discontinuity
(d) none of these
Answer
C
Question. Consider the function f(x) defined as
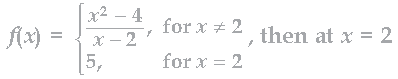
(a) f has removable discontinuity
(b) f has irremovable discontinuity
(c) f is continuous
(d) f is continuous if f(2) = 3
Answer
A
Question.
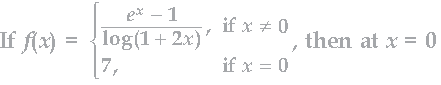
(a) f is continuous if f(0) = 2
(b) f is continuous
(c) f has irremovable discontinuity
(d) f has removable discontinuity
Answer
D
If a real valued function f(x) is finitely derivable at any point of its domain. It is necessarily continuous at that point. But its converse need not be true. For example, Every polynomial, constant function are both continuous as well as differentiable and inverse trigonometric functions are continuous and differentiable in its domains etc.
Based on the above information, answer the following questions:
Question. If f(x) =|x – 1|, x ∈ R, then at x = 1.
(a) f(x) is not continuous
(b) f(x) is continuous but not differentiable
(c) f(x) is continuous and differentiable
(d) none of these
Answer
B
Question.
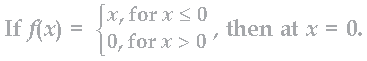
(a) f(x) is differentiable and continuous
(b) f(x) is neither continuous nor differentiable
(c) f(x) is continuous but not differentiable
(d) None of these
Answer
A
Question. If f(x) = |sin x|, then which of the following is true?
(a) f(x) is continuous and differentiable at x = 0
(b) f(x) is discontinuous at x = 0
(c) f(x) is continuous at x = 0 but not differentiable
(d) f(x) is differentiable but not continuous at x = π/2
Answer
B
Question. f(x) = x3 is
(a) continuous but not differentiable at x = 3
(b) continuous and differentiable at x = 3
(c) neither continuous nor differentiable at x = 3
(d) none of these
Answer
B
Question. If f(x) = sin–1x, 1 ≤ x ≤ 1, then
(a) f(x) is both continuous and differentiable
(b) f(x) is neither continuous nor differentiable
(c) f(x) is continuous but not differentiable
(d) None of these
Answer
A
A potter made a mud vessel, where the shape of the pot is based on f(x) = |x – 3| + |x – 2|, where f(x) represents the height of the pot.

Question. Will the slope vary with x value?
(a) Yes
(b) No
(c) Sometimes
(b) Never
Answer
A
Question. When x > 4, What will be the height in terms ofx?
(a) x – 2
(b) x – 3
(c) 2x – 5
(d) 5 – 2x
Answer
C
Question. When the x value lies between (2, 3) then the function is
(a) 2x – 5
(b) 5 – 2x
(c) 1
(d) 5
Answer
C
Question. What is f ’(x) at x = 3
(a) 2
(b) –2
(c) Function is not differentiable
(d) 1
Answer
C
Question. If the potter is trying to make a pot using the function f(x) = [x], will he get a pot or not? Why?
(a) Yes, because it is a continuous function
(b) Yes, because it is not continuous
(c) No, because it is a continuous function
(d) No, because it is not continuous
Answer
C
