Permutation Combination and Probability MCQ Questions

Please go through Permutation Combination and Probability MCQ with Answers for Environment Economics provided below. Students should have strong knowledge about the Permutation Combination and Probability as in various competition exams, MCQ questions are asked from this topic. We have provided below the biggest collection of Permutation Combination and Probability MCQ with Answers. These Business Statistics MCQ and objective questions will improve your performance in exams and help you to get good scores.

Multiple Choice Questions for Permutation Combination and Probability with Answers

Question. An intelligence agency forms a code of two distinct digits selected from 0, 1, 2,……, 9 such that the first digit of the code is non zero. The code, handwritten on the slip, can create confusion, when read upside down for example the code 91 can be read as 16. How many codes are there for which no such confusion can arise?
(a) 80
(b) 78
(c) 71
(d) 69

Question. The set of all possible outcomes of a random experiment is known as
(a) Permutation
(b) Combination
(c) Probability
(d) Sample space

Question. A doctor is to visit a patient. The probability that he will come by car taxi scooter or by other means of transport are 0.3, 0.2, 0.1 and 0.4. The probabilities that he will be late are 1/4, 1/3 and ½, if he comes by car taxi and scooter. But if he comes by other means of transport he will not be late. When he arrives he is late. Therefore the probability that he comes by car are
(a) 1/2
(b) 0
(c) 1/4
(d) 1

Question. What is the chance that a leap year selected at random will contain 53 Sundays?
(a) 2/7
(b) 3/7
(c) 1/7
(d) 5/7

Question. A box contains 6 red balls, 7 green balls and 5 blue balls. Each ball is of different size. The probability that the red ball selected is the smallest red ball is
(a) 1/8
(b) 1/3
(c) 1/6
(d) 2/3

Question. Boxes numbered 1,2,3,4 and 5 are kept in a row, and they which are to be filled with either a red ball or a blue ball, such that no two adjacent boxes can be filled with blue balls. Then how many different arrangements are possible, given that all balls of given colour are exactly identical in all respect?
(a) 8
(b) 10
(c) 154
(d) 22

Question. There are three cities A, B and C. Each of these cities is connected with the other two cities by at least one direct road. If a traveler wants to go from one city (origin) to another city (destination), she can do so either by traversing a road connecting the two cities directly, or by traversing two roads, the first connecting the origin to the third city and the second connecting the third city to the destination. In all, there are 33routes from A to B (including those via C), Similarly, there are 23 routes from B to C (including those via A). How many roads are there from A to C directly?
(a) 6
(b) 3
(c) 5
(d) 10

Question. Three of the 6 vertices if a regular hexagon are chosen at random. The probability that the triangle with these vertices is equilateral is
(a) 1/10
(b) 4/10
(c) 3/10
(d) 1/5

Question. What is the value of n(P(P(P(ø))))
(a) 3 elements
(b) 4 elements
(c) 8 elements
(d) 5 elements

Question. One red flag, three white flags and two blue flags are arranged in line such that
i) No two adjacent flags are of the same colour.
ii) The flags at the two ends of the line are of different colours.
In how many different ways the flag be arranged?
(a) 6
(b) 4
(c) 10
(d) 2

Question. Each of the 11 letters A. H, I, M, O, T, U, V, W, X and Z appears same hen looked at in the mirror. They are called symmetric letters. Other letters in the alphabet are asymmetric letters. How many four letter computer passwords can be formed using only the symmetric letters ( no repetition allowed)
(a) 7920
(b) 330
(c) 146.40
(d) 419430

Question. A card is drawn from a well shuffled pack of playing cards. Find the probability that it is either a diamond or a king
(a) 4/26
(b) 4/13
(c) 17/52
(d) 16/13

Question. A five digit number is formed using digits 1,3 5, 7 and 9without repeating any one of them. What is the sum of all such possible numbers?
(a) 6666600
(b) 6666660
(c) 6666666
(d) None of these

Question. 139 persons have signed for an elimination tournament. All players are to be paired up for the first round, but because 139 is an odd number one player gets a bye, which promotes him to the second round, without actually playing in the first round. The pairing continues on the next round, with a bye to any player left over. If the schedule is planned so that a minimum number of matches is required to determine the champion, the number of matches which must be played is
(a) 136
(b) 137
(c) 138
(d) 139

Question. Let A and B be the two possible outcomes of an experiment and suppose P(A) = 0.4 P(AUB) =0.7 and P(B) =p. For what choice of p are A and B mutually exclusive?
(a) 0.5
(b) 0.2
(c) 0.3
(d) 0.6

Question. A card is drawn at random from a well shuffled pack of cards. The probability that it is heart or a queen is
(a) 4/13
(b) 11/52
(c) ½
(d) 1/52

Question. If P(A) =1/4, P(B) =2/5 and P(AUB) =1/2 find P(A^c U B^c ), where A and B are two non mutually exclusive events connected with a random experiment E and Ac is the complement event of A.
(a) 0.85
(b) 0.58
(c) 0.80
(d) 0.50

Question. The result of an examination given to a class on three papers A, B and C are 40% failed in paper A, 30% failed in B, 25% failed in paper C, 15% failed in paper A and B both. 12% failed in B and C both, 10% failed in A and C both, 3% failed in A, B and C. What is the probability of a randomly selected candidates passing in all three papers?
(a) 0.6
(b) 0.39
(c) 0.56
(d) 0.42

Question. For a scholarship, at the most n candidates out of 2n + 1 can be selected. If the number of different ways of selection of at least one candidate is 63, the maximum number of candidates that can be selected for the scholarship is
(a) 3
(b) 4
(c) 6
(d) 5

Question. Ten points are marked on a straight line and 11 points are marked on another straight line. How many triangles can be constructed with vertices from among the above points?
(a) 495
(b) 550
(c) 1045
(d) 2475

Question. The figure below shows the network of cities A, B, C, D, E and F. The arrows show the permissible direction of travel. What is the number of distinct paths from A to F?

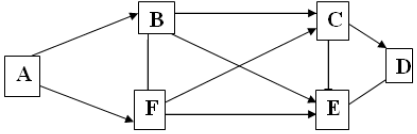
(a) 9
(b) 10
(c) 11
d) None of these

Question. If P(A) = 0.3 P(B) = 0.2 and P(C) =0.1 and A, B, C are independent events the probability of occurrence of at least one of the three events A,B, C is
(a) 0.41
(b) 0.37
(c) 0.496
(d) 0.387

Question. Probability that a man will be alive 25 years hence is 0.3 and the probability that his wife will be alive 25 years hence is 0.4. Find the probability that 25 years hence only the man will be alive will be
(a) 0.12
(b) 0.18
(c) 0.28
(d) 0.42

Question. A box of nine golf gloves contains two left-handed and seven right handed gloves. If three gloves are selected without replacement, what is the probability that all of them are left handed?
(a) 1
(b) 0
(c) 7/18
(d) 49/81

Question. A speaks the truth 3 times out of 4, and B 7 times out of 10; They both assert that a white ball has been drawn from a bag containing 6 balls of different colour. The truth in the assertion is
(a) 35/36
(b) 36/43
(c) 25/36
(d) 63/43

Question. There are V lines parallel to the X axis and W lines parallel to the Y axis. How many rectangles can be formed with the intersection of these lines?
(a) ^vP₂ .^wP₂
(b) ^vC₂ . ^wC₂
(c) ^vwC₂
(d) None of these

Question. From 4 men and 4 women a committee of 5 is to be formed. Find the number of ways of doing so if the committee consists of a president, a vice president and three secretaries?
(a) 720
(b) 450
(c) 1120
(d) None of these

Question. Three urns are given, each containing red and white balls. Urn 1: 6 red balls and 4 white, Urn 2: 2 red and 6 white, Urn3: 1 red and 8 white. An urn is chosen at random and a ball is drawn from this urn. The ball is red. The probability that the urn chosen was urn 1 is
(a) 196/173
(b) 173/196
(c) 173/198
(d) 198/173

Question. Out of all the 2-digit integers between 1 and 200, a 2- digit number has to be selected at random. What is the probability that the selected number is not divisible by 7?
(a) 11/90
(b) 33/90
(c) 55/90
(d) 77/90

Question. A piece of electronic equipment has two essential parts A and B. In the past, part A failed 30% of the times, part B failed 20% of the times and both failed simultaneously 5% of the times. Assuming that both parts must operate to enable the equipment to function, the probability that the equipment will function is
(a) 0.1
(b) 0.52
(c) 0.55
(d) 0.15

Question. In a certain college, the students engage in sports in the following proportion Football (F): 60% of all students Basketball (B): 50% of all students. Both football and basketball: 30% of all students. If a student is selected at random the probability that he will play neither sports is
(a) 0.8
(b) 0.10
(c) 0.7
(d) 0.20

Question. Amarnath appears in an exam that has 4 subjects. The chance he passes an individual subject’s test is 0.8. The probability that he will pass in at least one of the subjects is
(a) 0.99984
(b) 0.9984
(c) 0.0004
(d) None of these

Question. In how many ways can 10 identical presents be distributed among 6 children so that each child gets at least one present ?
(a) ¹⁵C₆
(b) ¹⁶C₆
(c) ⁹C₅
(d) 6¹⁰

Question. There are 6 pups and 4 cats. In how many can they be seated in a row so that no cats sit together:
(a) 6 ! 6 X 6 ! 6
(b) 10!/4!6!
(c) 6! X ⁷P4
(d) 6!7!

Question. Suppose it is 11 to 5 against a person who is now 38 years of age living till he is 73 and 5 to 3against B, now 43 living till he is 78. The chance that at least one of these persons will be alive 35 years hence is
(a) 0.47
(b) 0.57
(c) 0.37
(d) 0.67

Question. A box contains 2 tennis , 3 cricket and 4 squash balls. Three balls are drawn in succession with replacement. What is the probability that all are cricket balls:
(a) 1/27
(b) 2/27
(c) 3/27
(d) 1/9

Question. In a garden 40% of the flowers are roses and the rest are carnations. If 25% of the roses and 10% of the carnations are red the probability that a red flower selected at random is a rose is
(a) 5/8
(b) 2/8
(c) 7/8
(d) 3/8

Question. The problem in Mathematics is given to three students A, B and C whose chances of solving it are 1/3, 1/4 and 1/2. The probability that the problem will be solved is
(a) 1/12
(b) 3/4
(c) 7/12
(d) None

Question. A lady declares that by taking a cup of tea, she can discriminate whether the milk or tea infusion was added to the cup. It is proposed to test this assertion by means of an experiment with 12 cups of tea, 6 made in one way and 6 in the other, and presenting them to the lady for judgement in a random order. The probability that on the null hypothesis that the lady has no discrimination power, she would judge correctly all the 12 cups, it being known to her that 6 are of each kind would be
(a) 924
(b) 1/925
(c) 1/924
(d) 925

Question. A restaurant serves two special dishes A and B to its customers consisting of 60% men and 40% women. 80% of men order dish A and the rest B. 70% of women order B and the rest A. In what ratio of A to B should the restaurant prepare the two dishes?
(a) 3:2
(b) 2:3
(c) 1:2
(d) 2:1