Students can read the important questions given below for Surface areas and Volumes Class 9 Mathematics . All Surface areas and Volumes Class 9 Notes and questions with solutions have been prepared based on the latest syllabus and examination guidelines issued by CBSE, NCERT and KVS. You should read all notes provided by us and Class 9 Mathematics Important Questions provided for all chapters to get better marks in examinations. Mathematics Question Bank Class 9 is available on our website for free download in PDF.
Important Questions of Surface areas and Volumes Class 9
Very Short Answer Type Questions:
Question. A solid cube is cut into two cuboids of equal volumes. Find the total surface area of one of the cuboids.
Ans. Let edge of the solid cube be a cm.
Then, dimensions of each of the cuboids will be a cm, a cm and a/2 cm.
∴ Total surface area of one of the cuboids
= 2( a × a + a × a/2 + a/2 × a) = 2( a2 + a2/2 + a2/2) = 4a2 cm2
Question. A metal sheet 27 cm long, 8 cm broad and 1 cm thick is melted and recast into a cube. Then find the volume of cube formed.
Ans.Volume of sheet = (27 × 8 × 1) cm3
= 216 cm3
Volume of cube formed = 216 cm3
Question. The radii of the bases of a cylinder and a cone are in the ratio 3 : 4 and their heights are in the ratio 2 : 3. Then, find the ratio of their volumes.
Ans. Let the radii of the bases of a cylinder and a cone be 3x and 4x respectively and let their heights be 2y and 3y respectively.
∴ Ratio of their volumes = π × (3x)2 × 2y / 1/3π × (4x)2× 3y = 9/8 or 9:8
Question. Area of base of a solid hemisphere is 81p sq. cm. Then find its volume.
Ans. Let r be the radius of the hemisphere.
∴ πr2 = 81π ⇒ r2 = 81 ⇒ r = 9 cm.
Volume of hemisphere = 2/3πr3 = 2/3π(9)3 = 486π cm3
Question. The dimensions of a cinema hall are 120 m, 50 m, 30 m. Then find the volume of hall.
Ans. Length(l) = 120 m, Breadth(b) = 50 m, Height (h) = 30 m
Since the shape of cinema hall is of cuboid
∴ Volume of hall = l × b × h
= 120 × 50 × 30 m3 = 180000 m3
Short Answer Type Questions:
Question. Three equal cubes are placed adjacently in a row. Find the ratio of the total surface area of the resulting cuboid to that of the sum of the surface areas of three cubes.
Ans. Let the side of each cube be a units.
∴ TSA of 1 cube = 6a2 sq. units
⇒ TSA of 3 cubes = 3 × 6a2 = 18a2 sq. units
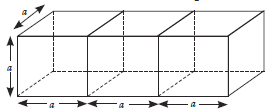
Now length, breadth and height of the resulting cuboid is 3a units, a units and a units respectively.
∴ TSA of cuboid = 2(lb + bh + hl)
= 2(3a × a + a × a + a × 3a)
=2(3a2 + a2 + 3a2) = 2 × 7a2 = 14a2 sq. units.
So, required ratio = 14a2/18a2 = 7/9 or : 7:9
Question. Find the diameter of the sphere, whose total surface area is 616 cm2.
Ans. Let r be the radius of the sphere.
Total surface area of sphere = 4πr2
⇒ 616 = 4 × 22/7 × r2 ⇒ r2 = 616 × 7/4×22 = 49 ⇒ r = 7 cm
∴ Diameter = 2r = 2 × 7 = 14 cm
Question. A cuboidal oil tin box is 4 m by 2 m by 0.75 m. Find the cost of the tin sheet required for making 20 such tin boxes, if the cost of tin sheet is Rs 20 per square metre.
Ans. Length, l = 4 m, breadth, b = 2 m and height, h = 0.75 m
Surface area of one tin box = 2(lb + bh + hl)
= 2 (4 × 2 + 2 × 0.75 + 0.75 × 4)
= 2 (8 + 1.5 + 3) = 2 × 12.5 = 25 m2
∴ Surface area of 20 such tin boxes = (20 × 25) m2
= 500 m2
Now, cost of 1 square metre of tin sheet = Rs 20
∴ Cost of 500 m2 of tin sheet = Rs(20 × 500) = Rs 10000
Question. If volume and surface area of a sphere is numerically equal then find its radius (in units).
Ans. Let r be the radius of the sphere.
∴ Volume of sphere = Surface area of sphere
∴ 4/3πr3 = 4πr2 ⇒ r3/r2 = 3 ⇒ r = units
Question. The total cost of making a solid spherical ball is Rs 67914 at the rate of Rs 14 per cubic metre. Find the radius of this ball.
Ans. Volume of spherical ball = Total cost/Cost of 1m3
⇒ 4/3πr3 = 67914/14 ⇒ 4/3 × 22/7 × r3 = 67914/14
⇒ r3 = 101871/88 ⇒ r3 = 1157.625 ⇒ r = 10.5 m
Question. The external diameter of an iron pipe is 35 cm and its length is 30 cm. If the thickness of the pipe is 2.5 cm, find the curved surface area of the pipe.
Ans. Length of the pipe, h = 30 cm
External radius of the pipe, R = 35/2 cm = 17.5 cm
∴ Thickness of the pipe = 2.5 cm
∴ Internal radius of the pipe, r = (17.5 – 2.5) cm
= 15 cm
Now, curved surface area of the pipe = External curved surface area + Internal curved surface area
= 2πRh + 2πrh = 2πh (R + r)
= 2 × 22/7 × 30(17.5 + 15) = 2 × 22/7 × 30 × 32.5
= 42900/7 = 6128.57 cm2
Question. The length and breadth of a rectangular solid are respectively 35 cm and 20 cm. If its volume is 7000 cm3, then find its height (in cm).
Ans. Let h be the height of the solid.
∴ Volume of cuboid = l × b × h
⇒ 7000 = 35 × 20 × h ⇒ h = 7000/700 = 10 cm
Question. A room is 16 m long, 9 m wide and 3 m high. It has two doors, each of dimensions (2 m × 2.5 m)and three windows, each of dimensions (1.6 m × 75 cm). Find the cost of distempering the walls of the room from inside at the rate of Rs 8 per sq. metre.
Ans. Given, length (l) = 16 m, breadth (b) = 9 m and height (h) = 3 m
∴ Area of 4 walls of the room = 2(l + b) × h
= 2(16 + 9) × 3 = 150 m2.
Area of 2 doors = 2 × (2 × 2.5) = 10 m2
Area of 3 windows = 3 × (1.6 × 75/100) = 3.6 m2.
Area not to be distempered = 10 + 3.6 = 13.6 m2
Area to be distempered = 150 – 13.6 = 136.4 m2
Cost of distempering the walls = Rs (136.4 × 8) = Rs 1091.20
Question. Three cubes each of edge 5 cm are joined end to end. Find the surface area of the resulting cuboid.
Ans.When three cubes are joined end to end, we get a cuboid such that Length of the resulting cuboid, l = 5 cm + 5 cm + 5 cm = 15 cm
Breadth of resulting cuboid, b = 5 cm
Height of the resulting cuboid, h = 5 cm
Surface area of the cuboid = 2 (lb + bh + hl)
= 2 (15 × 5 + 5 × 5 + 5 × 15) cm2
= 2 (75 + 25 + 75) cm2 = 350 cm2
Question. How many spherical balls of diameter 1 cm can be made from an iron ball of diameter 6 cm?
Ans. Volume of iron ball having diameter, 6 cm
= 4/3π(6/2)3 =4/3π(3)3
Volume of small ball of diameter, 1 cm = 4/3π(1/2)3
∴ Number of balls = 4/3π(3)3/4/3π(1/2)3 = 27 × 8 = 216
Question. The length of a cold storage is three times its breadth. Its height is 5 m. The area of its four walls (including doors) is 256 m2. Find its volume.
Ans. Let length, breadth and height of the cold storage be l, b and h respectively.
Then, l = 3b and h = 5 m.
Now, area of four walls = 256 m2
⇒ 2 (l + b)h = 256 ⇒ 2 (3b + b) × 5 = 256
⇒ 40b = 256 ⇒ b = 6.4 metres
∴ l = 3b = 3 × 6.4 = 19.2 m
Volume of the cold storage = l × b × h
= (19.2 × 6.4 × 5) m3 = 614.4 m3
Question. The curved surface area of a cone is 154 cm2. If its radius is x cm and slant height is 7 cm. Find the value of 20x.
Ans. We have, curved surface area = 154 cm2
⇒ πrl = 154 ⇒ r = 154 × 7/22 × 7 = 7
Now, r = x cm = 7 cm.
∴ x = 7 ⇒ 20x = 20 × 7 = 140
Long Answer Type Questions
Question. A sector of a circle of radius 15 cm and central angle of 120°. It is rolled up and the two bounding radii are joined together to form a cone of radius 5 cm. Find :
(i) the volume of the cone.
(ii) the total surface area of the cone.
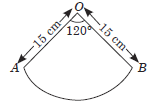
Ans. (i) The slant height of the cone = Radius of the given sector of a circle = 15 cm.
Now, let h be the height of the cone. Then,
h = √l2 − r2 [where l = slant height, r = radius of cone]
= √(15)2 − (5)2 = √225 − 25 = √200 = 10√2 cm
∴ Volume of the cone = 1/3πr2h
= 1/3π(5)2 × 10√2 = 1/3 × 22/7 × 25 ×10 × √2 = 369.29 cm
(ii) Total surface area of the cone = πr(r + l)
= 22 × 5(5 + 15) = 22/7 × 5 × 20 = 314.29 cm2
Question. Coins of same size (say 10 rupee coin) are placed one above the other and a cylindrical block is obtained. The volume of this block is 67.76 cm3. Find the number of coins arranged in the block, if thickness of each coin is 2 mm and radius of each coin is 1.4 cm.
Ans. Let h be the height of cylindrical block and n be the number of coins used to obtain it.
Volume of block = πr2h ⇒ 67.76 = 22/7× 1.4 × 1.4 × h
⇒ h = 67.76 × 7/22 × 1.4 × 1.4 =11cm = 110mm [∴ 1 cm = 10 mm]
Now, n × thickness of a coin = height of block
⇒ n × 2 = 110 ⇒ n = 55
Question. A plot of land is in the form of rectangle has dimension 240 m × 180 m. A drainlet 10 m wideis dug around it (on the outside) and the earth dug out is evenly sπread out over the plot increasing its surface level by 25 cm. Find the depth of the drainlet.
Ans. Volume of earth dug out = l × b × h
= 240 × 180 × 25/100 m3 = 10800 m3
Let the depth of the drainlet be x m.
∴ Volume of drainlet = 2[260 × 10 × x] + 2[180 × 10 × x]
= 8800 x m3
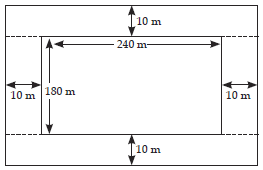
Now, volume of earth dug out = Volume of drainlet
⇒ 10800 = 8800x ⇒ x = 10800/8800 = 1.23 m (apπrox.)



