Check the below NCERT MCQ Class 12 Mathematics Chapter 4 Determinants with Answers available with PDF free download. MCQ Questions for Class 12 Mathematics with Answers were prepared based on the latest syllabus and examination pattern issued by CBSE, NCERT and KVS. Our teachers have provided below Determinants Mathematics Class 12 Mathematics MCQs Questions with answers which will help students to revise and get more marks in exams
Determinants Class 12 Mathematics MCQ Questions with Answers
Refer below for MCQ Class 12 Mathematics Chapter 4 Determinants with solutions. Solve questions and compare with the answers provided below
Question. The minor of the element a11 in the determinant
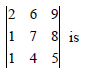
(a) 0
(b) 3
(c) 5
(d) 7
Answer
B
Question. Area of the triangle whose vertices are (a, b + c), (b, c + a) and (c, a + b), is
(a) 2 sq units
(b) 3 sq units
(c) 0 sq unit
(d) None of these
Answer
C
Question. Given : 2x – y – 4z = 2, x – 2y – z = – 4, x + y + λz = 4, then the value of λ such that the given system of equation has NO solution, is
(a) 3
(b) 1
(c) 0
(d) – 3
Answer
D
Question. In how many ways, the determinant of order 3 can be expanded?
(a) 5
(b) 4
(c) 3
(d) 6
Answer
D
Question. If Aij denotes the cofactor of the element aij of the determinant
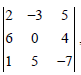
then value of a11A31 + a13A32 + a13A33 is
(a) 0
(b) 5
(c) 10
(d) – 5
Answer
A
Question.
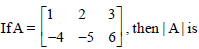
(a) 2
(b) 0
(c) –2
(d) Does not exist
Answer
D
Question.
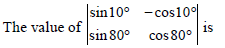
(a) –1
(b) 1
(c) 2
(d) 0
Answer
B
Question. If p, q, r are in A.P., then the value of
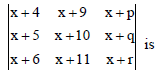
(a) x + 15
(b) x + 20
(c) x + p + q + r
(d) None of these
Answer
D
Question.
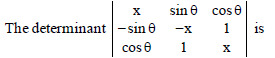
(a) independent of θ only
(b) independent of x only
(c) independent of both θ and x
(d) None of the above
Answer
A
Question.

(a) 2
(b) 4
(c) 6
(d) 8
Answer
C
Question. For positive numbers x, y, z the numerical value of the determinant
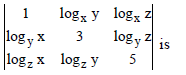
(a) 0
(b) log x log y log z
(c) 1
(d) 8
Answer
D
Question.
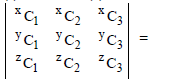
(a) xyz (x – y) (y – z) (z – x)
(b) xyz/6 (x – y)(y – z)(z – x)
(c) xyz/12 (x – y)(y – z)(z – x)
(d) None of these
Answer
C
Question.
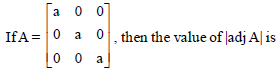
(a) a27
(b) a9
(c) a6
(d) a2
Answer
C
Question. If 1, ω, ω2 the cube roots of unity, then the value of
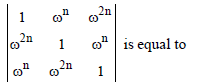
(a) 1
(b) ω
(c) ω2
(d) 0
Answer
D
Question. If the area of a triangle ABC, with vertices A(1, 3), B(0, 0) and C(k, 0) is 3 sq. units, then the value of k is
(a) 2
(b) 3
(c) 4
(d) 5
Answer
A
Question.
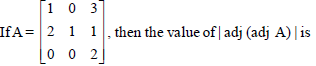
(a) 14
(b) 16
(c) 15
(d) 12
Answer
B
Question.
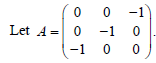
A The only correct statement about the matrix A is
(a) A2 = I
(b) A = (–1) I, where I is a unit matrix
(c) A–1 does not exist
(d) A is a zero matrix
Answer
A
Question.
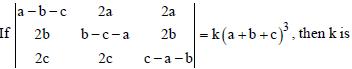
(a) 0
(b) 1
(c) 2
(d) 3
Answer
B
Question.
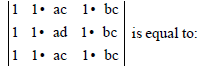
(a) a + b + c
(b) 1
(c) 0
(d) 3
Answer
C
Question. The value of the determinant
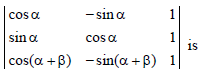
(a) independent of α
(b) independent of β
(c) independent of α and β
(d) None of the above
Answer
A
Question.
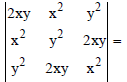
(a) (x3 + y3)2
(b) (x2 + y2)3
(c) – (x2 + y2)3
(d) –(x3 + y3)2
Answer
D
Question. The system of linear equations : x + y + z = 0, 2x + y – z = 0, 3x + 2y = 0 has :
(a) no solution
(b) a unique solution
(c) an infinitely many solution
(d) None of these
Answer
C
Question. If x, y ∈ R, then the determinant
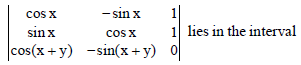
(a) [– √2, √2]
(b) [–1, 1]
(c) [– √2, 1]
(d) [–1,– √2]
Answer
A
Question.
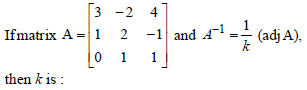
(a) 7
(b) – 7
(c) 15
(d) – 11
Answer
C
ASSERTION – REASON TYPE QUESTIONS
(a) Assertion is correct, reason is correct; reason is a correct explanation for assertion.
(b) Assertion is correct, reason is correct; reason is not a correct explanation for assertion
(c) Assertion is correct, reason is incorrect
(d) Assertion is incorrect, reason is correct.
Question. Assertion: The points A (a, b + c), B (b, c + a) and C (c, a + b) are collinear.
Reason: Area of a triangle with three collinear points is zero.
Answer
A
Question.
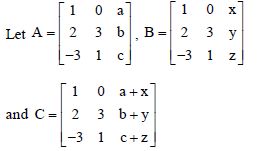
Assertion: det A + det B = det C.
Reason: A + B = C.
Answer
C
Question. Let A be a 2 × 2 matrix
Assertion: adj (adj A) = A
Reason: |adj A |= |A|
Answer
B
Question. Assertion: The value of determinant of a matrix and the value of determinant of its transpose are equal.
Reason: The value of determinant remains unchanged if its rows and columns are interchanged.
Answer
A
Question.
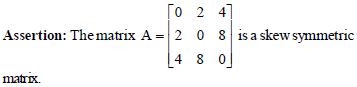
Reason: For the given matrix A’ = A.
Answer
D
Question. Consider the system of equations
x – 2y + 3z = –1
–x + y – 2z = k
x – 3y + 4z = 1
Assertion: The system of equations has no solution for k ≠ 3.
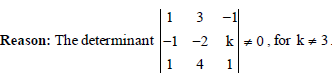
Answer
A
Case Based Questions
A company produces three products every day. Their production on certain day is 45 tons. It is found that the production of third product exceeds the production of first product by 8 tons while the total production of first and third product is twice the production of second product.
Using the concepts of matrices and determinants, answer the following questions:
Question. If x, y and z respectively denotes the quantity (in tons) of first, second and third product produced, then which of the following is true?
(a) x + y + z = 45
(b) x + 8 = z
(c) x – 2y + z = 0
(d) All of these
Answer
D
Question. Which of the following is not true?
(a) |A| = |A′|
(b) (A′)–1 = (A–1)′
(c) A is skew-symmetric matrix of order 3 then |A| = 0
(d) |AB| = |A| + |B|
Answer
D
Question.
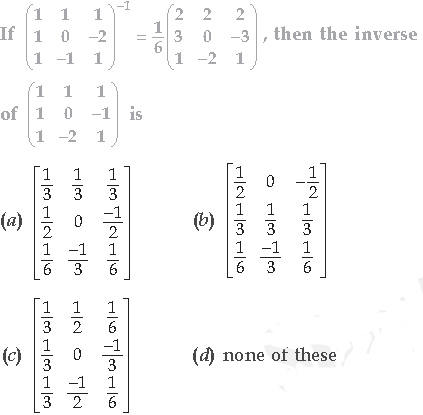
Answer
C
Question. x : y : z is equal to
(a) 12 : 13 : 20
(b) 11 : 15 : 19
(a) 15 : 19 : 11
(b) 13 : 12 : 20
Answer
B
Question. Which of the following is not true in the given determinant of A, where A = [aq]3×3?
(a) Order of minor is less than order of the determinant A.
(b) Minor of an element can never be equal to cofactor of the same element.
(c) Value of a determinant is obtained by multiplying elements of a row of column by corresponding cofactors.
(d) Order of minors and cofactors of same elements of A is same.
Answer
B
If there is a statement involving the natural number n such that (i) The statement is true for n = 1 (ii) When the statement is true for n = k (where k is some positive integer), then the statement is also true for n = k + 1. Then, the statement is true for all natural numbers n. Also, if A is a square matrix of order n, then A2 is defined as AA. In general, Am = AA…A (m times), where m is any positive integer
Based on the above information, answer the following questions:
Question.
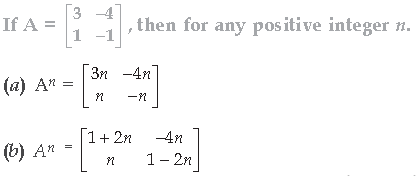
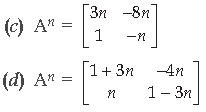
Answer
B
Question.
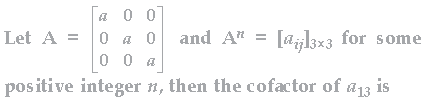
(a) an
(b) –an
(c) 2an
(d) 0
Answer
D
Question.
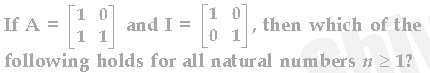
(a) An = nA – (n – 1)I
(b) An = 2n – 1 A – (n – 1)I
(c) An = nA + (n – 1)I
(d) An = 2n – 1 A + (n – 1)I
Answer
A
Question.
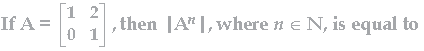
(a) 2n
(b) 3n
(c) n
(d) 1
Answer
D
Question. If A is a square matrix such that |A| = 2, then for any positive integer n, |An| is equal to
(a) 0
(b) 2n
(c) 2n
(d) n2
Answer
C
Each triangular face of the Pyramid of Peace in Kazakhstan is made up of 25 smaller equilateral triangles as shown in the figure.
Using the above information and concept of determinants, answer the following questions

Question. If the vertices of one of the smaller equilateral triangle are (0, 0), (3, √3) and (3,− √3), then the area of such triangle is
(a) √3 sq. units
(b) 2√3sq. units
(c) 3√3 sq. units
(d) none of these
Answer
C
Question. If (2, 4) and (2, 6) are two vertices of a smaller equilateral triangle, then the third vertex will lie on the line represented by
(a) x + y = 5
(b) x = 1 + √3
(c) x = 2 ± 3 √3
(d) 2x + y = 3
Answer
C
Question. The area of a face of the Pyramid is
(a) 25 √3 sq. units
(b) 50 √3 sq. units
(c) 75 √3 sq. units
(d) 35 √3 sq. units
Answer
C
Question. Let A(a, 0), B(0, b) and C(1, 1) be three points. If 1/a + 1/b, then the three points are
(a) vertices of an equilateral triangle
(b) vertices of a right angled triangle
(c) collinear
(d) vertices of an isosceles triangle
Answer
C
Question. The length of a altitude of a smaller equilateral triangle is
(a) 2 units
(b) 3 units
(c) √3 units
(d) 4 units
Answer
B
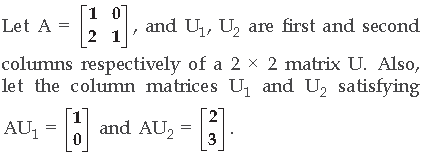
Based on the above information, answer the following questions:
Question. The value of |U| is
(a) 1
(b) –3
(c) 3
(d) –3
Answer
C
Question. The matrix U1 + U2 is equal to

Answer
C
Question. If X = [3 2]U[3/2], then the value of X =
(a) 3
(b) –3
(c) –5
(d) 5
Answer
D
Question. If U = [aij]2×2, then the value of a11A11 + a12A12, where Aij denotes the cofactor of aij is
(a) 1
(b) 2
(c) –3
(d) 3
Answer
D
Question. The minor of element at the position a22 in U is
(a) 1
(b) 2
(c) –2
(d) –1
Answer
A
The upward speed v(t) of a rocket at time t is approximated by v(t) = at2 + bt + c, 0 ≤ t ≤ 100, where a, b and c are constants. It has been found that the speed at times t = 3, t = 6 and t = 9 seconds are respectively 64, 133 and 208 miles per second.

Question. The value of a + c is
(a) 1
(b) 20
(c) 4/3
(d) none of these
Answer
C
Question. The value of b + c is
(a) 20
(b) 21
(c) 3/4
(d) 4/3
Answer
B
Question. v(t) is given by
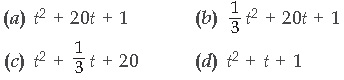
Answer
B
Question. The time at which the speed of rocket is 784 miles/sec. is
(a) 20 seconds
(b) 30 seconds
(c) 25 seconds
(d) 27 seconds
Answer
D
Question. The speed at time t = 15 seconds is
(a) 346 miles/sec.
(b) 356 miles/sec
(c) 366 miles/sec
(d) 376 miles/sec
Answer
D
