Please refer to Class 12 Mathematics Sample Paper With Solutions Set C provided below. The Sample Papers for Class 12 Mathematics have been prepared based on the latest pattern issued by CBSE. Students should practice these guess papers for class 12 Mathematics to gain more practice and get better marks in examinations. The Sample Papers for Mathematics Standard 12 will help you to understand the type of questions which can be asked in upcoming examinations.
PART – A
Section – I
1. If a matrix A is both symmetric and skew-symmetric, then show that A is a zero matrix.
Answer : Given, A is a symmetric matrix.
∴ AT = A …(i)
A is also a skew-symmetric matrix.
∴ AT = –A …(ii)
From (i) and (ii), A = –A ⇒ A = O
Hence, A is a zero matrix.
OR
If
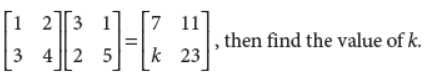
Answer :
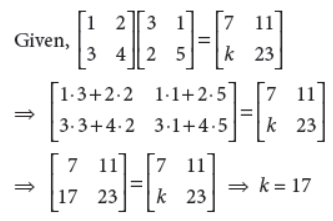
2. Evaluate :

Answer :

3. If the direction ratios of a line are 1, –3, 2, then find its direction cosines.
Answer :

OR
The coordinates of a point P are (3, 12, 4) w.r.t. origin O, then find the direction cosines of OP.
Answer : Direction ratios of OP are (3 – 0, 12 – 0, 4 – 0) or (3, 12, 4).
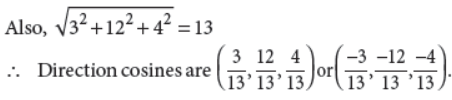
4. Let R be a relation defined on the set of natural numbers N as follow :
R = {(x, y) | x ∈ N, y ∈ N and 2x + y = 24}
Find the domain and range of the relation R.
Answer : Here, R = {(x, y) | x ∈ N, y ∈ N and 2x + y = 24}
∴ R = {(1, 22), (2, 20), (3, 18), (4, 16), (5, 14), (6, 12),
(7, 10), (8, 8), (9, 6), (10, 4), (11, 2)}
Domain of R = {1, 2, 3, 4, …, 11}
Range of R = {2, 4, 6, 8, 10, 12 ,…, 22}
5. Find the distance from the origin to the plane x + 3y – 2z + 1 = 0.
Answer :

OR
Find the foot of the perpendicular from (0, 0, 0) to 3x + 4y – 6z = 0.
Answer : Given plane is 3x + 4y – 6z = 0 …(i)
The d.r.’s of normal to plane (i) are 3, 4, –6.

6. Construct a matrix A = [aij]2×2, where aij = i + j.
Answer : Here, a11 = 1 + 1 = 2, a12 = 1 + 2 = 3,
a21 = 2 + 1 = 3 and a22 = 2 + 2 = 4

7. If p̅ = î + 2ĵ + 3k̂, q̅ = î + 4ĵ – 3k̂ are the position vectors of points P, Q respectively and point R (r̅,) divides the line PQ internally in the ratio 2 : 1, then find the coordinates of R.
Answer :
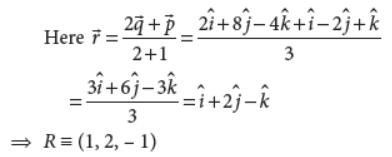
OR
a̅ = î + 3k̂, b̅ = 2î + 5ĵ , c̅ = 4î + 2ĵ and c̅ = t1a̅ + t2b̅ then find the value of t1 and t2.
Answer :
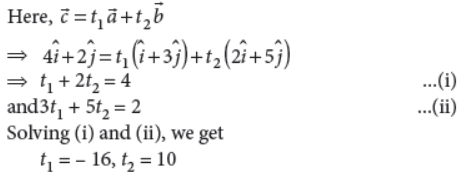
8. Find the principal value of tan-1 (√–3).
Answer :

9. Evaluate :

Answer :
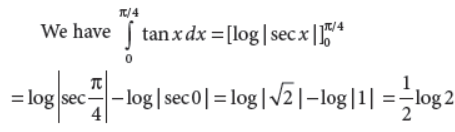
OR
Evaluate : ∫x cot-1xdx
Answer :

10. Find the value of î(ĵ x k̂) + ĵ( î x k̂) +k̂( î x ĵ)
Answer : î. î + ĵj(−ĵ)+ k̂.k̂ = 1−1+1= 1
11. If (2, 4, –3) is the foot of the perpendicular drawn from the origin to a plane, then find the equation of the plane.
Answer : Foot of perpendicular from (0, 0, 0) to the plane is (2, 4 –3), then
a̅ = 2î+ 4ĵ− 3k̂
Normal to plane is,
n̅ = 2î+ 4ĵ− 3k̂
∴ Equation of plane is,
r̅.n̅ = a̅.n̅
⇒ (xî + yĵ + zk̂).(2î + 4ĵ – 3k̂)
∴ Equation of plane is, r̅.n̅ = a̅.n̅
(xî + yĵ + zk̂).(2î + 4ĵ – 3k̂) = 4+16+9 = 29
⇒ 2x + 4y – 3z – 29 = 0
12. Find the domain of f(x) = sin–1 x + tan–1 x + sec–1x.
Answer : Given f(x) = sin–1x + tan–1x + sec–1x
Domain of sin–1x = [–1, 1]
Domain of tan–1x = (–∞, ∞)
Domain of sec–1x = (–∞, ∞) – (–1, 1)
Domain of f(x) = [–1, 1] ∩ (–∞, ∞) ∩ [(–∞, ∞) – (–1, 1)] = {–1, 1}
13. Find the equation of the line in symmetric form which passes through the points A(–2, –1, 5) and B(1, 3, –1).
Answer : The symmetric form of the equation of line passing through A(–2, –1, 5) and B(1, 3, –1) is
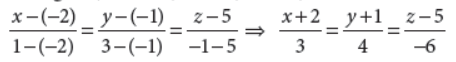
14. Find the value of x for which the matrix

Answer : A is singular ∴ |A| = 0

15. The cartesian equations of a line are 6x – 2 = 3y + 3 = 2z – 4. Find the direction ratios of the line.
Answer : The equations of the given line are
6x – 2 = 3y + 3 = 2z – 4
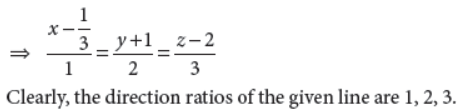
16. Find the integrating factor of the differential equation dy/dx + y/√1–x2 = x.
Answer :

Section – II
Case study-based questions are compulsory. Attempt any 4 sub parts from each question. Each sub-part carries 1 mark.
17. In a family there are four children. All of them have to work in fields to earn their livelihood at the age of 15.

Based on the above information, answer the following questions :
(i) Probability that all children working in fields are boys if it is given that elder child working in fields is a boy, is
(a) 3/8
(b) 1/8
(c) 5/8
(d) none of these
Answer : B
(ii) Probability that all children working in fields are grass, if first two children working in fields are girls, is
(a) 1/4
(b) 3/4
(c) 1/2
(d) none of these
Answer : A
(iii) Find the probability that two middle child working in fields are boys if it is given that first child working in fields is a girl.
(a) 0
(b) 3/4
(c) 1/4
(d) none of these
Answer : C
(iv) Find the probability that all children working in fields are girls if it is given that at least one of the children working in fields is a girl.
(a) 0
(b) 1/15
(c) 2/15
(d) 4/15
Answer : B
(v) Find the probability that all children working in fields are boys if it is given that at least three of the children working in fields are boys.
(a) 1/5
(b) 2/5
(c) 3/5
(d) 4/5
Answer : A
18. In a street two lamp posts are 300 feet apart. The light intensity at a distance d from the first (stronger) lamp post is 1000/d2 , the light intensity at distance d from the second (weaker) lamp post is 125/d2 (in both cases the light intensity is inversely proportional to the square of the distance to the light source).
The combined light intensity is the sum of the two light intensities coming from both lamp posts.
Based on the above information, answer the following.
(i) If you are in between the lamp posts, at distance x feet from the stronger light, then the formula for the combined light intensity coming from both lamp posts as function of x, is
(a) 1000/x2 + 125/x2
(b) 1000/(3000 –x2) + 125/x2
(c) 1000/x2 + 125/(3000 –x2)
(d) None of these
Answer : C
(ii) The maximum value of x can not be
(a) 100
(b) 200
(c) 300
(d) None of these
Answer : C
(iii) The minimum value of x can not be
(a) 0
(b) 100
(c) 200
(d) None of these
Answer : A
(iv) If I(x) denote the combined light intensity, then I(x) will be minimum when x =
(a) 100
(b) 200
(c) 300
(d) 150
Answer : B
(v) The darkest spot between the two lights is
(a) at a distance of 100 feet from the weaker lamp post.
(b) at distance of 100 feet from the stronger lamp post.
(c) at a distance of 200 feet from the weaker lamp post.
(d) None of these
Answer : A
PART – B
Section – III
19. Evaluate

Answer :
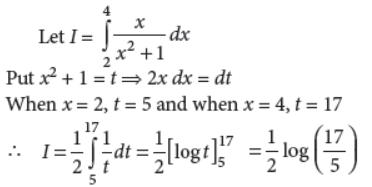
20. If y2 = ax2 + bx + c, then find the value of d/dx(y3y2)
Answer : Given, y2 = ax2 + bx + c
Differentiating both sides, we get 2yy1 = 2ax + b …(i)
Again differentiating, we get 2yy2 + y1(2y1) = 2a
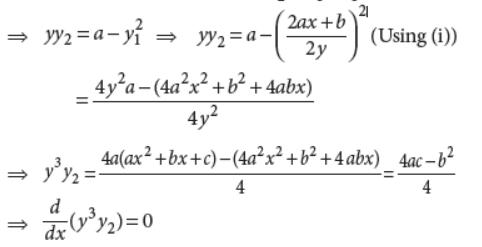
OR
Differentiate ex log(sin 2x) w.r.t. x.
Answer :


21. A bag contains 6 red, 5 blue and 7 white balls. If three balls are drawn one by one (without replacement), then what is the probability that all three balls are blue?
Answer : Let A, B and C be the events of drawing a blue ball in first, second and third draw respectively.
Then probability of getting blue ball in all three draws
= P(A ∩ B ∩ C) = P(A)⋅P(B|A)⋅P(C|A ∩ B)

22. Determine the area enclosed between the curve y = cos2 x, 0 ≤ x ≤ π/2 and the axes.
Answer :
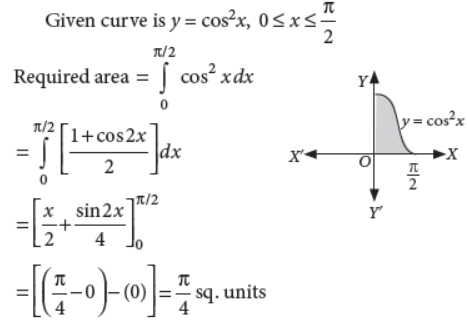
23. Find a unit vector perpendicular to the plane of a̅ = 2î – 6ĵ – 3k̂ and b̅ = 4î + 3ĵ – k̂.
Answer :

OR
Find the angle between the vectors a̅ + b̅ and a̅ −b̅ , where a̅ = î + ĵ + 4k̂ and b̅ = î – ĵ + 4k̂.
Answer :
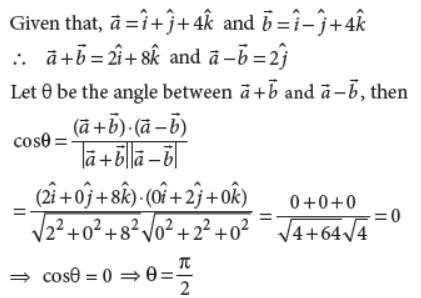
24. Solve the differential equation dy/dx + 1 = ex+y.
Answer :

25. An unbiased dice is thrown twice. Let the event A be ‘odd number on the first throw’ and B be the event ‘odd number on the second throw’. Check the independence of the events A and B.
Answer : If all the 36 elementary events of the experiment are considered to be equally likely, we have

26. Evaluate :
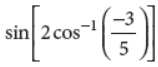
Answer :

27. If

Answer :

From (i) and (ii), we get R.H.S. ≠ L.H.S.
28. Find the values of x if f (x) = 6(x2 – 5x – 24) is an increasing function.
Answer :

OR
A rod 108 metres long is bent to form a rectangle. Find its dimensions, if its area is maximum.
Answer : Let x be the length and y be the breadth of the rectangle
∴ 2x + 2y = 108 ⇒ y = 54 – x
Now, area of rectangle = xy =x(54 – x)
Let f (x) = 54x – x2
∴ f ′(x) = 54 – 2x and f ′′(x) = – 2
For extreme values, f ′(x) = 0
⇒ 54 – 2x = 0 ⇒ x = 27
f ′′(27) = –2 < 0
∴ Area is maximum when x = 27, y = 27
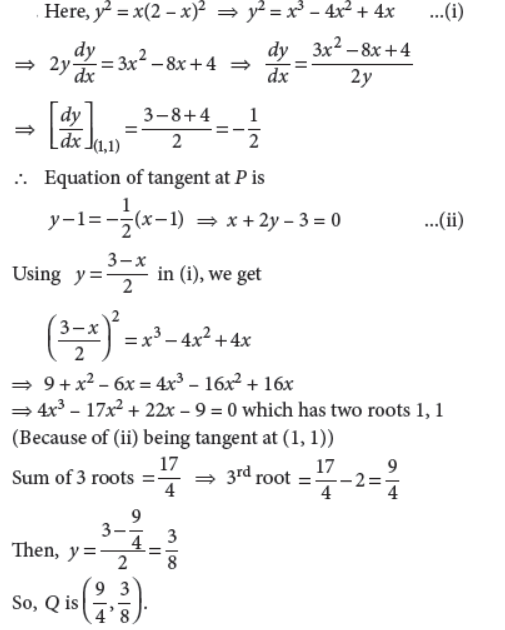
29. If the tangent at P(1, 1) on y2 = x(2 – x)2 meets the curve again at Q, then find the point Q.
Answer :
30. Show that the function f : R → R given by f(x) = x3 + x is bijective.
Answer : Here, f : R → R, f(x) = x3 + x
One-One : x1, x2 ∈ R such that, f(x1) = f(x2)
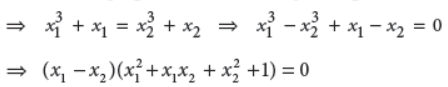

31. If xdy/dx + 2y = ln x, then find the value of e2 y(e) – y(1).
Answer :

OR
Solve the initial value problem 2xy + y2– 2x2 dy/dx = 0 y (1) = 2.
Answer :
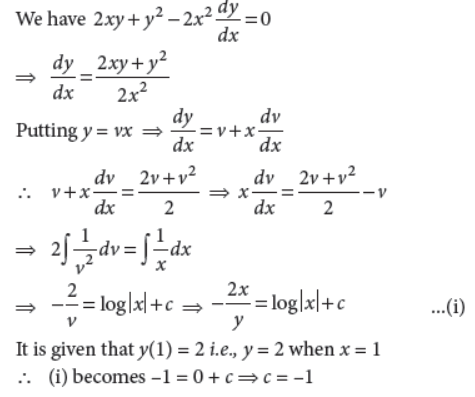

32. Find the value of k, for which

Answer : Since, f(x) is continuous at x = 0.

33. Find the area bounded by the circle x2 + y2 = 8x and the line x = 2.
Answer : Given curve is x2 + y2 = 8x


34. Evaluate
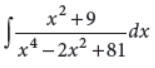
Answer :
OR
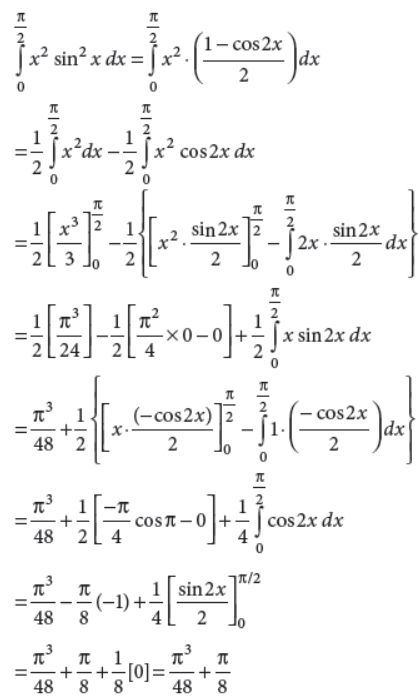
Evaluate :
35. Find the second order derivative of a sin3t with respect to a cos3t at t = π/ 4 .
Answer : Let y = asin3t and x = a cos3 t
On differentiating w.r.t. t, we get


Section – V
36. Solve the following problem graphically :
Maximize Z = 22x + 18y
Subject to constraints :
x + y ≤ 20
36x + 24y ≤ 576
x, y ≥ 0
Answer : To solve this LPP graphically, we first convert the inequations into equations l1 : x + y = 20,
l2 : 36x + 24y = 576, l3 : x = 0, l4 : y = 0. Draw the corresponding lines.
The feasible region of the LPP is shaded in figure.
The corner points of the feasible region OA2PB1 are
O(0, 0), A2(16, 0), P(8, 12) and B1(0, 20).

The values of the objective function Z at corner-points of the feasible region are given in the following table.
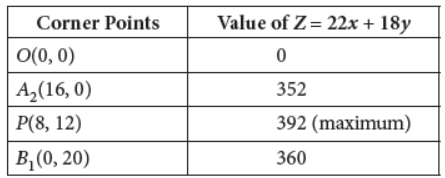
∴ Z has maximum value 392 at P(8, 12).
OR
Find the maximum value of z = 3x + 5y subject to x + 4y ≤ 24, 3x + y ≤ 21, x + y ≤ 9, x ≥ 0, y ≥ 0.
Answer : Converting inequations into equations and drawing the corresponding lines.
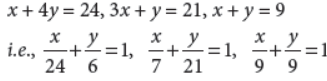
As x ≥ 0, y ≥ 0 solution lies in first quadrant

The feasible region of the LPP is shaded in figure. The corner points of the feasible region OABCD are
O(0, 0), A(7, 0), B(6, 3), C(4, 5) and D(0, 6).
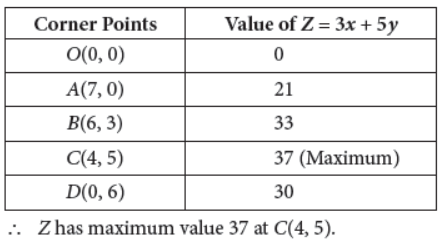
37. Find the equation of the plane containing the lines r̅ = î + ĵ + λ( î + ĵ – k̂) and r̅ = î + ĵ + μ(– î + ĵ – 2k̂)
Find the distance of this plane from origin and also from the point (1, 1, 1).
Answer :

This is the equation of the required plane.
We know that the distance of a point P with position

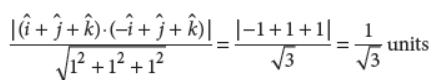
OR
Find the length of the perpendicular drawn from the point (2, 4, –1) to the line r̅ = î + ĵ + λ( 2î + ĵ + 2k̂)
Answer : Let M be the foot of the perpendicular drawn from

38. If

then find A–1 and hence solve the system of linear equations x + 2y + z = 4, –x + y + z = 0, x – 3y + z = 2.
Answer :
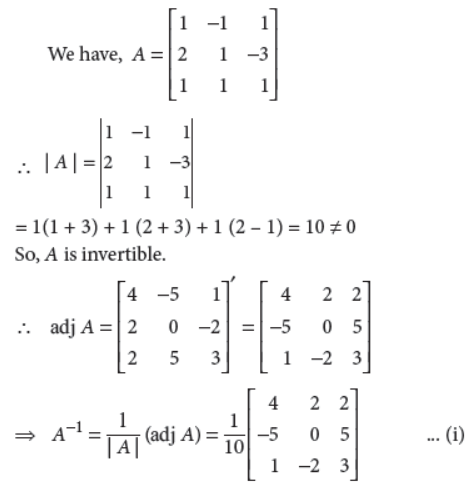
Now, the given system of equations is expressible as
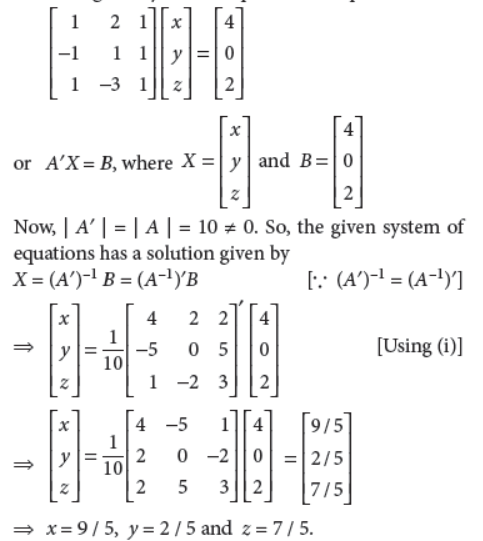
OR
Solve the following system of equations :
3x – y + z = 5
2x – 2y + 3z = 7
x + y – z = –1
Answer : The given system of equations can be written as AX = B

Hence, the solution of the given system of equations is x = 1, y = –1 and z = 1.
